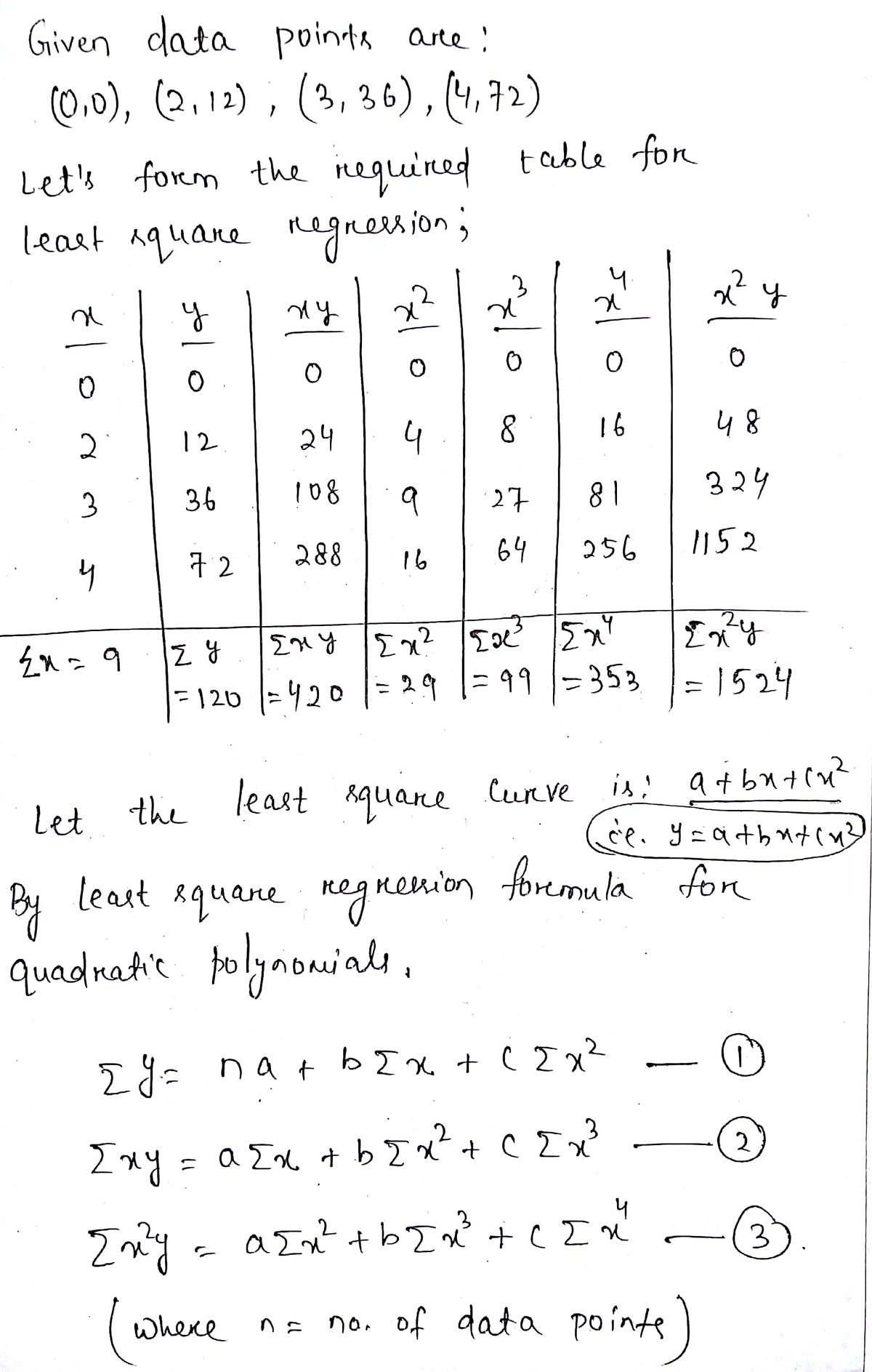
Find the least squares regression quadratic polynomial for the data points. (Let x be the independent variable and y be the dependent variable.)(0, 0), (2, 12), (3, 36), (4, 72)

Answered: Image /qna-images/answer/b85627df-4236-43c1-8a7d-d98999d072a8.jpg

Answered: Find the least squares regression…

Advanced Engineering Mathematics

10th Edition

ISBN:9780470458365

Author:Erwin Kreyszig

Publisher:Erwin Kreyszig

Chapter2: Second-order Linear Odes

Section: Chapter Questions

Problem 1RQ

See similar textbooks

Similar questions

Fit a linear function of the form f (t) = c0 + c1t to the data points (0, 0), (0, 1), (1, 1), using least squares. Use only paper and pencil. Sketch your solution, and explain why it makes sense.
We use the form ŷ = a + bx for the least-squares line. In some computer printouts, the least-squares equation is not given directly. Instead, the value of the constant a is given, and the coefficient b of the explanatory or predictor variable is displayed. Sometimes a is referred to as the constant, and sometimes as the intercept. Data from a report showed the following relationship between elevation (in thousands of feet) and average number of frost-free days per year in a state. A Minitab printout provides the following information. Predictor Coef SE Coef T. Constant 317.97 28.31 11.24 0.002 Elevation -28.572 3.511 -8.79 0.003 S = 11.8603 R-Sq 94.2% %3D Notice that "Elevation" is listed under "Predictor." This means that elevation is the explanatory variable x. Its coefficient is the slope b. "Constant" refers to a in the equation ŷ = a + bx. (a) Use the printout to write the least-squares equation. %3D (b) For each 1000-foot increase in elevation, how many fewer frost-free days are…
Use the following linear regression equation to answer the questions. X1 = 1.5 + 3.6x2 - 7.7x3 + 2.3x4 (a) Which variable is the response variable? O X4 O X2 O 3 Which variables are the explanatory variables? (Select all that apply.) O 3 O X4 O X1 O ×2 (b) Which number is the constant term? List the coefficients with their corresponding explanatory variables. constant X2 coefficient X, coefficient X4 coefficient (c) If x2 = 4, x3 = 9, and x4 = 10, what is the predicted value for x,? (Use 1 decimal place.) (d) Explain how each coefficient can be thought of as a "slope" under certain conditions. O If we look at all coefficients together, each one can be thought of as a "slope." O If we hold all explanatory variables as fixed constants, the intercept can be thought of as a "slope." O If we hold all other explanatory variables as fixed constants, then we can look at one coefficient as a "slope." O If we look at all coefficients together, the sum of them can be thought of as the overall…
We use the form ŷ = a + bx for the least-squares line. In some computer printouts, the least-squares equation is not given directly. Instead, the value of the constant a is given, and the coefficient b of the explanatory or predictor variable is displayed. Sometimes a is referred to as the constant, and sometimes as the intercept. Data from a report showed the following relationship between elevation (in thousands of feet) and average number of frost-free days per year in a state. A Minitab printout provides the following information. Predictor Сoef SE Coef T Constant 315.81 28.31 11.24 0.002 Elevation -31.650 3.511 -8.79 0.003 S = 11.8603 R-Sq = 94.6% Notice that "Elevation" is listed under "Predictor." This means that elevation is the explanatory variable x. Its coefficient is the slope b. "Constant" refers to a in the equation ŷ = a + bx. (a) Use the printout to write the least-squares equation. ŷ : + %| (b) For each 1000-foot increase in elevation, how many fewer frost-free days…
The following data is given: x -2 -1 0 1 2 y 1.5 3.2 4.5 3.4 2 Determine the coefficients a and b in the function y=a/(x2+b) that best fit the data. (Write the function in a linear form, and use linear least-squares regression to determine the value of the coefficients.) Once the coefficients are determined make a plot that shows the function and the data points.
A horizontal least squares regression line, implies that: Select one: a. The slope of the regression equation is 0 * b. None of the above is correct O c. The slope of the regression equation is positive d. The slope of the regression equation is negative
determine whether the statement is true or false. If the statement is false, rewrite it as a true statement. The y-intercept b0 of a least-squares regression line has a useful interpretation only if the x-values are either all positive or all negative.
It is thought that basketball teams that make too many fouls in a game tend to lose the game even if they otherwise play well. Let x be the number of fouls more than (i.e., over and above) the opposing team. Let y be the percentage of times the team with the larger number of fouls wins the game. x 1 2 5 6 y 48 41 33 26 Find the equation of the least-squares line = a + bx. (Round your answers to four decimal places.) = + x
а. Complete the table. (or just write answers) Xi Yi 2 2 3 4 Totals E x; = E yi = Exf = Σχy Find SSxy, SSXX B1. x, y, and fo- Write the equation of the least squares line. b. C. d) What will be y if x=10
Use the following linear regression equation to answer the questions. x1 = 2.0 + 3.6x2 – 7.8x3 + 2.1x4 a) Which variables are the explanatory variables? (Select all that apply.) x3 x1 x2 x4 (b) Which number is the constant term? List the coefficients with their corresponding explanatory variables. constant _____ x2 coefficient _____ x3 coefficient _____ x4 coefficient ______ (c) If x2 = 9, x3 = 3, and x4 = 6, what is the predicted value for x1? (Use 1 decimal place.)
Use the following linear regression equation to answer the questions. X1 = 1.2 + 3.6x2 - 7.6x3 + 2.5x4 (a) Which variable is the response variable? O x1 O X4 O x2 O x3 Which variables are the explanatory variables? (Select all that apply.) O x1 O X4 O X3 (b) Which number is the constant term? List the coefficients with their corresponding explanatory variables. constant X2 coefficient X3 coefficient X4 coefficient (c) If x2 = 8, X3 = 6, and x4 = 4, what is the predicted value for x1? (Use 1 decimal place.) (d) Explain how each coefficient can be thought of as a "slope" under certain conditions. O If we look at all coefficients together, each one can be thought of as a "slope." If we hold all explanatory variables as fixed constants, the intercept can be thought of as a "slope." O If we look at all coefficients together, the sum of them can be thought of as the overall "slope" of the regression line. O If we hold all other explanatory variables as fixed constants, then we can look at…

Recommended textbooks for you

Advanced Engineering Mathematics

Advanced Math

ISBN:

9780470458365

Author:

Erwin Kreyszig

Publisher:

Wiley, John & Sons, Incorporated

Numerical Methods for Engineers

Advanced Math

ISBN:

9780073397924

Author:

Steven C. Chapra Dr., Raymond P. Canale

Publisher:

McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…

Advanced Math

ISBN:

9781118141809

Author:

Nathan Klingbeil

Publisher:

WILEY

Advanced Engineering Mathematics

Advanced Math

ISBN:

9780470458365

Author:

Erwin Kreyszig

Publisher:

Wiley, John & Sons, Incorporated

Numerical Methods for Engineers

Advanced Math

ISBN:

9780073397924

Author:

Steven C. Chapra Dr., Raymond P. Canale

Publisher:

McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…

Advanced Math

ISBN:

9781118141809

Author:

Nathan Klingbeil

Publisher:

WILEY

Mathematics For Machine Technology

Advanced Math

ISBN:

9781337798310

Author:

Peterson, John.

Publisher:

Cengage Learning,

Basic Technical Mathematics

Advanced Math

ISBN:

9780134437705

Author:

Washington

Publisher:

PEARSON

Topology

Advanced Math

ISBN:

9780134689517

Author:

Munkres, James R.

Publisher:

Pearson,

SEE MORE TEXTBOOKS

Find the least squares regression quadratic polynomial for the data points. (Let x be the independent variable and y be the dependent variable.) (0, 0), (2, 12), (3, 36), (4, 72)