Find Position, Velocity and Acceleration Vectors Find the velocity vector for the position vector r(t) = (sin(12t), 5t7, e−5t). x component = y component z component Submit Question
Find Position, Velocity and Acceleration Vectors Find the velocity vector for the position vector r(t) = (sin(12t), 5t7, e−5t). x component = y component z component Submit Question
Advanced Engineering Mathematics
10th Edition
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Erwin Kreyszig
Chapter2: Second-order Linear Odes
Section: Chapter Questions
Problem 1RQ
Related questions
Question

Transcribed Image Text:### Find Position, Velocity, and Acceleration Vectors
**Problem Statement:**
Find the velocity vector for the position vector \(\vec{r}(t) = \langle \sin(12t), 5t^7, e^{-5t} \rangle\).
**Components to Determine:**
- **x component:**
- **y component:**
- **z component:**
Buttons:
- **Submit Question**
**Instructions:**
To determine the velocity vector, differentiate each component of the position vector \(\vec{r}(t)\) with respect to time \(t\).
**Hints for Students:**
- The x component is the derivative of \(\sin(12t)\).
- The y component is the derivative of \(5t^7\).
- The z component is the derivative of \(e^{-5t}\).
Fill in the blanks with the correct derivatives to find the velocity vector, and submit your answer by clicking the "Submit Question" button.
Expert Solution
Step 1: Formulae used
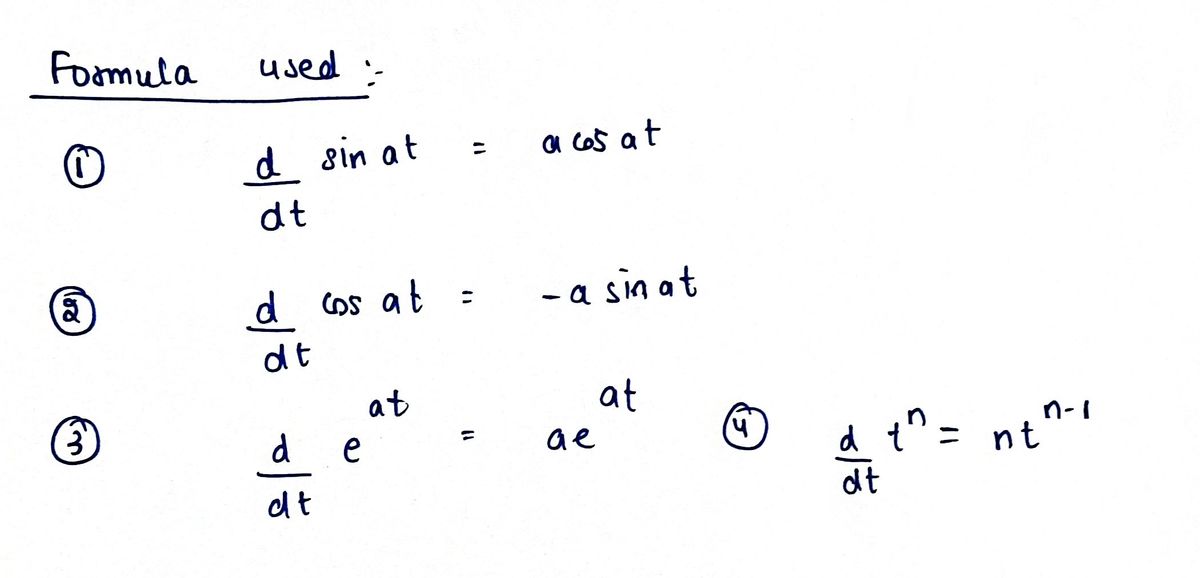
Step by step
Solved in 3 steps with 3 images

Recommended textbooks for you

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Mathematics For Machine Technology
Advanced Math
ISBN:
9781337798310
Author:
Peterson, John.
Publisher:
Cengage Learning,

