Algebra and Trigonometry (6th Edition)
6th Edition
ISBN:9780134463216
Author:Robert F. Blitzer
Publisher:Robert F. Blitzer
ChapterP: Prerequisites: Fundamental Concepts Of Algebra
Section: Chapter Questions
Problem 1MCCP: In Exercises 1-25, simplify the given expression or perform the indicated operation (and simplify,...
Related questions
Question
Please write clearly and legible
![**Ellipse Equation Problem**
*Find an equation of the ellipse that has center \((1, -3)\), a minor axis of length 2, and a vertex at \((-7, -3)\).*
---
This is a typical problem where you'll need to find the equation of an ellipse given specific characteristics:
1. **Center**: The center of the ellipse is \((h, k) = (1, -3)\).
2. **Minor Axis**: Since the minor axis is 2 units long, the semi-minor axis \(b\) is half of that length, so \(b = 1\).
3. **Vertex**: A vertex at \((-7, -3)\) indicates the ellipse is horizontally oriented. The radius along the major axis is the distance from the center to this vertex. Therefore, \(a = |-7 - 1| = 8\).
The general form of the equation of an ellipse centered at \((h, k)\) with semi-major axis \(a\) and semi-minor axis \(b\) (horizontally oriented) is:
\[
\frac{(x-h)^2}{a^2} + \frac{(y-k)^2}{b^2} = 1
\]
Substituting the given values:
\[
\frac{(x-1)^2}{64} + \frac{(y+3)^2}{1} = 1
\]](/v2/_next/image?url=https%3A%2F%2Fcontent.bartleby.com%2Fqna-images%2Fquestion%2Fac3be618-b645-4bd8-855b-808ff3fc18d4%2F88ef0846-6c9d-4398-b4c4-016d85880253%2F5ch0hz_processed.jpeg&w=3840&q=75)
Transcribed Image Text:**Ellipse Equation Problem**
*Find an equation of the ellipse that has center \((1, -3)\), a minor axis of length 2, and a vertex at \((-7, -3)\).*
---
This is a typical problem where you'll need to find the equation of an ellipse given specific characteristics:
1. **Center**: The center of the ellipse is \((h, k) = (1, -3)\).
2. **Minor Axis**: Since the minor axis is 2 units long, the semi-minor axis \(b\) is half of that length, so \(b = 1\).
3. **Vertex**: A vertex at \((-7, -3)\) indicates the ellipse is horizontally oriented. The radius along the major axis is the distance from the center to this vertex. Therefore, \(a = |-7 - 1| = 8\).
The general form of the equation of an ellipse centered at \((h, k)\) with semi-major axis \(a\) and semi-minor axis \(b\) (horizontally oriented) is:
\[
\frac{(x-h)^2}{a^2} + \frac{(y-k)^2}{b^2} = 1
\]
Substituting the given values:
\[
\frac{(x-1)^2}{64} + \frac{(y+3)^2}{1} = 1
\]
Expert Solution
Step 1
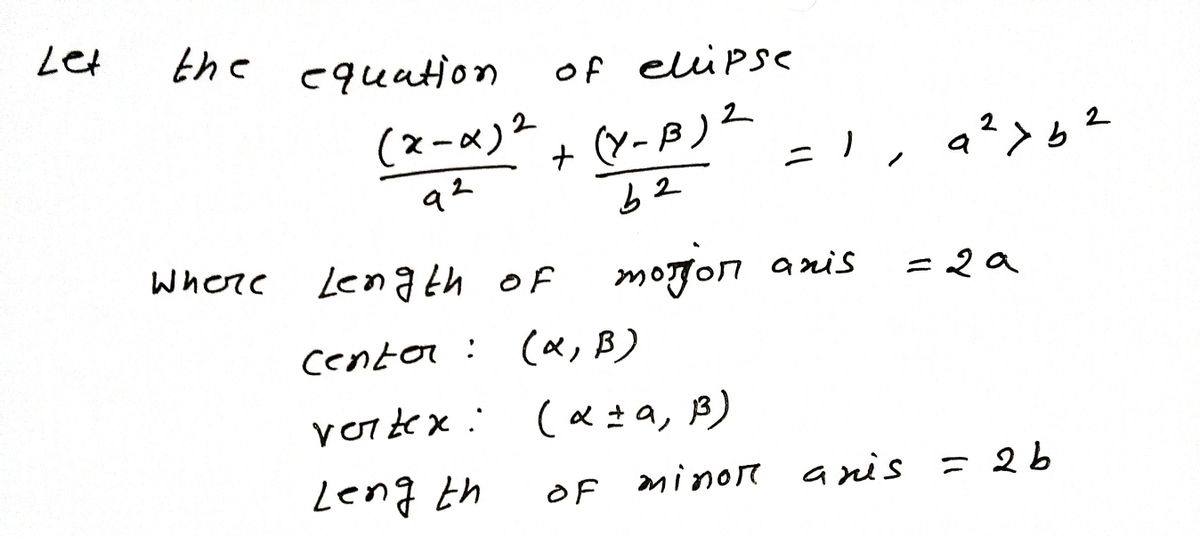
Step by step
Solved in 2 steps with 2 images

Recommended textbooks for you

Algebra and Trigonometry (6th Edition)
Algebra
ISBN:
9780134463216
Author:
Robert F. Blitzer
Publisher:
PEARSON

Contemporary Abstract Algebra
Algebra
ISBN:
9781305657960
Author:
Joseph Gallian
Publisher:
Cengage Learning

Linear Algebra: A Modern Introduction
Algebra
ISBN:
9781285463247
Author:
David Poole
Publisher:
Cengage Learning

Algebra and Trigonometry (6th Edition)
Algebra
ISBN:
9780134463216
Author:
Robert F. Blitzer
Publisher:
PEARSON

Contemporary Abstract Algebra
Algebra
ISBN:
9781305657960
Author:
Joseph Gallian
Publisher:
Cengage Learning

Linear Algebra: A Modern Introduction
Algebra
ISBN:
9781285463247
Author:
David Poole
Publisher:
Cengage Learning

Algebra And Trigonometry (11th Edition)
Algebra
ISBN:
9780135163078
Author:
Michael Sullivan
Publisher:
PEARSON

Introduction to Linear Algebra, Fifth Edition
Algebra
ISBN:
9780980232776
Author:
Gilbert Strang
Publisher:
Wellesley-Cambridge Press

College Algebra (Collegiate Math)
Algebra
ISBN:
9780077836344
Author:
Julie Miller, Donna Gerken
Publisher:
McGraw-Hill Education