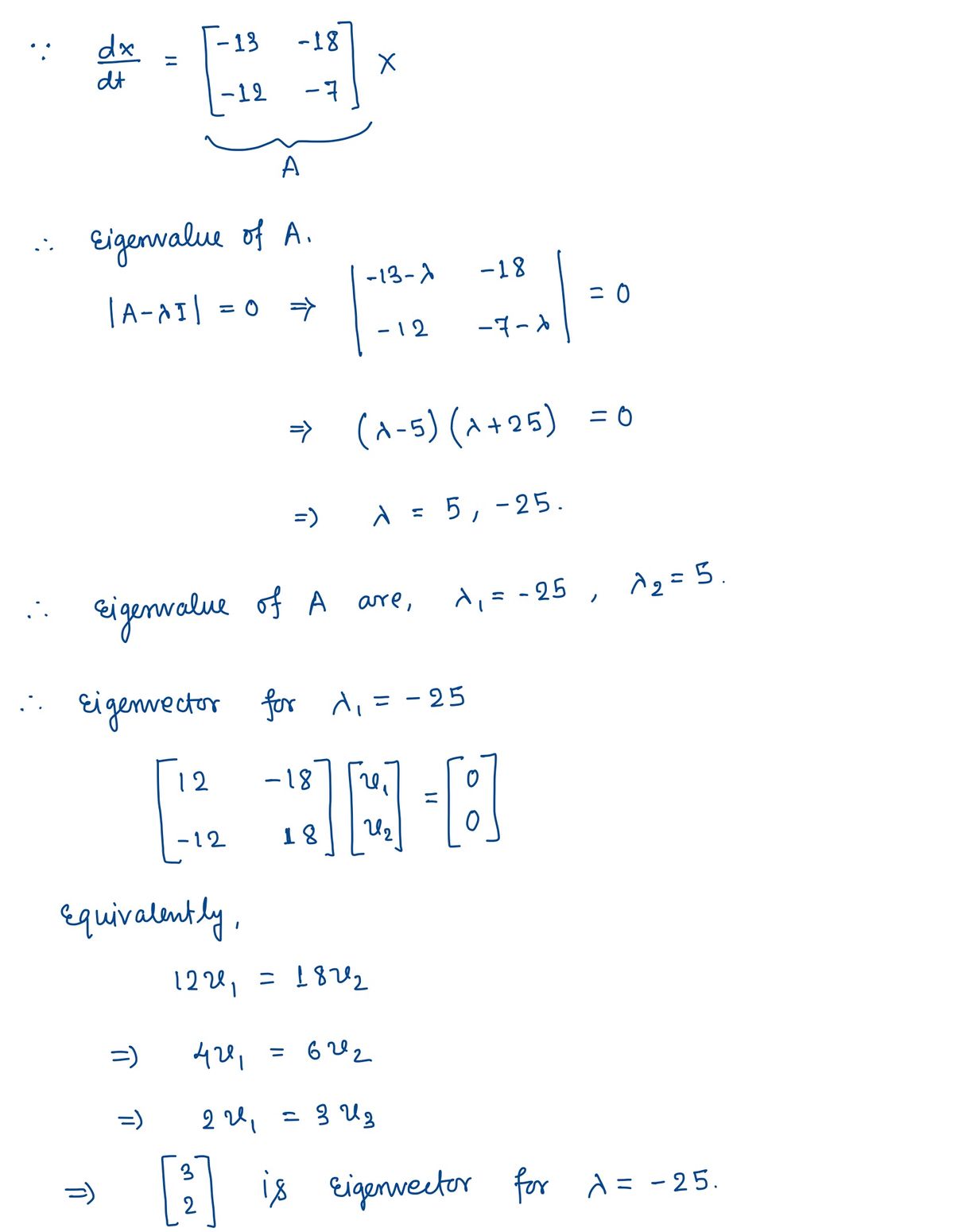
Find a general solution formula for the following linear system using the eigenvector method. dx -13 -18 dt 12 -7 Use cl, c2 for the arbitrary constants. You can use the vector field plot above to check the sanity of your answer: Are the arrows ingoing/outgoing consistently with your eigenvalues? Do the eigenvectors look right?
Find a general solution formula for the following linear system using the eigenvector method. dx -13 -18 dt 12 -7 Use cl, c2 for the arbitrary constants. You can use the vector field plot above to check the sanity of your answer: Are the arrows ingoing/outgoing consistently with your eigenvalues? Do the eigenvectors look right?
Advanced Engineering Mathematics
10th Edition
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Erwin Kreyszig
Chapter2: Second-order Linear Odes
Section: Chapter Questions
Problem 1RQ
Related questions
Question
![**Image Transcription for Educational Website**
---
**Find a general solution formula for the following linear system using the eigenvector method.**
\[
\frac{dx}{dt} = \begin{bmatrix} -13 & -18 \\ -12 & -7 \end{bmatrix} x
\]
**Use c1, c2 for the arbitrary constants. You can use the vector field plot above to check the sanity of your answer: Are the arrows ingoing/outgoing consistently with your eigenvalues? Do the eigenvectors look right?**
---
**Diagram Explanation:**
The diagram above is a vector field plot. It shows the behavior of the differential equations system in the phase plane. The vectors represented by arrows indicate the direction and relative magnitude of the rate of change of the components of vector \( x \) at any given point in the plane.
- **Arrows:** The purple arrows in the plot illustrate the flow direction of the system. Depending on the vector field's properties and the eigenvalues calculated, these arrows should indicate convergence (ingoing) or divergence (outgoing) behavior.
- **Lines:** There are diagonal lines in blue and green. These lines represent potential eigenvectors, which are direction vectors that remain unchanged in direction under the linear transformation (though they may change in magnitude). These should align with the eigenvectors derived from the matrix once computed.
**Reflection:** Check the alignment of the arrows with the lines and assess whether the direction and behavior match the expected patterns based on eigenvalues and eigenvectors determined from the given matrix.](/v2/_next/image?url=https%3A%2F%2Fcontent.bartleby.com%2Fqna-images%2Fquestion%2Fb493ecdd-cbdc-400d-a05a-de2010eb2d52%2Fd044323c-e341-4e92-aa57-8f5af49937ae%2Fy9ov8wn_processed.png&w=3840&q=75)
Transcribed Image Text:**Image Transcription for Educational Website**
---
**Find a general solution formula for the following linear system using the eigenvector method.**
\[
\frac{dx}{dt} = \begin{bmatrix} -13 & -18 \\ -12 & -7 \end{bmatrix} x
\]
**Use c1, c2 for the arbitrary constants. You can use the vector field plot above to check the sanity of your answer: Are the arrows ingoing/outgoing consistently with your eigenvalues? Do the eigenvectors look right?**
---
**Diagram Explanation:**
The diagram above is a vector field plot. It shows the behavior of the differential equations system in the phase plane. The vectors represented by arrows indicate the direction and relative magnitude of the rate of change of the components of vector \( x \) at any given point in the plane.
- **Arrows:** The purple arrows in the plot illustrate the flow direction of the system. Depending on the vector field's properties and the eigenvalues calculated, these arrows should indicate convergence (ingoing) or divergence (outgoing) behavior.
- **Lines:** There are diagonal lines in blue and green. These lines represent potential eigenvectors, which are direction vectors that remain unchanged in direction under the linear transformation (though they may change in magnitude). These should align with the eigenvectors derived from the matrix once computed.
**Reflection:** Check the alignment of the arrows with the lines and assess whether the direction and behavior match the expected patterns based on eigenvalues and eigenvectors determined from the given matrix.
Expert Solution
Step 1
Step by step
Solved in 2 steps with 2 images

Recommended textbooks for you

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Mathematics For Machine Technology
Advanced Math
ISBN:
9781337798310
Author:
Peterson, John.
Publisher:
Cengage Learning,

