need help with the picture below Does the graph represent $ y $ as a function of $ x $?- A. Yes, this represents a function- B. No, this does not represent a functionIf the graph represents $ y $ as a function of $ x $, state the domain and choose the correct range of the function. If the graph does not represent $ y $ as a function of $ x $, leave the answer blanks for $ x $ and choose option (E) from the multiple choice.The domain is: [Textbox for input]**Note:** Write your answer in interval notation. If the answer includes more than one interval write the intervals separated by the union symbol, $ U $. If the answer is $ -\infty $, input $-infinity$; if the answer is $ \infty $, input $ infinity $.The range is:- A. $[-8, -3] \cup \{-1\}$- B. $[-8, -5)$ $\cup$ \{-1\}- C. $[-8, -3)$- D. $[-8, -1]$- E. 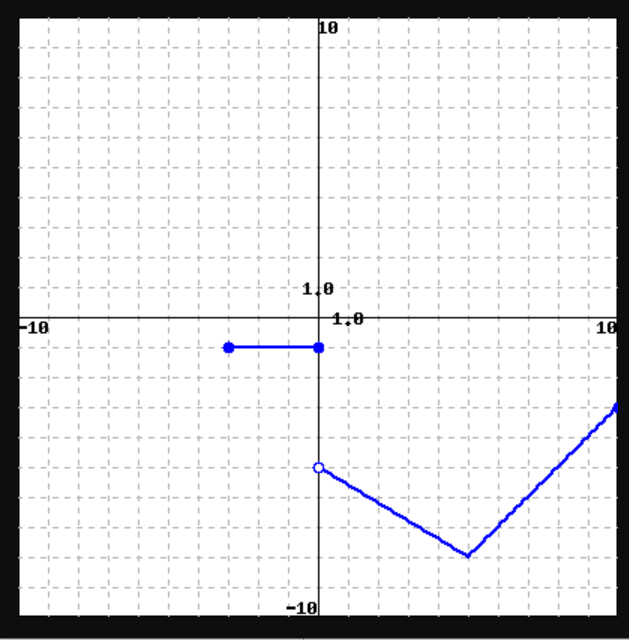Not a function The image displays a two-dimensional Cartesian coordinate plane with a piecewise linear graph. The x-axis and y-axis both range from -10 to 10, marked at intervals of 1 on a grid.### Graph Description:1. **Horizontal Segment:** - Positioned parallel to the x-axis at $ y = 1 $. - Begins approximately at $ x = 2 $ and ends at $ x = 4 $. - Both endpoints are marked with solid blue dots, indicating that the segment includes these points.2. **Angular Segment:** - Starts with an open blue dot at $(-2, -8)$, indicating that the segment does not include this point. - Moves upwards to a minimum point at the origin $(0, -10)$. - Then, it continues with a sharp turn, changing direction upwards to the right and ending at $(6, 3)$, marked with a solid blue dot.### Key Observations:- The line includes points of change where it starts and ends, and it exhibits two distinct paths: a horizontal section and an angular segment starting at negative values and moving upward.- The open and closed dots indicate whether the endpoints are included in the section of the graph they define.- This piecewise function graph can be used to illustrate concepts such as open and closed intervals, endpoints, and piecewise functions in mathematics.

Answered: Image /qna-images/answer/f762f74d-53e9-4094-be3d-ce830cbdf13b.jpg

Does the graph represent y as a function of a? OA. Yes, this represents a function OB. No, this does not represent a function If the graph represents y as a function of a, state the domain and choose the correct range of the function. If the graph does not represent y as a function of a, leave the answer blanks for and choose option (E) from the multiple choice. The domain is: Note: Write your answer in interval notation. If the answer includes more than one interval write the intervals separated by the union symbol, U. If the answer is-oo, input-infinity; if the answer is oo, input infinity. The range is: OA. [-8,-3] U {-1} OB. [-8,-5) U{-1} OC. [-8,-3] OD. [-8,-1] OE. Not a function 9.

Does the graph represent y as a function of a? OA. Yes, this represents a function OB. No, this does not represent a function If the graph represents y as a function of a, state the domain and choose the correct range of the function. If the graph does not represent y as a function of a, leave the answer blanks for and choose option (E) from the multiple choice. The domain is: Note: Write your answer in interval notation. If the answer includes more than one interval write the intervals separated by the union symbol, U. If the answer is-oo, input-infinity; if the answer is oo, input infinity. The range is: OA. [-8,-3] U {-1} OB. [-8,-5) U{-1} OC. [-8,-3] OD. [-8,-1] OE. Not a function 9.

Algebra and Trigonometry (6th Edition)

6th Edition

ISBN:9780134463216

Author:Robert F. Blitzer

Publisher:Robert F. Blitzer

ChapterP: Prerequisites: Fundamental Concepts Of Algebra

Section: Chapter Questions

Problem 1MCCP: In Exercises 1-25, simplify the given expression or perform the indicated operation (and simplify,...

See similar textbooks

Similar questions

Help with number 14
If you can show me what this image looks like translated down and reflected horizontally, it'd help a lot. Thank you! 4. Use patty papers to translate this image down and then reflect it horizontally. Submit the patty papers to your teacher. F I H G
The image below represents the framework for the roof of a house y R
NUMBER OF SQUARES ist 2nd 3rd Figure Number 1 2 3 4 8 10 PATTERN 1. Make a data table of the visual pattern. 2. Make a graph of the visual pattern. 3. Write an equation to represent the number of squares in the nth term of the visual pattern. Number of Squares FIGURE NUMBER
Please help.
A ***** bit ni bil p ph b Hertil a il tit °F Lostly sunny F1 1 Q A A clock with an hour hand that is 12 inches long is hanging on a wall. At noon, the vertical distance between the tip of the hour hand and the ceiling is 21 inches. At 3 P.M., the distance is 33 inches; at 6 P.M., 45 inches; at 9 P.M., 33 inches; and at midnight the distance is again 21 inches. If f(x) represents the distance between the tip of the hour hand and the ceiling x hours after noon, write the function that represents this situation in the form of f(x) = acos(bx) + d. Z f(x) = -12 cos(x) +33 f(x) = -33 cos (2x) +12 O F2 2 f(x) = -12 cos (2x) + 33 f(x) = -33 cos (x) + 12 @ W S X F3 # 3 E D F4 C 54 $ R F5 F V % 5 Q Search F6 T G A 6 B F7 4- Y H F8 & 7 + U N F9 J * 00 8 €1 M F10 Lo ( 9 K F11 G ) O L F12 O P Prt Sc { + 11 Insert [ 1 ? 1 Del Backspace | Enter 12:31 PM 6/19/2023 * ㄱ k Home Shift S