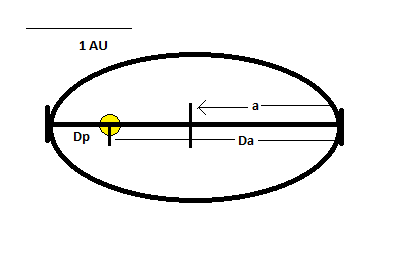
Determine the semimajor axis, a, of the ellipse shown below by following steps a) through d). Express all your answers in the appropriate number of significant figures. a) Measure the length of the scale bar on the screen in the units of your measuring device (cm-ruler or inch-ruler or ...). Convert the length of the scale bar into units of AU. This is going to be your scale factor to multiply all other measurements by. b) Measure D, with your measuring device then convert to AU using your scale factor from a). c) Measure D, with your measuring device then convert to AU using your scale factor from a). d) Determine the semimajor axis, a, by taking the average of D, and D...
Determine the semimajor axis, a, of the ellipse shown below by following steps a) through d). Express all your answers in the appropriate number of significant figures. a) Measure the length of the scale bar on the screen in the units of your measuring device (cm-ruler or inch-ruler or ...). Convert the length of the scale bar into units of AU. This is going to be your scale factor to multiply all other measurements by. b) Measure D, with your measuring device then convert to AU using your scale factor from a). c) Measure D, with your measuring device then convert to AU using your scale factor from a). d) Determine the semimajor axis, a, by taking the average of D, and D...
Advanced Engineering Mathematics
10th Edition
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Erwin Kreyszig
Chapter2: Second-order Linear Odes
Section: Chapter Questions
Problem 1RQ
Related questions
Question

Transcribed Image Text:a) Length of scale bar with units of your measuring device:
scale factor:
b) Dp in your units:
Dp in AU:
c) D, in your units:
D, in AU:

Transcribed Image Text:Determine the semimajor axis, a, of the ellipse shown below by following steps a)
through d). Express all your answers in the appropriate number of significant figures.
a) Measure the length of the scale bar on the screen in the units of your measuring
device (cm-ruler or inch-ruler or ...). Convert the length of the scale bar into units of
AU. This is going to be your scale factor to multiply all other measurements by.
b) Measure D, with your measuring device then convert to AU using your scale factor from a).
c) Measure D, with your measuring device then convert to AU using your scale factor from a).
d) Determine the semimajor axis, a, by taking the average of Dp and Da
E EM
V
graph
B I U A
Lato (Recom... V 19px... T
.
a) Length of scale bar with units of your measuring device:
a
(/)
DC
***
Expert Solution
Step 1
Since we know 1 AU = 14959807300000 cm
so,
(a) By measuring the line of the scale bar by a cm-ruler scale, it comes out to be 3.4 cm.
This means that 1 AU on the ground distance is represented in 3.4 cm in the image.
i.e., 3.4 cm = 1 AU = 14959807300000 cm
then 1 cm = 14959807300000 / 3.4 = 4.39 x 1012
so the scale of the image is 1 : 4.39 x 1012
Trending now
This is a popular solution!
Step by step
Solved in 2 steps with 1 images

Recommended textbooks for you

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Mathematics For Machine Technology
Advanced Math
ISBN:
9781337798310
Author:
Peterson, John.
Publisher:
Cengage Learning,

