Determine if the vectors are linearly independent. V1 Select the correct choice below and, if necessary, fill in the answer box to complete your choice. O A. The vectors are linearly independent because the vector equation x, v, + x2v2 = 0 has only the trivial solution. O B. The vectors are not linearly independent because if c, =|| and c2 = 1, both not zero, then c,V, +CzV2 = 0.
Determine if the vectors are linearly independent. V1 Select the correct choice below and, if necessary, fill in the answer box to complete your choice. O A. The vectors are linearly independent because the vector equation x, v, + x2v2 = 0 has only the trivial solution. O B. The vectors are not linearly independent because if c, =|| and c2 = 1, both not zero, then c,V, +CzV2 = 0.
Advanced Engineering Mathematics
10th Edition
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Erwin Kreyszig
Chapter2: Second-order Linear Odes
Section: Chapter Questions
Problem 1RQ
Related questions
Question
Two parts to this question
![**Determine if the vectors are linearly independent.**
\[
v_1 = \begin{bmatrix} 1 \\ -3 \end{bmatrix}, \quad v_2 = \begin{bmatrix} -2 \\ 6 \end{bmatrix}
\]
---
**Select the correct choice below and, if necessary, fill in the answer box to complete your choice.**
- ☐ A. The vectors are linearly independent because the vector equation \( x_1v_1 + x_2v_2 = 0 \) has only the trivial solution.
- ☑ B. The vectors are not linearly independent because if \( c_1 = \) [ ] and \( c_2 = 1 \), both not zero, then \( c_1v_1 + c_2v_2 = 0 \).](/v2/_next/image?url=https%3A%2F%2Fcontent.bartleby.com%2Fqna-images%2Fquestion%2F3d31c5b4-c6dc-4739-be04-6ff777f4b60f%2Fc107656e-94cf-4003-b731-c5a30be98469%2F7a0ea7_processed.png&w=3840&q=75)
Transcribed Image Text:**Determine if the vectors are linearly independent.**
\[
v_1 = \begin{bmatrix} 1 \\ -3 \end{bmatrix}, \quad v_2 = \begin{bmatrix} -2 \\ 6 \end{bmatrix}
\]
---
**Select the correct choice below and, if necessary, fill in the answer box to complete your choice.**
- ☐ A. The vectors are linearly independent because the vector equation \( x_1v_1 + x_2v_2 = 0 \) has only the trivial solution.
- ☑ B. The vectors are not linearly independent because if \( c_1 = \) [ ] and \( c_2 = 1 \), both not zero, then \( c_1v_1 + c_2v_2 = 0 \).
![**Determine if the columns of the matrix form a linearly independent set. Justify your answer.**
---
**Matrix:**
\[
\begin{bmatrix}
-2 & -1 & 0 \\
0 & -1 & 7 \\
1 & 1 & -14 \\
2 & 1 & -28
\end{bmatrix}
\]
**Instructions:**
Select the correct choice below and fill in the answer box within your choice. (Type an integer or simplified fraction for each matrix element.)
- **A.** If A is the given matrix, then the augmented matrix \(\Box\) represents the equation \(Ax = 0\). The reduced echelon form of this matrix indicates that \(Ax = 0\) has more than one solution. Therefore, the columns of A do not form a linearly independent set.
- **B.** If A is the given matrix, then the augmented matrix \(\Box\) represents the equation \(Ax = 0\). The reduced echelon form of this matrix indicates that \(Ax = 0\) has only the trivial solution. Therefore, the columns of A form a linearly independent set.
- **C.** If A is the given matrix, then the augmented matrix \(\Box\) represents the equation \(Ax = 0\). The reduced echelon form of this matrix indicates that \(Ax = 0\) has only the trivial solution. Therefore, the columns of A do not form a linearly independent set.
- **D.** If A is the given matrix, then the augmented matrix \(\Box\) represents the equation \(Ax = 0\). The reduced echelon form of this matrix indicates that \(Ax = 0\) has more than one solution. Therefore, the columns of A form a linearly independent set.](/v2/_next/image?url=https%3A%2F%2Fcontent.bartleby.com%2Fqna-images%2Fquestion%2F3d31c5b4-c6dc-4739-be04-6ff777f4b60f%2Fc107656e-94cf-4003-b731-c5a30be98469%2Fh0tjg0q_processed.png&w=3840&q=75)
Transcribed Image Text:**Determine if the columns of the matrix form a linearly independent set. Justify your answer.**
---
**Matrix:**
\[
\begin{bmatrix}
-2 & -1 & 0 \\
0 & -1 & 7 \\
1 & 1 & -14 \\
2 & 1 & -28
\end{bmatrix}
\]
**Instructions:**
Select the correct choice below and fill in the answer box within your choice. (Type an integer or simplified fraction for each matrix element.)
- **A.** If A is the given matrix, then the augmented matrix \(\Box\) represents the equation \(Ax = 0\). The reduced echelon form of this matrix indicates that \(Ax = 0\) has more than one solution. Therefore, the columns of A do not form a linearly independent set.
- **B.** If A is the given matrix, then the augmented matrix \(\Box\) represents the equation \(Ax = 0\). The reduced echelon form of this matrix indicates that \(Ax = 0\) has only the trivial solution. Therefore, the columns of A form a linearly independent set.
- **C.** If A is the given matrix, then the augmented matrix \(\Box\) represents the equation \(Ax = 0\). The reduced echelon form of this matrix indicates that \(Ax = 0\) has only the trivial solution. Therefore, the columns of A do not form a linearly independent set.
- **D.** If A is the given matrix, then the augmented matrix \(\Box\) represents the equation \(Ax = 0\). The reduced echelon form of this matrix indicates that \(Ax = 0\) has more than one solution. Therefore, the columns of A form a linearly independent set.
Expert Solution
Step 1
Since you have asked multiple question, we will solve the first question for you. If you want any specific question to be solved then please specify the question number or post only that question.
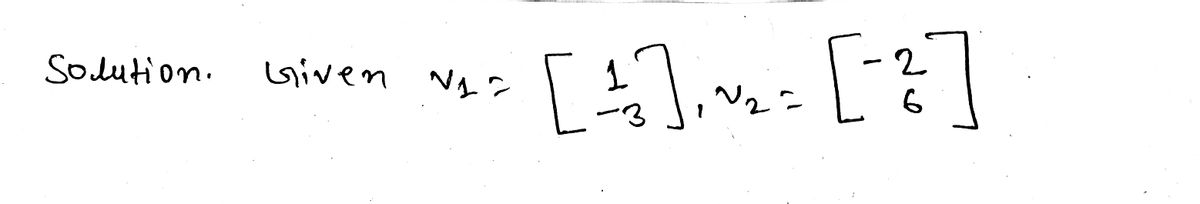
Step by step
Solved in 2 steps with 2 images

Recommended textbooks for you

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Mathematics For Machine Technology
Advanced Math
ISBN:
9781337798310
Author:
Peterson, John.
Publisher:
Cengage Learning,

