1. Consider a monopolistic firm operating in three different markets. Its revenue and cost functions: R = R₁ (Q₁) + R₂(Q2) + R3(Q3) C = C(Q) where Q = Q₁ + Q₂ + Q3- a. Define the profit maximization problem of the firm. b. Present the first-order condition (set of equations). c. Provide an economic interpretation to the first order condition. In particular, connect marginal revenues and demand elasticities to explain under what conditions the firm will charge a higher price. d. Present the Hessian of the firm's objective function. e. Analyze the second-order sufficient condition. f. Assume each of the revenue functions is concave and convex costs. Would this structure secure the second-order condition? Explain.
1. Consider a monopolistic firm operating in three different markets. Its revenue and cost functions: R = R₁ (Q₁) + R₂(Q2) + R3(Q3) C = C(Q) where Q = Q₁ + Q₂ + Q3- a. Define the profit maximization problem of the firm. b. Present the first-order condition (set of equations). c. Provide an economic interpretation to the first order condition. In particular, connect marginal revenues and demand elasticities to explain under what conditions the firm will charge a higher price. d. Present the Hessian of the firm's objective function. e. Analyze the second-order sufficient condition. f. Assume each of the revenue functions is concave and convex costs. Would this structure secure the second-order condition? Explain.
Chapter1: Making Economics Decisions
Section: Chapter Questions
Problem 1QTC
Related questions
Question
Please help solve this and explain into details. Thank you so much.
![### Monopolistic Firm Analysis in Three Different Markets
Consider a monopolistic firm operating in three distinct markets. The firm's revenue and cost functions are expressed as follows:
\[ R = R_1(Q_1) + R_2(Q_2) + R_3(Q_3) \]
\[ C = C(Q) \text{ where } Q = Q_1 + Q_2 + Q_3 \]
#### Questions and Instructions:
**1. Define the profit maximization problem of the firm.**
- Outline the optimization problem considering the revenues from each market and the total cost.
**2. Present the first-order condition (set of equations).**
- Derive and list the necessary conditions for profit maximization by setting up the firm's Lagrange function and finding its partial derivatives.
**3. Provide an economic interpretation to the first-order condition.**
- Explain the relationship between marginal revenues and demand elasticities. Discuss the conditions under which the firm will charge a higher price in each market, assuming different demand elasticities.
**4. Present the Hessian of the firm’s objective function.**
- Calculate and display the Hessian matrix, which includes the second partial derivatives of the profit function.
**5. Analyze the second-order sufficient condition.**
- Discuss the conditions under which the second-order derivatives ensure that the solution to the first-order conditions is a maximum.
**6. Assume each of the revenue functions is concave and convex costs. Would this structure secure the second-order condition? Explain.**
- Evaluate if the concavity of the revenue functions and convexity of the cost function fulfill the second-order sufficient conditions for profit maximization.
This exercise dives into advanced economic concepts that are crucial for understanding the pricing and output decisions of monopolistic firms across multiple markets. It emphasizes mathematical rigor in deriving optimal strategies under given economic conditions.](/v2/_next/image?url=https%3A%2F%2Fcontent.bartleby.com%2Fqna-images%2Fquestion%2F362a762f-914f-42d1-b0fa-542c2b1764ea%2F7103258e-2ef1-4bf9-8bc6-8e8ae5dc34bb%2Fgugivsr_processed.png&w=3840&q=75)
Transcribed Image Text:### Monopolistic Firm Analysis in Three Different Markets
Consider a monopolistic firm operating in three distinct markets. The firm's revenue and cost functions are expressed as follows:
\[ R = R_1(Q_1) + R_2(Q_2) + R_3(Q_3) \]
\[ C = C(Q) \text{ where } Q = Q_1 + Q_2 + Q_3 \]
#### Questions and Instructions:
**1. Define the profit maximization problem of the firm.**
- Outline the optimization problem considering the revenues from each market and the total cost.
**2. Present the first-order condition (set of equations).**
- Derive and list the necessary conditions for profit maximization by setting up the firm's Lagrange function and finding its partial derivatives.
**3. Provide an economic interpretation to the first-order condition.**
- Explain the relationship between marginal revenues and demand elasticities. Discuss the conditions under which the firm will charge a higher price in each market, assuming different demand elasticities.
**4. Present the Hessian of the firm’s objective function.**
- Calculate and display the Hessian matrix, which includes the second partial derivatives of the profit function.
**5. Analyze the second-order sufficient condition.**
- Discuss the conditions under which the second-order derivatives ensure that the solution to the first-order conditions is a maximum.
**6. Assume each of the revenue functions is concave and convex costs. Would this structure secure the second-order condition? Explain.**
- Evaluate if the concavity of the revenue functions and convexity of the cost function fulfill the second-order sufficient conditions for profit maximization.
This exercise dives into advanced economic concepts that are crucial for understanding the pricing and output decisions of monopolistic firms across multiple markets. It emphasizes mathematical rigor in deriving optimal strategies under given economic conditions.
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution!
Trending now
This is a popular solution!
Step by step
Solved in 4 steps

Follow-up Questions
Read through expert solutions to related follow-up questions below.
Follow-up Question
Continue from part d to part f. Thank you!
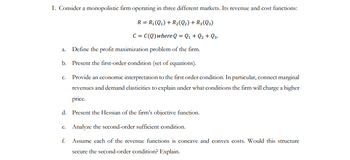
Transcribed Image Text:1. Consider a monopolistic firm operating in three different markets. Its revenue and cost functions:
R = R₁ (Q₁) + R₂(Q2) + R3(Q3)
C = C(Q) where Q = Q₁ + Q₂ + Q3.
a.
b.
C.
e.
Define the profit maximization problem of the firm.
Present the first-order condition (set of equations).
d. Present the Hessian of the firm's objective function.
Analyze the second-order sufficient condition.
Assume each of the revenue functions is concave and convex costs. Would this structure
secure the second-order condition? Explain.
f.
Provide an economic interpretation to the first order condition. In particular, connect marginal
revenues and demand elasticities to explain under what conditions the firm will charge a higher
price.
Solution
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, economics and related others by exploring similar questions and additional content below.Recommended textbooks for you


Principles of Economics (12th Edition)
Economics
ISBN:
9780134078779
Author:
Karl E. Case, Ray C. Fair, Sharon E. Oster
Publisher:
PEARSON

Engineering Economy (17th Edition)
Economics
ISBN:
9780134870069
Author:
William G. Sullivan, Elin M. Wicks, C. Patrick Koelling
Publisher:
PEARSON


Principles of Economics (12th Edition)
Economics
ISBN:
9780134078779
Author:
Karl E. Case, Ray C. Fair, Sharon E. Oster
Publisher:
PEARSON

Engineering Economy (17th Edition)
Economics
ISBN:
9780134870069
Author:
William G. Sullivan, Elin M. Wicks, C. Patrick Koelling
Publisher:
PEARSON

Principles of Economics (MindTap Course List)
Economics
ISBN:
9781305585126
Author:
N. Gregory Mankiw
Publisher:
Cengage Learning

Managerial Economics: A Problem Solving Approach
Economics
ISBN:
9781337106665
Author:
Luke M. Froeb, Brian T. McCann, Michael R. Ward, Mike Shor
Publisher:
Cengage Learning

Managerial Economics & Business Strategy (Mcgraw-…
Economics
ISBN:
9781259290619
Author:
Michael Baye, Jeff Prince
Publisher:
McGraw-Hill Education