Consider the equation for kinetic energy: KE = 1/2mv^2 = 1/2 * m * v^2. If I ask you to take the derivative of kinetic energy, you should ask "the derivative with respect to what?" a) Suppose mass m is constant. Compute the derivative of KE with respect to v, (d(KE)/dv). b) Who takes derivatives with respect to velocity? No one. Except you, just now. Sorry. The rate of change of energy with respect to time is more important: it is the Power. Now, consider velocity v to be a function of time, v(t). We will rewrite KE showing this time dependance: KE= 1/2 * m * v(t)^2. Show that (d(KE)/dt) = F(t)v(t). Hint: use Newton's second law, F = ma, to simplify. c) In the computation above, we assumed m was constant, and v was changing in time. Think of a physical situation in which both m and v are varying in time. d) Compute the Power when both mass and velocity are changing in time. (First rewrite KE(t) showing time dependence, then compute (d(KE)/dt).
Consider the equation for kinetic energy: KE = 1/2mv^2 = 1/2 * m * v^2. If I ask you to take the derivative of kinetic energy, you should ask "the derivative with respect to what?"
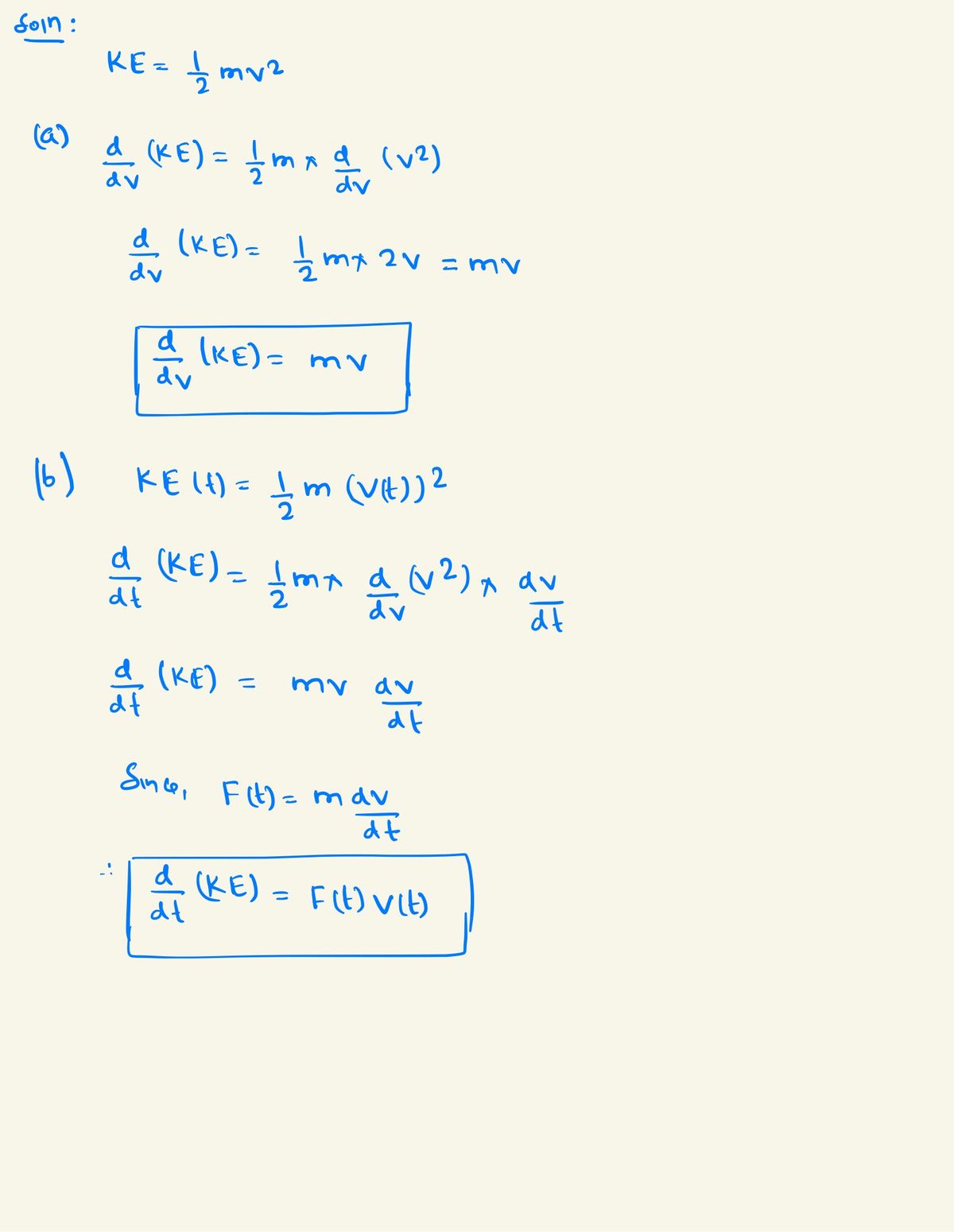
a) Suppose mass m is constant. Compute the derivative of KE with respect to v, (d(KE)/dv).
b) Who takes derivatives with respect to velocity? No one. Except you, just now. Sorry.
The rate of change of energy with respect to time is more important: it is the Power. Now, consider velocity v to be a function of time, v(t). We will rewrite KE showing this time dependance: KE= 1/2 * m * v(t)^2. Show that (d(KE)/dt) = F(t)v(t). Hint: use Newton's second law, F = ma, to simplify.
c) In the computation above, we assumed m was constant, and v was changing in time. Think of a physical situation in which both m and v are varying in time.
d) Compute the Power when both mass and velocity are changing in time. (First rewrite KE(t) showing time dependence, then compute (d(KE)/dt).
Step by step
Solved in 2 steps with 2 images









