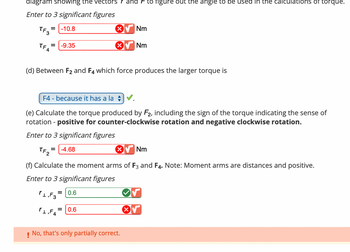
### Torque and Moment Arm Calculations#### (c) Calculating Torques for \( F_3 \) and \( F_4 \)Calculate the torques produced by \( F_3 \) and \( F_4 \), including the sign of the torque to indicate the sense of rotation: *positive for counter-clockwise rotation* and *negative for clockwise rotation*.**Help:** When using the formula for torque, consider the angle between the tails of the two vectors involved. Draw a diagram showing the vectors \( \vec{r} \) and \( \vec{F} \) to figure out the angle to use in torque calculations.- **Enter to 3 significant figures** \( \tau_{F_3} = \) \(\_\_\_\) Nm \( \tau_{F_4} = \) \(\_\_\_\) Nm*(Note: Between \( F_3 \) and \( F_4 \), selection for the larger torque is crossed out with a black scribble.)*#### (e) Calculating Torque for \( F_2 \)Calculate the torque produced by \( F_2 \), specifying the sign for rotation: *positive for counter-clockwise rotation* and *negative for clockwise rotation*.- **Enter to 3 significant figures** \( \tau_{F_2} = \) \(\_\_\_\) Nm#### (f) Calculating Moment Arms for \( F_3 \) and \( F_4 \)Calculate the moment arms for \( F_3 \) and \( F_4 \). Note that moment arms are distances and always positive.- **Enter to 3 significant figures** \( r_{\perp, F_3} = \) \(\_\_\_\) m \( r_{\perp, F_4} = \) \(\_\_\_\) m **Title: Torque and Rotation Analysis of a Meter Rule**A meter rule is anchored at the point O and is free to rotate about an axis passing through O, perpendicular to the plane of the meter rule. Forces \( F_1, F_2, F_3, F_4, \) and \( F_5 \), of equal magnitudes, act on the ruler at different locations. The magnitude of each force is 25.0 N and \( \theta = 30^\circ \). The distances along the ruler are given in cm. Assume all quantities are correct to 3 significant figures.**Diagram Explanation:**1. **Anchor Point (O):** The meter rule is anchored at point O, allowing it to rotate.2. **Forces:** - \( F_1 \) is acting vertically downward at 0 cm. - \( F_2 \) is acting at 30 cm, forming a \( 30^\circ \) angle with the vertical. - \( F_3 \) is acting vertically downward at 60 cm. - \( F_4 \) is acting at 60 cm, forming a \( 30^\circ \) angle with the vertical. - \( F_5 \) is acting horizontally to the right at 100 cm.3. **Tasks:** - **(a)** Determine whether each force can or cannot rotate the ruler and select the correct rationale. - **(b)** Identify which force between \( F_2 \) or \( F_4 \) produces a larger torque. - **(c)** Calculate the torques produced by \( F_3 \) and \( F_4 \), including the sign of the torque indicating the sense of rotation: positive for counter-clockwise rotation and negative for clockwise rotation. A hint is provided regarding the angle between two vectors. - **(d)** Determine which force between \( F_2 \) and \( F_4 \) produces the larger torque.**Interactive Elements:**- Dropdown menus to select the rationale for each force's ability to rotate the ruler.- Input fields for calculated torques (\( T_{F_3} \) and \( T_{F_4} \)) to three significant figures.This exercise helps understand the concepts of torque and rotational equilibrium by analyzing the forces and angles involved.

Resolve the components of F4. Vertical component is F4 cos = 18*cos(30) = Vertical component of F4 is 9NAnd horizontal component is F4sin=18*sin(30) = 18*0.5=9NHorizontal component of F4 is 9NNow torque OR where sin is the angle between r (the position at which force is applied from O) and F (the component of force responsible for turning effect)Therefore F3=-10.8N-m (negative sign as the rotation is clockwise) (vertical component will cause the ruler to rotate and not the horizontal component, horizontal component is acting along +ve x axis so its actually trying to pull out ruler from O)so F4=-9.35NmF2 is acting at distance r = 30cm = 0.3mvertical component of F2=F2cos30 = 9TF2=0.3*9=-4.68Nm clockwise.Moment arms of F3 and F4 is 60cm or 0.6mAccording to guidelines we can solve only first three subparts.C)Tf3=-10.8N-mTf4=-9.35N-me)Tf2=-4.68N-mF)moment arm of F3 and F4 is 60cm=0.6m