b) Suppose that a sequence of mutually independent and identically distributed continuous random variables X₁, X2, X3,..., Xn has the probability density function i) 1 √2π (x-0)² 2 foro < x <∞ elsewhere Show that f(x; 0) belongs to the one-parameter regular exponential family. Clearly indicate the following functions, h(x), c(0), w(0) and t(x). f(x; 0) = e
Q: n Show that for any &> 0 and S₁ = 1X₁, lim P(|S₁ - 01 ≥ ) = 0. Show that a statistic S, in a) is the…
A: We will use Chebychev's inequality to calculate the required limit of probability. To obtain the MLE…
Q: elsewhere i) Show that f(x; 0) belongs to the one-parameter regular exponential family. Clearly…
A:
Q: Compute P(B|A) and P(A|B).
A: f(x) is the probability density function of some random process. Suppose A = [−3, 3] ∪ [6, 12] is…
Q: Let X be a continuous random variable with the following PDF fx(2) = {** Į ce to z 20 otherwise…
A:
Q: 1) Let X₁, X₂, X3....X₁ be a sequence of independent and identically distributed with the following…
A: Given X~N(mean=θ, variance σ 2=1)
Q: 1. Let X be a continuous random variable with probability density function Compute E(X). f(x) = 0…
A:
Q: Let X be a continuous random variable with probability density function 2x f(x; 0) = () e-²/0 for x…
A: Given density function of X is, fx=2xθe-x2θ, x>0 Consider, EX2=∫0∞x2fxdx…
Q: Suppose that a sequence of mutually independent and identically distributed discrete random…
A: Given X~Pois(θ) Mean=variance=θ X1,X2,..Xn be the independent variables Note: According to Bartleby…
Q: Choose two numbers, n¡ and n, randomly between 0 and 1000 and determine c 2) such that f(x) = cx…
A: Let n1=50 and n2=60. Then, to find c, use the condition of valid probability distribution function…
Q: Find the mean and variance of the continuous random variable X with probability density function…
A:
Q: Let X be a random variable with probability density function S c(8x – a?) if \ 0 8
A:
Q: Estimate 6 by the method of moments estimator.
A: In method of moments we equate the sample moments and population moments to get the required…
Q: b) Suppose that a sequence of mutually independent and identically distributed continuous random…
A:
Q: Show that for any &> 0 and Sn = 1X₁, lim P(|S₁ − 0| ≥ ɛ) = 0. n-00
A: We will use Chebychev's inequality to find the limit of given probability.
Q: 1) Let X₁, X₂, X3,... X be a sequence of independent and identically distributed with the following…
A: Given that For given pdf we have to proof that it is a one parameter regular exponential family of…
Q: Show that the function defined as follows is a probability density function on the given interval;…
A:
Q: D. A Student says that since -1/2 is "in the middle" of the non-zero values that P(X > -1/2) = P(X…
A:
Q: Let X1, X2,..., X, be a random sample from a U (0, 0) population. (a) Find the probability density…
A: Hello! As you have posted more than 3 sub parts, we are answering the first 3 sub-parts. In case…
Q: Let X1,..., Xn be a random sample from a population with probability density function (pdf) f(x | 0)…
A: We have given that Let X 1 ,...,X n be a random sample from a population with probability density…
Q: 1) Let X₁, X2, X3, ... Xn be a sequence of independent and identically distributed with the…
A: As per our guidelines I can solve only first 3 subparts. Kindly post the remaining subpart again and…
Q: Suppose an electric-vehicle manufacturing company estimates that a driver who commutes 50 miles per…
A: Given, A random variable X~∪70,110
Q: Suppose that X1, X2, and X3 are i.I.d random variables each with density function f(x)= {((1/4)x^3)…
A: We want to find the expected value of M e.g E(M)= ?
Q: Find E[x] , P[A] , px|A(X) and E [x | A]. b) Let y=x2.Find E[y] and Var[y] .
A:
Q: Show that for any e > 0 and S, 1X₁, lim P(|S₁-81 ≥ 2) = 0. y'a 3140
A: We will use Chebychev's inequality to find the required limit of probability.
Q: i) the value of w that makes f(x₁, x₂) a probability density function. ii) the joint cumulative…
A:
Q: Suppose that the probability density function of x is f(x) = 0, (3x², 0 (2/3)) b) Determine the…
A:
Q: Without using MGF technique, prove or disapprove that is a pivotal quantity
A:
Q: b) Suppose that X₁ and X₂ have the joint probability density function defined as (WX1X2, f(x₁,x₂) =…
A:
Q: e n Show that for any &> 0 and S, ₁X₁, lim P(S₁-0 ≥ 8) = 0. =
A: We will use Chebychev's inequality to find the required limit of probability.
Q: b) Suppose that a sequence of mutually independent and identically distributed continuous random…
A:
Q: Suppose that X1, X2, . . . , X7 are i.i.d. random variables, each with density function f(x)=…
A: In question, We have given X1, X2, . . . , X7 are i.i.d random variables having pdf f(x). And M is…
Q: b) Suppose that X₁ and X₂ have the joint probability density function defined as f(x1, x₂) =…
A: Given The joint pdf of X1 and X2: f(x1,x2) = wx1x2, 0 <x1< 1, 0 < x2 < 1
Q: Let X1, X2, ..., Xn be a sample of n units from a population with a probability density function f…
A:
Q: 0 <x < 1 2x, f(x) =D { %3D 0, otherwise.
A: Solution: From the given information,
Q: ) Show that = ₁₁X₁ is the maximum likelihood estimator of the parameter 0. Zi=1
A:
Q: If X is uniformly distributed over [-1, 2], find | () the cumulative distribution function of Yı =…
A:
Q: Show that for any >0 and S₁ = 1X₁, lim P(|S₁ - 0 ≥ ) = 0. ε n 71-00 Show that a statistic S, in a)…
A: To obtain the maximum likelihood estimator of θ we have to determine the likelihood and…
Q: The pdf of a continuous random variable is defined as f(x)= 3k/x3 over [10, ∞) and 0 elsewhere. Find…
A:
Q: Show that for any e > 0 and Sn = Show that a statistic S, in a) is the maximum likelihood estimator…
A: The given probability function is of Poisson distribution with parameter θ due to the form of given…
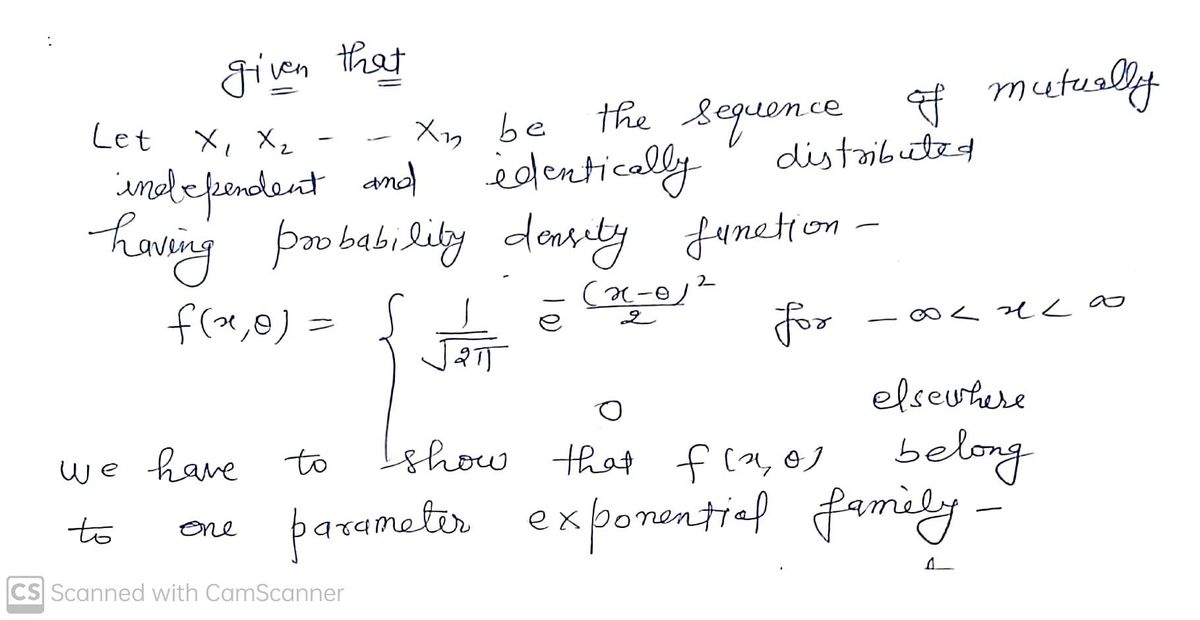
Given that
Step by step
Solved in 2 steps with 2 images
- Suppose that a sequence of mutually independent and identically distributed discrete random variables X₁, X₂, X3, ..., Xn has the following probability density function 0xe-0 x! 0, a) b) c) f(x; 0) = for x = 0,1,2,... elsewhere 1 n Show that for any & > 0 and S₂ = ₁X₁, lim P(|S, − 0| ≥ ɛ) = 0. - Zi=1 n n→∞ Show that a statistic Sn in a) is the maximum likelihood estimator of the parameter 8. Let Ô₁ X₁+2X₂+2X3-X4 = 4 ¹ and Ô₂ = ²(X₁ + X₂ + X3 + X4) be two unbiased estimators of 8. Which one of the two estimators is more efficient?2. A random variable X has a probability density function (pdf) given by , 0 |X > ).Suppose that a sequence of mutually independent and identically distributed discrete random variables X₁, X₂, X3,..., X has the following probability density function (exe-e a) b) c) f(x; 0) = x! 0, " for x = 0,1,2,... elsewhere Show that for any &> 0 and S₂ = 1X₁, lim P(|S₂ - 0 ≥ ) = 0. 12-00 Show that a statistic S, in a) is the maximum likelihood estimator of the parameter 0. Let 0₁ = X₁+²x₂+2x₁-X and Ô₂ = (X₁ + X₂ + X3 + X4) be two unbiased estimators of 8. Which 4 one of the two estimators is more efficient? d) What is the Cramer-Rao lower bound for the variance of the unbiased estimator of the parameter 9? e) Use the one-parameter regular exponential family definition to find the functions, h(x), c(0), w(0) and t(x).
- 1) Let X₁, X₂, X3, ... Xn be a sequence of independent and identically distributed with the following probability density function 1-1/(x-0² e f(x; 0)=√√2π if - ∞ < x <∞o otherwise 0, a) Is f(x; 0) a member of one-parameter regular exponential family? If yes, write down the functions, h(x), c(0), w(0) and t(x). b) Find the maximum likelihood estimator of the parameter 0. c) Find the variance of the maximum likelihood estimator of the parameter 8. d) Which one between X and (X₁ + X₂ − 2X3 + 2X4) statistics is more efficient?Let X₁, X₂, X3,..., Xn denote a random sample of size n from the population distributed with the following probability density function: f) f(x; 0): = {CO.. ((0+1)xº, if 0b) Let X₁, X2, X3.....Xn be a random sample of n from population X distributed with the following probability density function: ze zo, f(x;0)=√2m0 0, (i) Find the parameter space of 0. (ii) Find the maximum likelihood estimator of 0. if -∞b) Suppose that X₁ and X₂ have the joint probability density function defined as f(x₁, x₂) = (x1x²,0x₁51, 0 ≤ x₂ ≤1 elsewhere Find: i) the value of w that makes f(x₁, x₂) a probability density function. ii) the joint cumulative distribution function for X₁ and X₂. iii) P (X₂ ≤ | X₂ ≤ ³).b) Suppose that a sequence of mutually independent and identically distributed continuous random variables X₁, X₂, X3X₁ has the probability density function 1 (x-8)² 2 for -00Suppose that a sequence of mutually independent and identically distributed discrete random variables X₁, X₂, X3, ..., Xn has the following probability density function a) b) c) f(x; 0) = 0xe-0 x! 0, J for x = 0,1,2,... elsewhere Show that for any & > 0 and S₂ = = =₁ X₁, lim P(|S₂ − 0| ≥ ɛ) = 0. n n-00 one of the two estimators is more efficient? Show that a statistic S₁ in a) is the maximum likelihood estimator of the parameter 8. Let Ô₁ = X1+2X2+2X3-X4 4 ¹ and Ô₂ = ²(X₁ + X₂ + X3 + X4) be two unbiased estimators of 0. WhichThe time, X, for clearing side effects of water purification, in days, has the following cumulative distribution F: 1 F(x) = 0 for x 9 2 a) What is the probability density function for X for 1 5? c) What is the probability X 7? e) What is the probability that X > 6.3? f) What is the probability that X > 7 given the X > 6.3? g) Calculate the 70th percentile of X. h) What is the expected value of X? i) What is the expected value of x2 ? j) What is the variance of X? k) What is the probability that X is more than 0.1 below its expected value?1. Consider a random variable X with probability density function given as: 0 if x < 0, 1 if 0 ≤ x ≤ 1, f(x) 2 1 if 1 < <∞. -2x² Verify if f(x) is indeed a density function. If it is, find the distribution function of X.Recommended textbooks for youA First Course in Probability (10th Edition)ProbabilityISBN:9780134753119Author:Sheldon RossPublisher:PEARSON
A First Course in Probability (10th Edition)ProbabilityISBN:9780134753119Author:Sheldon RossPublisher:PEARSON