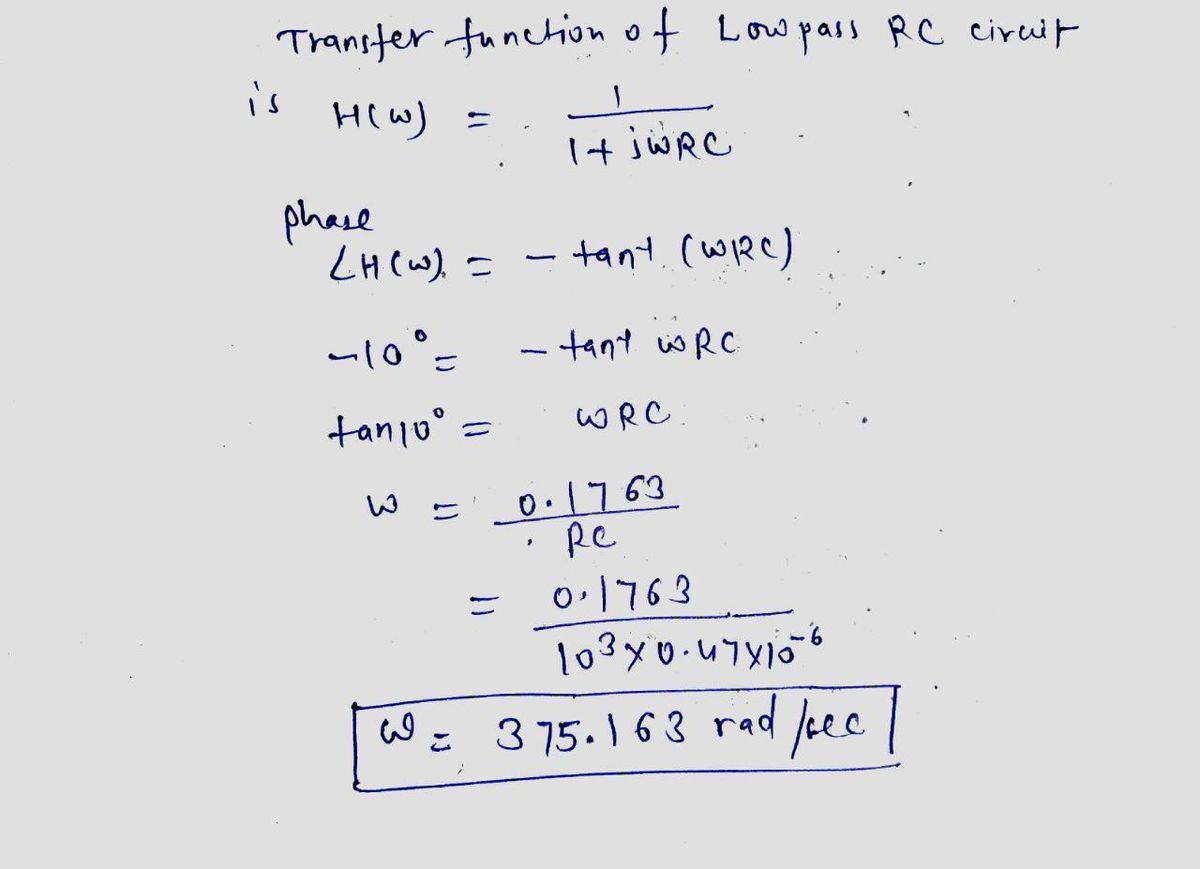
At what frequency is the phase shift introduced by the circuit of Example 6.7 equal to -10 degrees.
At what frequency is the phase shift introduced by the circuit of Example 6.7 equal to -10 degrees.
Introductory Circuit Analysis (13th Edition)
13th Edition
ISBN:9780133923605
Author:Robert L. Boylestad
Publisher:Robert L. Boylestad
Chapter1: Introduction
Section: Chapter Questions
Problem 1P: Visit your local library (at school or home) and describe the extent to which it provides literature...
Related questions
Question
At what frequency is the phase shift introduced by the circuit of Example 6.7 equal to -10 degrees.
![**Example 6.7: Frequency Response of RC Low-Pass Filter**
**Problem:**
Compute the response of the RC filter of Figure 6.20 to sinusoidal inputs at the frequencies of 60 and 10,000 Hz.
**Solution:**
**Known Quantities:**
\( R = 1 \, \text{k}\Omega \); \( C = 0.47 \, \mu F \); \( v_i(t) = 5 \cos(\omega t) \) V.
**Find:**
The output voltage \( v_o(t) \) at each frequency.
**Assumptions:**
None.
**Analysis:**
In this problem, the input signal voltage and the frequency response of the circuit (equation 6.43) are known, and the output voltage must be found at two different frequencies. If the voltages are represented in phasor form, the frequency response can be used for calculation:
\[
\frac{V_o(j\omega)}{V_i} = H_V(j\omega) = \frac{1}{1 + j\omega CR}
\]
\[
V_o(j\omega) = H_V(j\omega)V_i(j\omega) = \frac{1}{1 + j\omega CR} V_i(j\omega)
\]
The cutoff frequency of the filter is \( \omega_0 = 1/RC = 2,128 \, \text{rad/s} \) such that the expression for the frequency response in the form of equations 6.45 and 6.46 is:
\[
H_V(j\omega) = \frac{1}{1 + j(\omega/\omega_0)} \quad \big|H_V(j\omega)\big| = \frac{1}{\sqrt{1 + (\omega/\omega_0)^2}} \quad \Delta H(j\omega) = -\arctan\left(\frac{\omega}{\omega_0}\right)
\]
Next, recognize that at \( \omega = 60 \, \text{Hz} = 120\pi \, \text{rad/s} \), the ratio \( \omega/\omega_0 = 0.177 \). At \( \omega = 10,000 \, \text{Hz} = 20,000\pi \), \( \omega/\omega_0 =](/v2/_next/image?url=https%3A%2F%2Fcontent.bartleby.com%2Fqna-images%2Fquestion%2Fd85b24bb-dcb8-4da6-8613-614e9a19dac7%2F7387b235-63dd-451f-b0ee-6e7f44b6728d%2Fnlsnxv7_processed.jpeg&w=3840&q=75)
Transcribed Image Text:**Example 6.7: Frequency Response of RC Low-Pass Filter**
**Problem:**
Compute the response of the RC filter of Figure 6.20 to sinusoidal inputs at the frequencies of 60 and 10,000 Hz.
**Solution:**
**Known Quantities:**
\( R = 1 \, \text{k}\Omega \); \( C = 0.47 \, \mu F \); \( v_i(t) = 5 \cos(\omega t) \) V.
**Find:**
The output voltage \( v_o(t) \) at each frequency.
**Assumptions:**
None.
**Analysis:**
In this problem, the input signal voltage and the frequency response of the circuit (equation 6.43) are known, and the output voltage must be found at two different frequencies. If the voltages are represented in phasor form, the frequency response can be used for calculation:
\[
\frac{V_o(j\omega)}{V_i} = H_V(j\omega) = \frac{1}{1 + j\omega CR}
\]
\[
V_o(j\omega) = H_V(j\omega)V_i(j\omega) = \frac{1}{1 + j\omega CR} V_i(j\omega)
\]
The cutoff frequency of the filter is \( \omega_0 = 1/RC = 2,128 \, \text{rad/s} \) such that the expression for the frequency response in the form of equations 6.45 and 6.46 is:
\[
H_V(j\omega) = \frac{1}{1 + j(\omega/\omega_0)} \quad \big|H_V(j\omega)\big| = \frac{1}{\sqrt{1 + (\omega/\omega_0)^2}} \quad \Delta H(j\omega) = -\arctan\left(\frac{\omega}{\omega_0}\right)
\]
Next, recognize that at \( \omega = 60 \, \text{Hz} = 120\pi \, \text{rad/s} \), the ratio \( \omega/\omega_0 = 0.177 \). At \( \omega = 10,000 \, \text{Hz} = 20,000\pi \), \( \omega/\omega_0 =

Transcribed Image Text:**Chapter 6: Frequency Response and System Concepts**
---
This section discusses the frequency response of an RC filter as highlighted in Example 6.7. The analysis is depicted through two graphs: the magnitude response and the phase response.
**Magnitude Response of RC Filter (Example 6.7)**
- **Graph Description**: The magnitude response graph plots the amplitude ratio against the radian frequency (in rad/s) on a logarithmic scale. The x-axis covers frequencies from \(10^0\) to \(10^6\) rad/s, while the y-axis represents the amplitude ratio from 0 to 1.0.
- **Details**: The graph indicates a high amplitude at lower frequencies, which gradually decreases as the frequency increases, forming a classic low-pass filter response.
**Phase Response of RC Filter (Example 6.7)**
- **Graph Description**: The phase response graph displays the phase shift in degrees against the radian frequency (in rad/s), again on a logarithmic scale. The x-axis is similar to the magnitude response, ranging from \(10^0\) to \(10^6\) rad/s, while the y-axis ranges from -100 to 0 degrees.
- **Details**: The phase shift starts from near 0 degrees at low frequencies and progressively shifts to approximately -90 degrees as the frequency increases.
**Figure 6.24: Response of RC Filter of Example 6.7**
These graphs help in understanding the behavior of the RC filter, showcasing how it allows low frequencies to pass with minimal attenuation while reducing the amplitude and altering the phase of higher frequencies.
Expert Solution
Step 1
Step by step
Solved in 2 steps with 1 images

Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, electrical-engineering and related others by exploring similar questions and additional content below.Recommended textbooks for you

Introductory Circuit Analysis (13th Edition)
Electrical Engineering
ISBN:
9780133923605
Author:
Robert L. Boylestad
Publisher:
PEARSON

Delmar's Standard Textbook Of Electricity
Electrical Engineering
ISBN:
9781337900348
Author:
Stephen L. Herman
Publisher:
Cengage Learning

Programmable Logic Controllers
Electrical Engineering
ISBN:
9780073373843
Author:
Frank D. Petruzella
Publisher:
McGraw-Hill Education

Introductory Circuit Analysis (13th Edition)
Electrical Engineering
ISBN:
9780133923605
Author:
Robert L. Boylestad
Publisher:
PEARSON

Delmar's Standard Textbook Of Electricity
Electrical Engineering
ISBN:
9781337900348
Author:
Stephen L. Herman
Publisher:
Cengage Learning

Programmable Logic Controllers
Electrical Engineering
ISBN:
9780073373843
Author:
Frank D. Petruzella
Publisher:
McGraw-Hill Education

Fundamentals of Electric Circuits
Electrical Engineering
ISBN:
9780078028229
Author:
Charles K Alexander, Matthew Sadiku
Publisher:
McGraw-Hill Education

Electric Circuits. (11th Edition)
Electrical Engineering
ISBN:
9780134746968
Author:
James W. Nilsson, Susan Riedel
Publisher:
PEARSON

Engineering Electromagnetics
Electrical Engineering
ISBN:
9780078028151
Author:
Hayt, William H. (william Hart), Jr, BUCK, John A.
Publisher:
Mcgraw-hill Education,