At a post office, a parcel that is an m kg box slides down a ramp inclined at an angle θ with the horizontal, as shown. The coefficient of kinetic friction between the box and the ramp is μK, and the coefficient of static friction for the same is μS. Notice that the coordinate axes have been chosen with the x axis directed up the incline, as shown. Part (b) Input an expression for the net force in the x direction consistent with the coordinate axes on the diagram. Express your answer in terms of the mass of the box, m, the acceleration due to gravity, g, the angle of the incline, θ, the magnitude of the normal vector FN, and appropriate coefficients of friction. The coefficient of kinetic friction between the box and the ramp is μK, and the coefficient of static friction for the same is μS. Part (c) Input an expression for the net force in the y direction consistent with the coordinate axes on the diagram. Express your answer in terms of the mass of the box, m, the acceleration due to gravity, g, the angle of the incline, θ, and the magnitude of the normal vector FN. Part (d) Input an expression for the magnitude of the normal force. Express your answer in terms of the mass of the box, m, the acceleration due to gravity, g, and the angle of the incline, θ. Part (e) Input an expression for the acceleration of the box in the x direction consistent with the coordinate axes on the diagram. Express your answer in terms of the mass of the box, m, the acceleration due to gravity, g, the angle of the incline, θ, and appropriate coefficients of friction. The coefficient of kinetic friction between the box and the ramp is μK, and the coefficient of static friction for the same is μS. Part (f) Enter a numeric answer for the magnitude of the acceleration in meters per squared seconds when the angle of the incline is 28° and the coefficient of kinetic friction is 0.035. Part (g) Using the numbers from the previous step, give a numeric answer for the time in seconds that elapse when the package, initially at rest, travels a distance of 2.25 m down the ramp. Part (h) Using the numbers from the previous steps, give a numeric answer for the speed of the package at the moment it has traveled a distance of 2.25 m down the ramp.
Problem 6: At a post office, a parcel that is an m kg box slides down a ramp inclined at an angle θ with the horizontal, as shown. The coefficient of kinetic friction between the box and the ramp is μK, and the coefficient of static friction for the same is μS. Notice that the coordinate axes have been chosen with the x axis directed up the incline, as shown.
Part (b) Input an expression for the net force in the x direction consistent with the coordinate axes on the diagram. Express your answer in terms of the mass of the box, m, the acceleration due to gravity, g, the angle of the incline, θ, the magnitude of the normal
Part (c) Input an expression for the net force in the y direction consistent with the coordinate axes on the diagram. Express your answer in terms of the mass of the box, m, the acceleration due to gravity, g, the angle of the incline, θ, and the magnitude of the normal vector FN.
Part (d) Input an expression for the magnitude of the normal force. Express your answer in terms of the mass of the box, m, the acceleration due to gravity, g, and the angle of the incline, θ.
Part (e) Input an expression for the acceleration of the box in the x direction consistent with the coordinate axes on the diagram. Express your answer in terms of the mass of the box, m, the acceleration due to gravity, g, the angle of the incline, θ, and appropriate coefficients of friction. The coefficient of kinetic friction between the box and the ramp is μK, and the coefficient of static friction for the same is μS.
Part (f) Enter a numeric answer for the magnitude of the acceleration in meters per squared seconds when the angle of the incline is 28° and the coefficient of kinetic friction is 0.035.
Part (g) Using the numbers from the previous step, give a numeric answer for the time in seconds that elapse when the package, initially at rest, travels a distance of 2.25 m down the ramp.
Part (h) Using the numbers from the previous steps, give a numeric answer for the speed of the package at the moment it has traveled a distance of 2.25 m down the ramp.

As per expert Guidelines we are supposed to answer only the first three parts of the given multipart Question. sorry for the inconvenience caused. 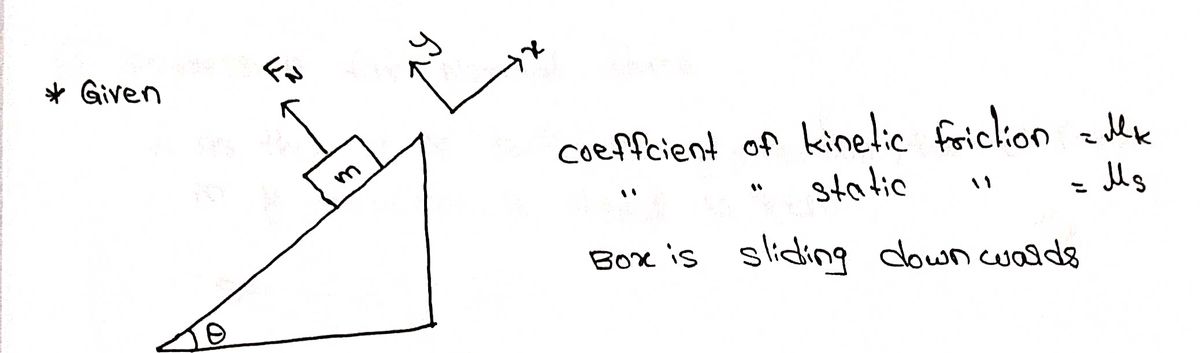
Trending now
This is a popular solution!
Step by step
Solved in 3 steps with 3 images









