Assume that you measure the dimensions of a hollow cube with vernier caliper many time and obtain the following average values in cm: Height: 4 Inner width: 2.23 Outer width: 7.02 Inner length: 1 Outer length: 1 Using these values, calculate the volume of the hollow cube in cm³. Express your result with 3 significant figures. Answer:
Q: The edges of a shoebox are measured to be 11.3 cm, 19.4 cm, and 29 cm.
A: Given: length =11.3 cm Width= 19.4 cm Height = 29 cm
Q: The depth of the ocean is sometimes measured in fathoms (1 fathom = 6 feet). Distance on the surface…
A:
Q: A sphere of radius r has surface area A = 4πr2 and volumeV = (4/3)πr3. If the radius of sphere 2 is…
A: a)The ratio of the areas, A2/A1
Q: (a) A pyramid has a height of 461 ft and its base covers an area of 12.0 acres (see figure below).…
A:
Q: Calculate the value of the error with one decimal place for the multiplication of x and y: z=xxy…
A:
Q: A) If the drop is a cube, what is its surface area? (Hint, volume of a cube = L3. Surface area of a…
A: Volume of the drop is V = 2 cm3 Length of te cube be L Radius of the sphere be R.
Q: The edges of a shoebox are measured to be 10.9 cm, 18.4 cm, and 29 cm. Determine the volume of the…
A: Given Data: The length of a shoebox is, l=29 cm The breadth of a shoebox is, b=18.4 cm The height of…
Q: the A partly-full paint can has 0.493 U.S. gallons of paint left in it. (a) What is the volume of…
A:
Q: Water has a density of 1.00 Mg/m3. What is the density expressed in imperial units? Express your…
A: To convert the density of water from metric units (Mg/m^3) to imperial units (slugs/ft^3), you can…
Q: Suppose 1.5 cubic meter of oil is spilled into the ocean. Find the area of the resulting slick,…
A: Finding the total area occupied by the spilled oil.Given information:The volume of the oil spilled…
Q: fine grains of beach sand are assumed to be spheres of radius a. 45.7 μm. These grains are made of…
A: Given data: The diameter of each grain is, The density of the silicon dioxide grain is, Formula…
Q: The diameter of a sphere is measured to be 6.12 in. (a) Find the radius of the sphere in…
A: diameter of the sphere d = 6.12 in
Q: The micrometer (1 µm) is often called the micron. (a) How many microns make up 4.0 km? | um (b) What…
A: Given 1μm=10-6 m
Q: Estimate the number of breaths taken by a human being during an average lifetime. (Hint: Show…
A:
Q: Problem 6: The sides of a small rectangular box are measured to be 1.55 + 0.045 cm, 2.1 + 0.025cm,…
A:
Q: Estimate the mass of a human kidney if it is roughly a sphere with a radius of 4.29 cm. Answer in…
A: Hi, you have submitted multiple questions and it is not specified in which problem you need help.…
Q: (1) You are given a cylinder with true dimensions: h = 2.00 in and d = 1.50 in. Suppose you make a…
A: Step 1: Step 2: Step 3: Step 4:
Q: Two types of barrel units were in use in the 1920s in the United States. The apple barrel had a…
A: Solution: Given: 1 in3 = 0.0163871 liter V1 = 1 apple-barrel = 7056 in3 V2 = 1 cranberry-barrel =…
Q: Consider a rectangle with a measured width of ? = 9.6 ± 0.3 cm and a length of ? = 30.2 ± 0.7 cm.…
A:
Q: A typical human cell is approximately 12.00 μm in diameter and enclosed by a membrane that is 9.000…
A: Given:- A typical human cell has an approximate in diameter of d= 12.00 μm The thickness of the…
Q: A spherical balloon is partially blown up and its surface area is measured. More air is then added,…
A: Given: Surface area of the balloon expands by a factor of 9.90
Q: Morty measures a Meeseeks box (an object that is roughly a cube) with an old rusty ruler. Because of…
A: Given that, Width (W)=20.2 cm=0.202 m Height (H)=19 cm=0.19 mDepth(D)=20.31 cm=0.2031 mMass(M)=7.401…
Q: A pyramid has a square base of side x and height h. The volume V of the pyramid is given by the…
A: We are given volume of pyramid. We are given uncertainty in height. We are also given uncertainty in…
Q: 9. Grains of California beach sand are approximately spheres with a radius of, on average, 50 µm.…
A: 9. Let the given grain’s radius be denoted by R and let the given cube’s side length be denoted by…
Q: You've been asked to calculate the mass of a conical pile of sawdust measuring 10 meters high with a…
A: Solution:- We are given with height of a conical pile of…
Q: Estimate the number of tennis ball that would fit into a room that is 4m long, 4m wide, and 3m high.…
A:
Q: a be Your Answer: C6 C₂ x10 C3 C5 In the figure C₁ = 12 μF, C₂ = 14 μF, C3 = 18 μF, C4 = 18 μF, C5 =…
A:
Q: An open metal bucket is in the form of a frustum cone with a height of 21 cm and a radii of its…
A: Given Frustum cone height, h= 21 cm Upper end radius , R = 20 cm Lower end radius , r = 10 cm…
Q: A lecture period (50.0 min) is close to 1.00 microcentury. (a) How long is a microcentury? (b) Usin…
A: (a)we know that,minutes in 1 century=52.6×106 minutesminutes in 1 micro century= 52.6 minutes
Q: The density of the Earth can be expressed by the equation density = C{1.22 -(r/R)}, where R is the…
A: The mass can be represented as, M=∫0RρdV=∫0RC1.22-rR4πr2drM=4πC∫0R1.22r2-r3RdrM=4πC1.22r33-1Rr440R
Q: The length and width of a rectangular room are measured to be 3.885 ± 0.0065 m and 3.2 ± 0.0045 m.…
A: Given The length of a rectangular room = l = 3.885 ± 0.0065 m The width a rectangular room = b = 3.2…
Q: One mole of Gold (Au) atom has a mass of 197 g. We know that the density of gold is 19.30 g/cm3 in…
A:
Q: (a) The volume V of a growing spherical cell is V = ³, where the radius is measured in micrometers…
A: According to question we need to find--- (a) Find the average rate of change of V with respect to…

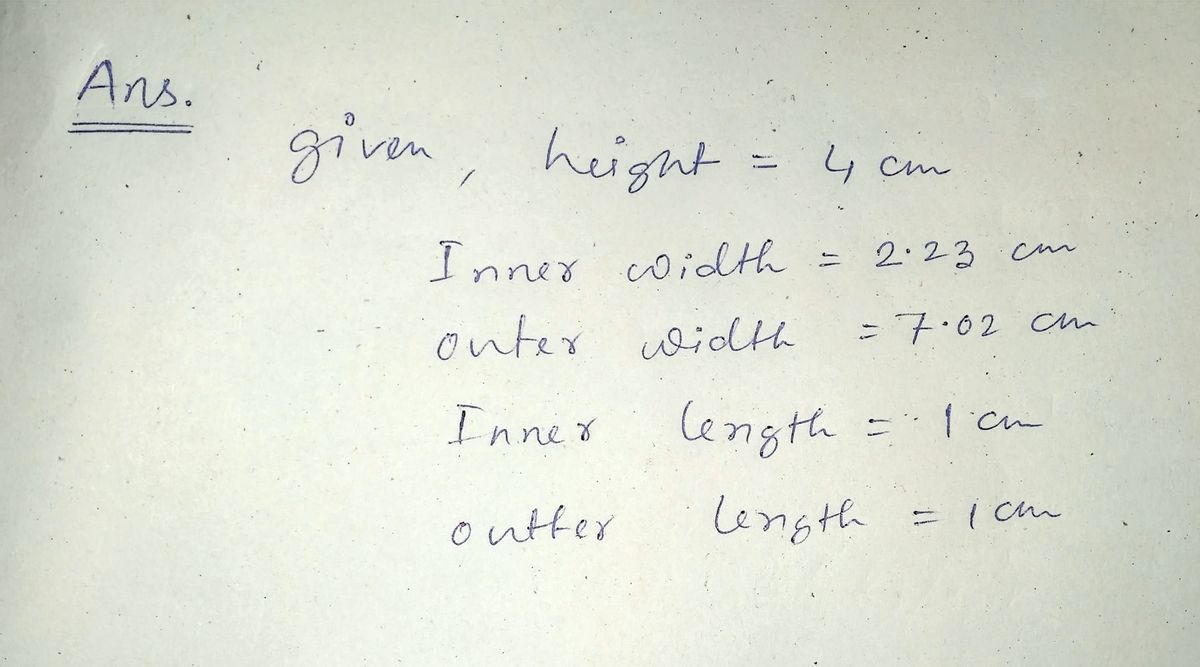
Step by step
Solved in 2 steps with 2 images

- The base of a pyramid covers an area of 12.8 acres (1 acre = 43,560 ft²) and has a height of 471 ft. If the volume of a pyramid is given by the expression V = bh/3, where b is the area of the base and h is the height, find the volume of this pyramid in cubic meters. m³ ⒸSylvain Grandadam/Photo Researchers, Inc.Physical units in mechanics are usually some combination of the dimensions time T, mass M, and length L. Consider the physical quantities m, r, u, a, and t with dimensions [m] = M, [r] = L, [u] = LT-, [a] = LT-2, and [1] = T. Enter the dimensional expression of the quantity on the right-hand side of each equation. Your answers may contain only M, L, T, and exponents. Assume that each of the following equations is dimensionally consistent. Lo = mur [Lo] = W = mar [W] = T3.
- You purchased 5.2kg of apples from a store. You noticed that they used the smallest division of 4.2 g to weight them. What is the relative error of this weight measurement as a percentage. You want to calculate the area of your dorm which is in a rectangular shape. You measure the length and the width of the room as L= 4.8+(-.01) m, W= 4.4+(-.01) m. What is the absolute error in the area in m^2 units?- Your answer is partially correct. A partly-full paint can has 0.515 U.S. gallons of paint left in it. (a) What is the volume of the paint, in cubic meters? (b) If all the remaining paint is used to coat a wall evenly (wall area = 12.1 m²), how thick is the layer of wet paint? Give your answer in meters. (a) Number i 1.949 (b) Number i 312.1 Units Units m^3 m^2Using a ruler, you measure the length of a rectangle to be 12.75 cm and the width to be 3.64 cm. Calculate the area of this rectangle and include the correct number of significant figures.
- A person's blood pressure is measured to be: 115 ± 7 mm Hg. What is its percent uncertainty? (Express your answer to the correct number of significant figures and proper units. Use unrounded values in your calculations.)Imagine a library : there are shelves 8 m high, filled with books on both sides, if the width of the shelves is 60 cm and the distance between the shelves (corridor) is 1.5 m. So first, calculate how many shelves to occupy an area of 3500 m2, how many books are on one shelf, and finally how many books are in total.... Information about the dimension of each book and also the length of the book shelves or other. Assume yourself Answer with the step thank U... Please no reject this question is complete im needed in 30-40 minutes thank uthe average density of an atom is approximately 103 kg/m3. The nucleus of an atom has a radius about 10-5 times that of the entire atom, and contains nearly all the mass of the atom. What is the approximate density, in kilograms per cubic meter, of a nucleus?
- NASA The radius of the planet Saturn is 5.85 × 107 m, and its mass is 5.68 x 1026 kg (Fig. P1.48). (a) Find the density of Saturn (its mass divided by its volume) in grams per cubic centimeter. (The volume of a sphere is given by (4/3) 3.) (b) Find the area of Saturn in square feet. (The surface area of a sphere is given by 4πr².) Figure P1.48 A view of Saturn.What is the percent error in the accuracy in the measured volume of the cube? Use the calculated value as the expected value. Round your answer to 2 digits.Calculate the approximate number of board feet (one board foot is defined as a volume of 1 in x 1 ft x 1 ft) of lumber that would be available in a log that has a diameter of 26 cm and a length of 2.4 m. Assume the log is a right cylinder and that the saw mill must make the log into a rectangular prism before cutting it into boards.