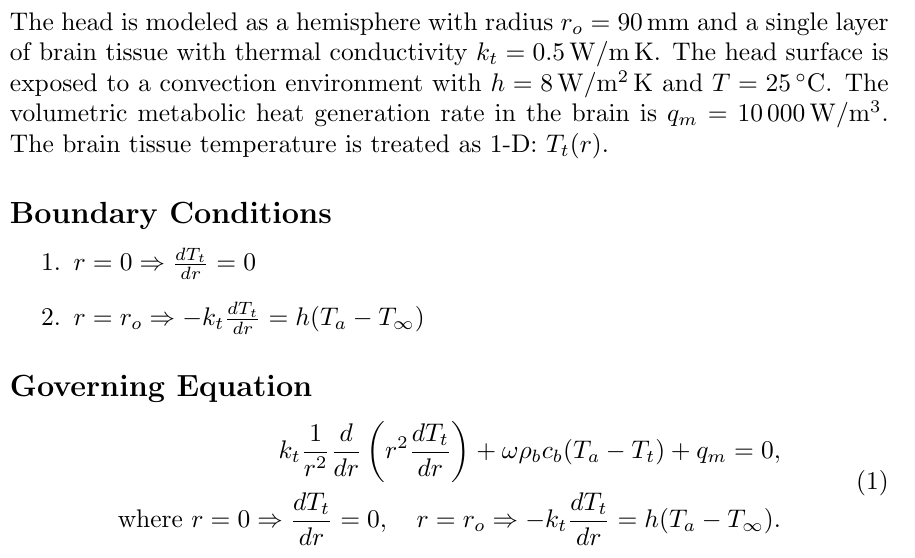
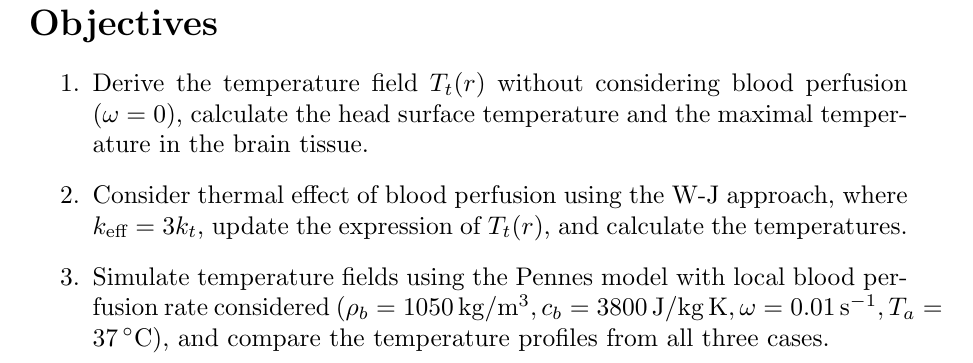
Assume that the head can be modeled as a hemisphere (radius ro-90 mm) with a single layer of brain tissue (k-0.5 W/m °C). The bottom of the hemisphere is insulated. The head surface is assumed exposed to a convection environment (h-8 W/m²K, T-25°C). Based on the boundary conditions, the brain tissue temperature can be treated as 1-D, i.e., T.(r), where r is the radial variable in the spherical coordinates. The volumetric metabolic heat generation rate in the brain is uniformly distributed and is equal to q-10000 W/m³. Consider two situations, a) no blood perfusion is considered; b) blood perfusion rate is uniform in the brain and is equal to -0.01 s¹. The governing equation (steady state, 1-D heat transfer in the spherical coordinate system, with or without the Pennes perfusion term) and boundary conditions for both situations can be written as: 1 d +@pc,(T-T)+q=0 k₁ dr dr 0 dT, r=r₁ k₁. h(T, -T.) dr (a) For the first situation without considering blood perfusion via letting -0 in the above equation, derive the temperature field T.(r). Use the values given to plot the radial temperature distribution T.(r) vs. r from Excel. Calculate the head surface temperature (r=ro) and the maximal temperature (r=0) in the brain tissue. (b) For the second situation, we consider thermal effect of blood perfusion using the W-J approach. Let @-0 in the above equation, however, change k, by keff when keff=3 kr (the W- r=0 dT, dr J approach). The derived expression of T.(r) in (a) can be easily updated via replacing k, by Keff Again plot the radial temperature distribution T,(r) vs. r from Excel. Calculate the head surface temperature (r=ro) and the maximal temperature (r=0) in the brain tissue. This is the simulation prediction using the WJ equation. (c) For the third situation, we will simulate temperature fields using the Pennes model, where the local blood perfusion rate is considered (p=1050 kg/m³, c-3800 J/kg °C, a 0.01 s¹, and Ta-37°C), the temperature distribution is derived and expressed as: T,(r)={ T. + + p.c, k, + 9₁ wp.c sinhl/m,ca/k, r) kr+sinh(√mp,c./k, r.). √ep,c./k,cosh(√mp,c./k, r.) if r*0 T ifr=0 @p,c Use Excel to plot the temperature distribution in the same plot as in (a), and compare the three curves. What is the role of the blood perfusion rate playing on the temperature profile? Do you think that the W-J model is good to predict the temperature profile? Note that in the W-J model, the surface temperature does not change from (a), explain briefly why.
Assume that the head can be modeled as a hemisphere (radius ro-90 mm) with a single layer of brain tissue (k-0.5 W/m °C). The bottom of the hemisphere is insulated. The head surface is assumed exposed to a convection environment (h-8 W/m²K, T-25°C). Based on the boundary conditions, the brain tissue temperature can be treated as 1-D, i.e., T.(r), where r is the radial variable in the spherical coordinates. The volumetric metabolic heat generation rate in the brain is uniformly distributed and is equal to q-10000 W/m³. Consider two situations, a) no blood perfusion is considered; b) blood perfusion rate is uniform in the brain and is equal to -0.01 s¹. The governing equation (steady state, 1-D heat transfer in the spherical coordinate system, with or without the Pennes perfusion term) and boundary conditions for both situations can be written as: 1 d +@pc,(T-T)+q=0 k₁ dr dr 0 dT, r=r₁ k₁. h(T, -T.) dr (a) For the first situation without considering blood perfusion via letting -0 in the above equation, derive the temperature field T.(r). Use the values given to plot the radial temperature distribution T.(r) vs. r from Excel. Calculate the head surface temperature (r=ro) and the maximal temperature (r=0) in the brain tissue. (b) For the second situation, we consider thermal effect of blood perfusion using the W-J approach. Let @-0 in the above equation, however, change k, by keff when keff=3 kr (the W- r=0 dT, dr J approach). The derived expression of T.(r) in (a) can be easily updated via replacing k, by Keff Again plot the radial temperature distribution T,(r) vs. r from Excel. Calculate the head surface temperature (r=ro) and the maximal temperature (r=0) in the brain tissue. This is the simulation prediction using the WJ equation. (c) For the third situation, we will simulate temperature fields using the Pennes model, where the local blood perfusion rate is considered (p=1050 kg/m³, c-3800 J/kg °C, a 0.01 s¹, and Ta-37°C), the temperature distribution is derived and expressed as: T,(r)={ T. + + p.c, k, + 9₁ wp.c sinhl/m,ca/k, r) kr+sinh(√mp,c./k, r.). √ep,c./k,cosh(√mp,c./k, r.) if r*0 T ifr=0 @p,c Use Excel to plot the temperature distribution in the same plot as in (a), and compare the three curves. What is the role of the blood perfusion rate playing on the temperature profile? Do you think that the W-J model is good to predict the temperature profile? Note that in the W-J model, the surface temperature does not change from (a), explain briefly why.
Elements Of Electromagnetics
7th Edition
ISBN:9780190698614
Author:Sadiku, Matthew N. O.
Publisher:Sadiku, Matthew N. O.
ChapterMA: Math Assessment
Section: Chapter Questions
Problem 1.1MA
Related questions
Question
![The image presents a detailed explanation of a thermal model for the human head, simplified as a hemisphere with a radius of \( r_0 = 90 \, \text{mm} \). The head is assumed to have a single layer of brain tissue characterized by a thermal conductivity \( k_t = 0.5 \, \text{W/m°C} \). The hemisphere's bottom is insulated, while the surface is exposed to a convective environment with \( h = 8 \, \text{W/m}^2\text{K} \) and ambient temperature \( T_a = 25^\circ \text{C} \).
### Situations Considered:
1. **Without Blood Perfusion**:
- The volumetric metabolic heat generation \( q_{\text{m}} = 10000 \, \text{W/m}^3 \).
- Governing equation:
\[
\frac{1}{r^2} \frac{d}{dr} \left( r^2 \frac{dT_f}{dr} \right) + \frac{\omega \rho_p c_b (T_a - T_f) + q_{\text{m}}}{k} = 0
\]
- Boundary conditions are:
- At \( r = 0 \): \(\frac{dT_f}{dr} = 0\)
- At \( r = r_0 \): \(-k \frac{dT_f}{dr} = h(T_f - T_a)\)
2. **With Uniform Blood Perfusion (\(\omega = 0.01 \, \text{s}^{-1}\))**:
- Using the W-J approach, modify:
- \( k_t \to k_{\text{eff}} = 3 \, k_t \)
3. **Considering Local Blood Perfusion using the Pennes Model**:
- Perfusion parameters:
- Density \( \rho_p = 1050 \, \text{kg/m}^3 \),
- Specific heat \( c_b = 3800 \, \text{J/kg°C} \),
- Blood perfusion rate \( \omega = 0.01 \, \text{s}^{-1} \),
- Arterial temperature \( T_a = 37^\circ \text{C} \).
- The temperature distribution](/v2/_next/image?url=https%3A%2F%2Fcontent.bartleby.com%2Fqna-images%2Fquestion%2Ff04af373-6616-44d4-9142-5aa7a1930a82%2Fbae020b5-92a2-4399-97ba-b2796db8d211%2Fx0tcq6_processed.jpeg&w=3840&q=75)
Transcribed Image Text:The image presents a detailed explanation of a thermal model for the human head, simplified as a hemisphere with a radius of \( r_0 = 90 \, \text{mm} \). The head is assumed to have a single layer of brain tissue characterized by a thermal conductivity \( k_t = 0.5 \, \text{W/m°C} \). The hemisphere's bottom is insulated, while the surface is exposed to a convective environment with \( h = 8 \, \text{W/m}^2\text{K} \) and ambient temperature \( T_a = 25^\circ \text{C} \).
### Situations Considered:
1. **Without Blood Perfusion**:
- The volumetric metabolic heat generation \( q_{\text{m}} = 10000 \, \text{W/m}^3 \).
- Governing equation:
\[
\frac{1}{r^2} \frac{d}{dr} \left( r^2 \frac{dT_f}{dr} \right) + \frac{\omega \rho_p c_b (T_a - T_f) + q_{\text{m}}}{k} = 0
\]
- Boundary conditions are:
- At \( r = 0 \): \(\frac{dT_f}{dr} = 0\)
- At \( r = r_0 \): \(-k \frac{dT_f}{dr} = h(T_f - T_a)\)
2. **With Uniform Blood Perfusion (\(\omega = 0.01 \, \text{s}^{-1}\))**:
- Using the W-J approach, modify:
- \( k_t \to k_{\text{eff}} = 3 \, k_t \)
3. **Considering Local Blood Perfusion using the Pennes Model**:
- Perfusion parameters:
- Density \( \rho_p = 1050 \, \text{kg/m}^3 \),
- Specific heat \( c_b = 3800 \, \text{J/kg°C} \),
- Blood perfusion rate \( \omega = 0.01 \, \text{s}^{-1} \),
- Arterial temperature \( T_a = 37^\circ \text{C} \).
- The temperature distribution
Expert Solution
Step 1: Given Data
Step by step
Solved in 3 steps with 4 images

Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, mechanical-engineering and related others by exploring similar questions and additional content below.Recommended textbooks for you

Elements Of Electromagnetics
Mechanical Engineering
ISBN:
9780190698614
Author:
Sadiku, Matthew N. O.
Publisher:
Oxford University Press

Mechanics of Materials (10th Edition)
Mechanical Engineering
ISBN:
9780134319650
Author:
Russell C. Hibbeler
Publisher:
PEARSON

Thermodynamics: An Engineering Approach
Mechanical Engineering
ISBN:
9781259822674
Author:
Yunus A. Cengel Dr., Michael A. Boles
Publisher:
McGraw-Hill Education

Elements Of Electromagnetics
Mechanical Engineering
ISBN:
9780190698614
Author:
Sadiku, Matthew N. O.
Publisher:
Oxford University Press

Mechanics of Materials (10th Edition)
Mechanical Engineering
ISBN:
9780134319650
Author:
Russell C. Hibbeler
Publisher:
PEARSON

Thermodynamics: An Engineering Approach
Mechanical Engineering
ISBN:
9781259822674
Author:
Yunus A. Cengel Dr., Michael A. Boles
Publisher:
McGraw-Hill Education

Control Systems Engineering
Mechanical Engineering
ISBN:
9781118170519
Author:
Norman S. Nise
Publisher:
WILEY

Mechanics of Materials (MindTap Course List)
Mechanical Engineering
ISBN:
9781337093347
Author:
Barry J. Goodno, James M. Gere
Publisher:
Cengage Learning

Engineering Mechanics: Statics
Mechanical Engineering
ISBN:
9781118807330
Author:
James L. Meriam, L. G. Kraige, J. N. Bolton
Publisher:
WILEY