Alphabet institute organizes workshop every month on organizational management for maximum 35 people. The interested people can reserve their seat online. Most of the time, even though the people reserve their seat, at the end some of them will cancel it. Keeping this situation in mind, the institute allows reserving 10% more than their maximum capacity. According to the past statistics around 9%/people cancel their seat at the last moment. Based on the given information: a. Calculate the probability that all the people who reserve the seat online can join the
Contingency Table
A contingency table can be defined as the visual representation of the relationship between two or more categorical variables that can be evaluated and registered. It is a categorical version of the scatterplot, which is used to investigate the linear relationship between two variables. A contingency table is indeed a type of frequency distribution table that displays two variables at the same time.
Binomial Distribution
Binomial is an algebraic expression of the sum or the difference of two terms. Before knowing about binomial distribution, we must know about the binomial theorem.
pls answer it quickly

(a). Obtain the probability that all the people who reserve the seat online will join the workshop:
It is given that the Alphabet institute organizes workshop for a maximum of 35 people.
The organization allows reservation for 10% more than their maximum capacity.
That is, the number of reservations will be 35 + (10% of 35) = 35 + 3.5 = 39.
It is given that, 9% of people will cancel their seats.
Consider the event that the people cancel their seat as a “success”. The probability of success in each trial is p = 0.09. Then q = 1 – 0.09 = 0.91.
To allocate all the people, 4 people should cancel their seats.
Consider X as the number of people who cancel their seats. Then, X has a Binomial distribution with parameters (n = 39, p = 0.09).
The probability that all the people who reserve the seat online will join the workshop is 0.1989 from the calculation given below:
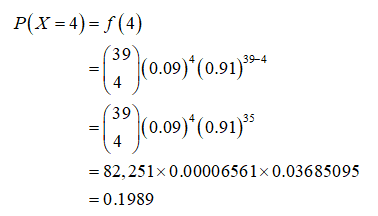
Thus, the probability that all the people who reserve the seat online will join the workshop is 0.1989.
(b). Obtain the probability that out of selected people at least 2 will not show up:
The probability that out of selected people at least 2 will not show up is 0.23246 from the calculation given below:
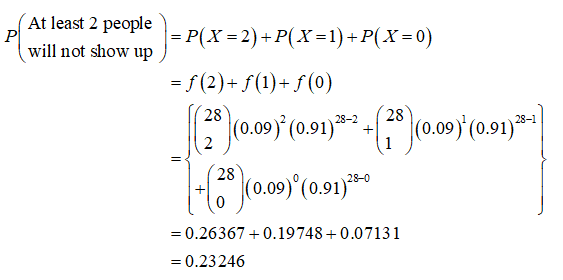
Thus, the probability that out of selected people at least 2 will not show up is 0.23246.
Step by step
Solved in 3 steps with 3 images




