**2. Determine if each relationship is a function. Justify your conclusion.** **(a) The equation: \( y = 4x \)** This equation represents a linear function. For every input \( x \), there is exactly one output \( y \). **(b) The equation: \( 2x + 3y = 12 \)** This is a linear equation. Solving for \( y \) gives \( y = \frac{12 - 2x}{3} \), which shows that for every \( x \), there is exactly one \( y \). Thus, it is a function. **(c) The graph:** A parabolic graph is shown, opening upwards. This is typical for a quadratic function, and since it passes the vertical line test, it is a function. **(d) The graph:** A circle with center at the origin is shown. A vertical line can intersect the circle at more than one point, so it is not a function. **(e) The table:** | x | 1 | 2 | 3 | 2 | 1 | 2 | 3 | 2 | 1 | |---|---|---|---|---|---|---|---|---|---| | y | 10 | 25 | 15 | 25 | 10 | 25 | 15 | 25 | 10 | Here, the \( x \)-values of 2 are repeated with different \( y \)-values, so it is not a function. **(f) The table:** | x | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | |---|---|---|---|---|---|---|---|---|---| | y | 0 | 10 | 10 | 20 | 20 | 30 | 30 | 30 | 30 | Each \( x \)-value has exactly one \( y \)-value, so this is a function.
**2. Determine if each relationship is a function. Justify your conclusion.** **(a) The equation: \( y = 4x \)** This equation represents a linear function. For every input \( x \), there is exactly one output \( y \). **(b) The equation: \( 2x + 3y = 12 \)** This is a linear equation. Solving for \( y \) gives \( y = \frac{12 - 2x}{3} \), which shows that for every \( x \), there is exactly one \( y \). Thus, it is a function. **(c) The graph:** A parabolic graph is shown, opening upwards. This is typical for a quadratic function, and since it passes the vertical line test, it is a function. **(d) The graph:** A circle with center at the origin is shown. A vertical line can intersect the circle at more than one point, so it is not a function. **(e) The table:** | x | 1 | 2 | 3 | 2 | 1 | 2 | 3 | 2 | 1 | |---|---|---|---|---|---|---|---|---|---| | y | 10 | 25 | 15 | 25 | 10 | 25 | 15 | 25 | 10 | Here, the \( x \)-values of 2 are repeated with different \( y \)-values, so it is not a function. **(f) The table:** | x | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | |---|---|---|---|---|---|---|---|---|---| | y | 0 | 10 | 10 | 20 | 20 | 30 | 30 | 30 | 30 | Each \( x \)-value has exactly one \( y \)-value, so this is a function.
Algebra and Trigonometry (6th Edition)
6th Edition
ISBN:9780134463216
Author:Robert F. Blitzer
Publisher:Robert F. Blitzer
ChapterP: Prerequisites: Fundamental Concepts Of Algebra
Section: Chapter Questions
Problem 1MCCP: In Exercises 1-25, simplify the given expression or perform the indicated operation (and simplify,...
Related questions
Question
100%

Transcribed Image Text:**2. Determine if each relationship is a function. Justify your conclusion.**
**(a) The equation: \( y = 4x \)**
This equation represents a linear function. For every input \( x \), there is exactly one output \( y \).
**(b) The equation: \( 2x + 3y = 12 \)**
This is a linear equation. Solving for \( y \) gives \( y = \frac{12 - 2x}{3} \), which shows that for every \( x \), there is exactly one \( y \). Thus, it is a function.
**(c) The graph:**
A parabolic graph is shown, opening upwards. This is typical for a quadratic function, and since it passes the vertical line test, it is a function.
**(d) The graph:**
A circle with center at the origin is shown. A vertical line can intersect the circle at more than one point, so it is not a function.
**(e) The table:**
| x | 1 | 2 | 3 | 2 | 1 | 2 | 3 | 2 | 1 |
|---|---|---|---|---|---|---|---|---|---|
| y | 10 | 25 | 15 | 25 | 10 | 25 | 15 | 25 | 10 |
Here, the \( x \)-values of 2 are repeated with different \( y \)-values, so it is not a function.
**(f) The table:**
| x | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 |
|---|---|---|---|---|---|---|---|---|---|
| y | 0 | 10 | 10 | 20 | 20 | 30 | 30 | 30 | 30 |
Each \( x \)-value has exactly one \( y \)-value, so this is a function.
Expert Solution
Step 1
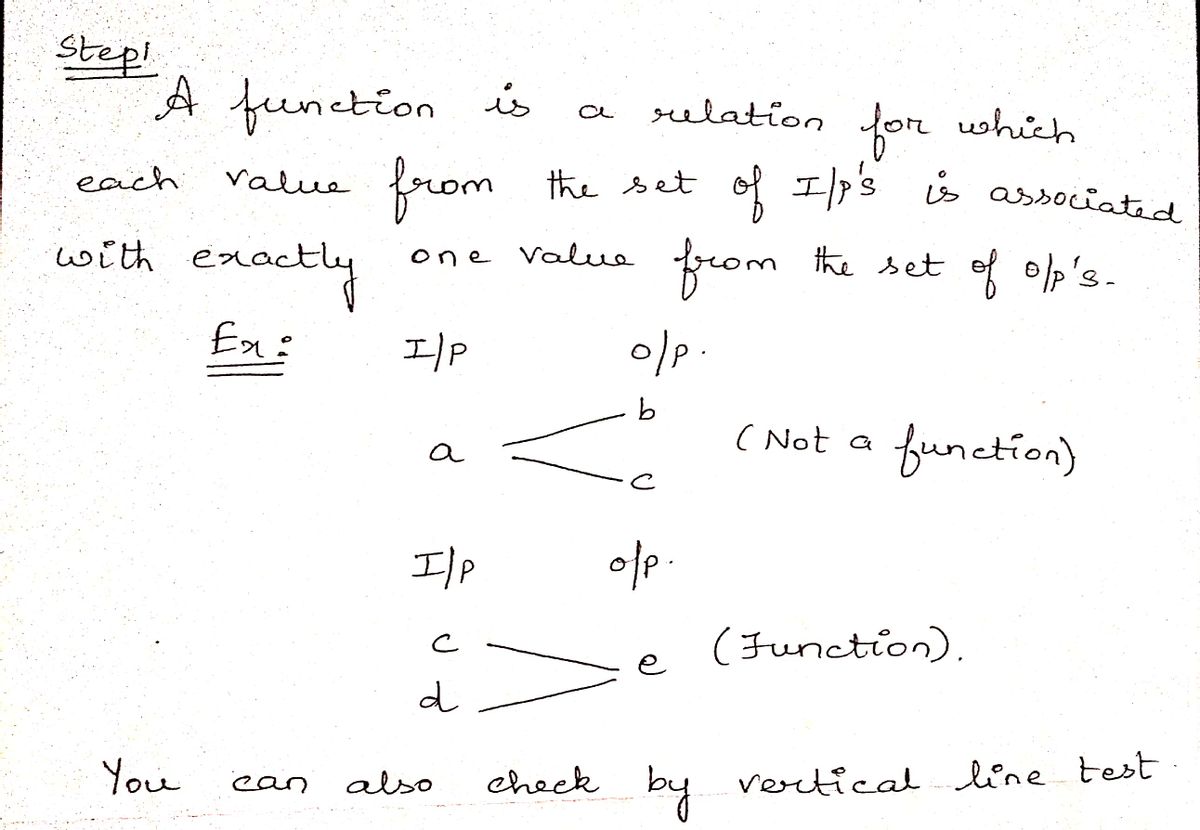
Trending now
This is a popular solution!
Step by step
Solved in 5 steps with 5 images

Recommended textbooks for you

Algebra and Trigonometry (6th Edition)
Algebra
ISBN:
9780134463216
Author:
Robert F. Blitzer
Publisher:
PEARSON

Contemporary Abstract Algebra
Algebra
ISBN:
9781305657960
Author:
Joseph Gallian
Publisher:
Cengage Learning

Linear Algebra: A Modern Introduction
Algebra
ISBN:
9781285463247
Author:
David Poole
Publisher:
Cengage Learning

Algebra and Trigonometry (6th Edition)
Algebra
ISBN:
9780134463216
Author:
Robert F. Blitzer
Publisher:
PEARSON

Contemporary Abstract Algebra
Algebra
ISBN:
9781305657960
Author:
Joseph Gallian
Publisher:
Cengage Learning

Linear Algebra: A Modern Introduction
Algebra
ISBN:
9781285463247
Author:
David Poole
Publisher:
Cengage Learning

Algebra And Trigonometry (11th Edition)
Algebra
ISBN:
9780135163078
Author:
Michael Sullivan
Publisher:
PEARSON

Introduction to Linear Algebra, Fifth Edition
Algebra
ISBN:
9780980232776
Author:
Gilbert Strang
Publisher:
Wellesley-Cambridge Press

College Algebra (Collegiate Math)
Algebra
ISBN:
9780077836344
Author:
Julie Miller, Donna Gerken
Publisher:
McGraw-Hill Education