a) What is the magnitude of the acceleration of each block?b) How much time would I take for the 4.3kg block to fall to the floor? In the system shown below, two blocks are connected by a massless string that passes over ahoop-shaped pulley. The pulley is 11 cm in diameter and has 1.7 kg of mass. As the pulley turns,friction between the pulley and its axle exerts 0.60 Nxm of torque. The blocks are released fromrest.4.3 kg1.5ma.6 kg floor

Answered: a) What is the magnitude of the…

Related questions

Question

a) What is the magnitude of the acceleration of each block?

b) How much time would I take for the 4.3kg block to fall to the floor?

In the system shown below, two blocks are connected by a massless string that passes over a
hoop-shaped pulley. The pulley is 11 cm in diameter and has 1.7 kg of mass. As the pulley turns,
friction between the pulley and its axle exerts 0.60 Nxm of torque. The blocks are released from
rest.
4.3 kg
1.5m
a.6 kg floor

Expert Solution

Step 1

Newton's Second law of motion

According to Newton's Second Law of motion, the rate of change of momentum of an object is directly proportional to the net external force applied to the object. Mathematically it is represented as

$\sum_{i = 1}^{n} F_{i} = m a$

The LHS is the net external force acting on an object of mass $m$ causing an acceleration of $a$

For rotation, the net external torque acting on a rigid body is equal to the moment of inertia times the angular acceleration.

$\sum_{i = 1}^{n} τ_{i} = I α$

Step 2

a) Let the mass of the bigger block be $m$ and the smaller block be $M$ . Let us draw the diagrams showing all the forces.

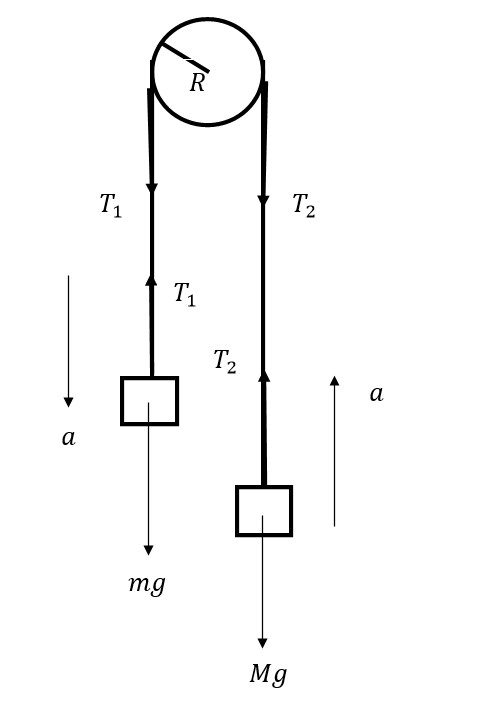
Let us consider the bigger block and the upward as positive. Balancing forces and by Newton's Second law we get

$T_{1} - m g = - m a \Rightarrow T_{1} = m g - m a$

For the smaller block

$T_{2} - M g = M a \Rightarrow T_{2} = M g + M a$

Let us consider the anti-clockwise torque to be positive then the torque due to frictional force will be opposite to the motion of the pulley. Thus the torque due to frictional force will be in the clockwise direction. Therefore by Newton's second law of motion for rotation

$T_{1} R - f R - T_{2} R = I α$

$f R$ is the torque due to friction on the pulley. The moment of inertia of the pulley which is in the shape of a ring about an axis passing through the center and perpendicular to the surface is

$I = M_{P} R^{2}$

For no slipping condition $α = \frac{a}{R}$

This gives

$T_{1} R - f R - T_{2} R = M_{P} R^{2} \times \frac{a}{R} \Rightarrow T_{1} R - f R - T_{2} R = M_{P} a R$

This gives

$(m g - m a) R - f R - (M g + M a) = M_{P} a R$

Let us substitute all the values

$((4.3 \times 9.8) - (4.3 \times a)) \frac{0.11}{2} - 0.6 - ((2.6 \times 9.8) + (2.6 \times a)) \frac{0.11}{2} = 1.7 \times a \times \frac{0.11}{2} \Rightarrow 2.3177 - 0.2365 a - 0.6 - 1.4014 - 0.143 a = 0.0935 a \Rightarrow 0.0935 a + 0.2365 a + 0.143 a = 2.3177 - 0.6 - 1.4014 \Rightarrow 0.473 a = 0.3163 \Rightarrow a = \frac{0.3163}{0.473} = 0.67 m / s^{2}$

b) From the kinematics equation

$s = u t + \frac{1}{2} a t^{2}$

we can find the time for the 4.3 kg body to reach the ground.

Since the displacement is in the downward direction $s = - 1.5 m$

Let the time taken be $t s$ . Thus

$- 1.5 = \frac{1}{2} \times (- 0.67) \times t^{2} \Rightarrow t = \sqrt{\frac{1.5 \times 2}{0.67}} s = 2.12 s$

Trending now

This is a popular solution!

Step by step

Solved in 3 steps with 1 images