A) The area under the curve y 8 2² from x = 1 to a = t is equal to B) The area under the curve from x = 1 to x = 10 is equal to C) The area under the curve from = 1 to x = 100 is equal to D) The area under the curve from x = 1 to x = 1000 is equal to E) The total area under this curve for > 1 is equal to
A) The area under the curve y 8 2² from x = 1 to a = t is equal to B) The area under the curve from x = 1 to x = 10 is equal to C) The area under the curve from = 1 to x = 100 is equal to D) The area under the curve from x = 1 to x = 1000 is equal to E) The total area under this curve for > 1 is equal to
Calculus: Early Transcendentals
8th Edition
ISBN:9781285741550
Author:James Stewart
Publisher:James Stewart
Chapter1: Functions And Models
Section: Chapter Questions
Problem 1RCC: (a) What is a function? What are its domain and range? (b) What is the graph of a function? (c) How...
Related questions
Question
100%
![### Calculating the Area Under the Curve
This section focuses on evaluating the area under the curve of the function \( y = \frac{8}{x^2} \) for various intervals. Use integration techniques to solve these problems.
**A) Evaluate the definite integral of the function \( y = \frac{8}{x^2} \) from \( x = 1 \) to \( x = t \).**
\[ \text{The area is equal to } \boxed{} \]
**B) Calculate the area under the curve from \( x = 1 \) to \( x = 10 \).**
\[ \text{The area is equal to } \boxed{} \]
**C) Find the area under the curve from \( x = 1 \) to \( x = 100 \).**
\[ \text{The area is equal to } \boxed{} \]
**D) Determine the area under the curve from \( x = 1 \) to \( x = 1000 \).**
\[ \text{The area is equal to } \boxed{} \]
**E) Calculate the total area under the curve for \( x \geq 1 \).**
\[ \text{The total area is equal to } \boxed{} \]
### Additional Notes
- Ensure proper application of the definite integral formula for finding the area under the curve.
- Consider the behavior of the function as \( x \) approaches large values and potential implications for convergence to a finite area.](/v2/_next/image?url=https%3A%2F%2Fcontent.bartleby.com%2Fqna-images%2Fquestion%2Fe8fabc15-e5db-480c-94d9-e42b67b656a8%2Fe257340e-810a-4365-a412-779319fd3e36%2F25raxt_processed.jpeg&w=3840&q=75)
Transcribed Image Text:### Calculating the Area Under the Curve
This section focuses on evaluating the area under the curve of the function \( y = \frac{8}{x^2} \) for various intervals. Use integration techniques to solve these problems.
**A) Evaluate the definite integral of the function \( y = \frac{8}{x^2} \) from \( x = 1 \) to \( x = t \).**
\[ \text{The area is equal to } \boxed{} \]
**B) Calculate the area under the curve from \( x = 1 \) to \( x = 10 \).**
\[ \text{The area is equal to } \boxed{} \]
**C) Find the area under the curve from \( x = 1 \) to \( x = 100 \).**
\[ \text{The area is equal to } \boxed{} \]
**D) Determine the area under the curve from \( x = 1 \) to \( x = 1000 \).**
\[ \text{The area is equal to } \boxed{} \]
**E) Calculate the total area under the curve for \( x \geq 1 \).**
\[ \text{The total area is equal to } \boxed{} \]
### Additional Notes
- Ensure proper application of the definite integral formula for finding the area under the curve.
- Consider the behavior of the function as \( x \) approaches large values and potential implications for convergence to a finite area.
Expert Solution
Step 1: Solution of Part A to C
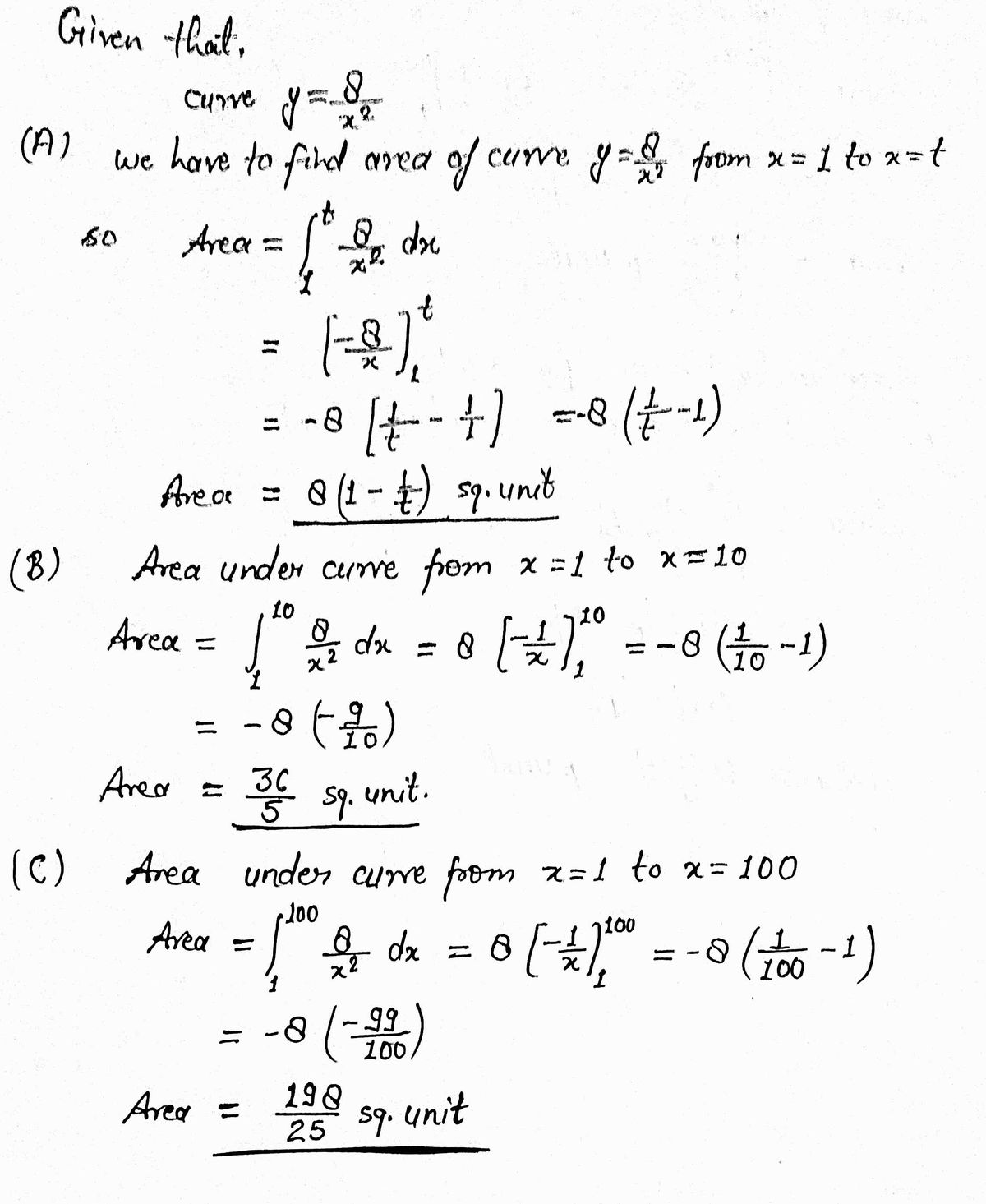
Step by step
Solved in 2 steps with 2 images

Recommended textbooks for you

Calculus: Early Transcendentals
Calculus
ISBN:
9781285741550
Author:
James Stewart
Publisher:
Cengage Learning

Thomas' Calculus (14th Edition)
Calculus
ISBN:
9780134438986
Author:
Joel R. Hass, Christopher E. Heil, Maurice D. Weir
Publisher:
PEARSON

Calculus: Early Transcendentals (3rd Edition)
Calculus
ISBN:
9780134763644
Author:
William L. Briggs, Lyle Cochran, Bernard Gillett, Eric Schulz
Publisher:
PEARSON

Calculus: Early Transcendentals
Calculus
ISBN:
9781285741550
Author:
James Stewart
Publisher:
Cengage Learning

Thomas' Calculus (14th Edition)
Calculus
ISBN:
9780134438986
Author:
Joel R. Hass, Christopher E. Heil, Maurice D. Weir
Publisher:
PEARSON

Calculus: Early Transcendentals (3rd Edition)
Calculus
ISBN:
9780134763644
Author:
William L. Briggs, Lyle Cochran, Bernard Gillett, Eric Schulz
Publisher:
PEARSON

Calculus: Early Transcendentals
Calculus
ISBN:
9781319050740
Author:
Jon Rogawski, Colin Adams, Robert Franzosa
Publisher:
W. H. Freeman


Calculus: Early Transcendental Functions
Calculus
ISBN:
9781337552516
Author:
Ron Larson, Bruce H. Edwards
Publisher:
Cengage Learning