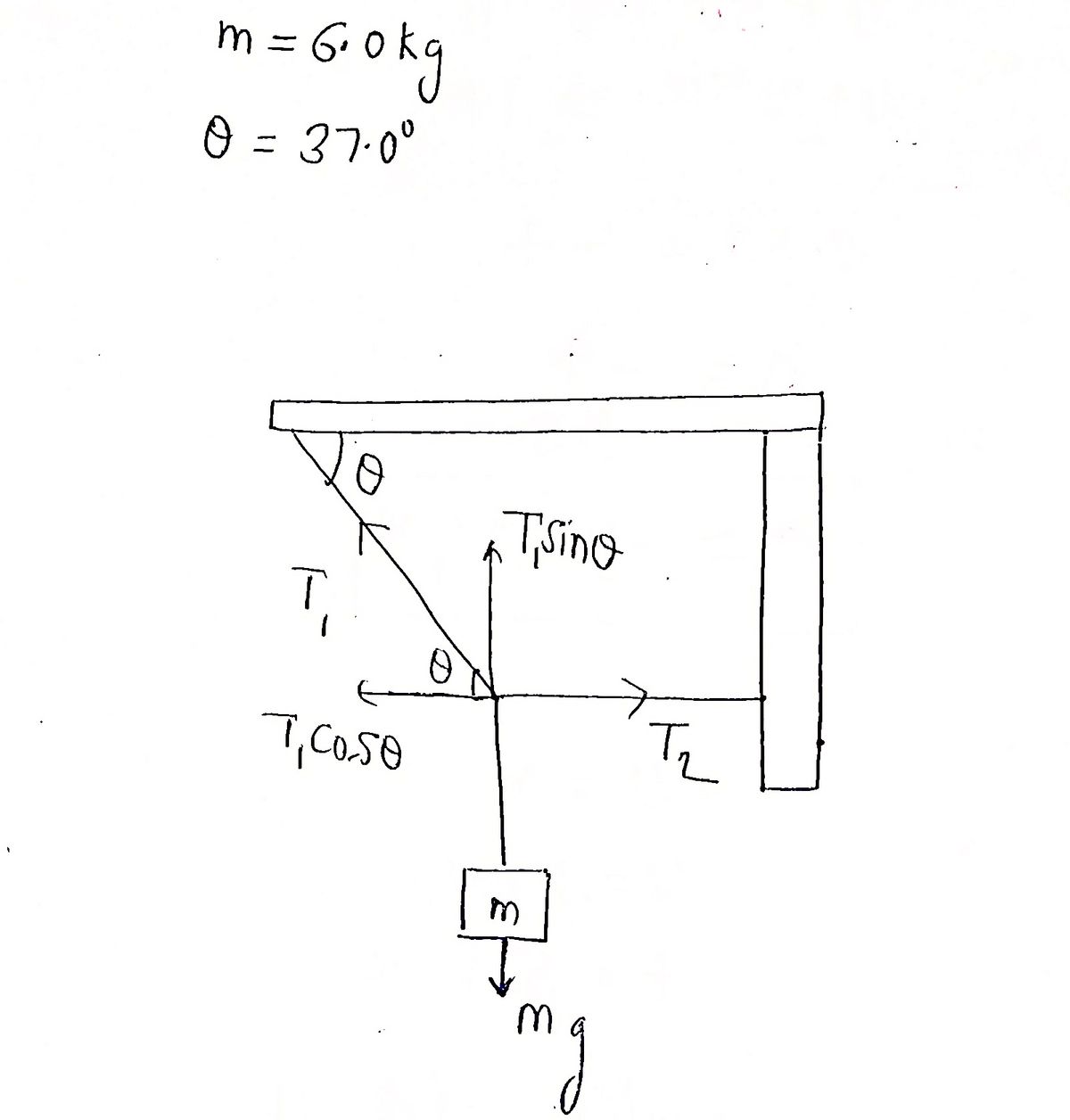
Must sketch free body diagram **Title: Solving Tension in Cables Using Translational Equilibrium****Problem Statement:**A system of cables is used to support a block as shown in Figure 2 below. Find the tension in the two cables \( T_1 \) and \( T_2 \) if the mass of the block \( m = 6.0 \, \text{kg} \) and the angle \( \theta = 37.0^\circ \). Neglect the mass of the cables.*(Hint: Use the conditions of translational equilibrium.)***Diagram Explanation:**In Figure 2, a block of mass \( m \) is suspended by two cables. Cable \( T_1 \) is positioned at an angle \( \theta \) with the horizontal ceiling and exerts tension in the direction of the cable. Cable \( T_2 \) is horizontal, exerting tension directly to the right.**Figure 2:**- The diagram shows a block suspended from a point where two cables meet.- \( T_1 \) is the tension in the left cable forming an angle \( \theta \) with the ceiling.- \( T_2 \) is the tension in the right cable, which is horizontal.- The block with mass \( m \) hangs directly downward from the point where the cables meet.**Concepts Involved:**- Translational equilibrium occurs when the sum of forces in any direction is zero.- The forces involved include gravitational force on the block (its weight) and tensions in the cables.**Steps to Solve:**1. **Resolve Forces:** - Consider the vertical and horizontal components of forces. 2. **Vertical Forces:** - The vertical component of \( T_1 \) (\( T_1 \sin \theta \)) balances the weight of the block (\( mg \)). - Equation: \( T_1 \sin \theta = mg \).3. **Horizontal Forces:** - The horizontal component of \( T_1 \) (\( T_1 \cos \theta \)) balances \( T_2 \). - Equation: \( T_1 \cos \theta = T_2 \).4. **Calculations:** - Use the equations to solve for \( T_1 \) and \( T_2 \).Understanding and applying these principles will allow you to find the tensions in the cables when

Answered: Image /qna-images/answer/f4f44044-60af-4cd6-9216-0ec958d29ecb.jpg

A system of cables is used to support a block as shown in Figure 2 below. Find the tension in the two cables T₁ and T₂ if the mass of the block m = 6.0 kg and the angle 0 = 37.0°. Neglect the mass of the cables. (Hint: Use the conditions of translational equilibrium.) 0 T₁ Figure 2 m T₂

A system of cables is used to support a block as shown in Figure 2 below. Find the tension in the two cables T₁ and T₂ if the mass of the block m = 6.0 kg and the angle 0 = 37.0°. Neglect the mass of the cables. (Hint: Use the conditions of translational equilibrium.) 0 T₁ Figure 2 m T₂

College Physics

11th Edition

ISBN:9781305952300

Author:Raymond A. Serway, Chris Vuille

Publisher:Raymond A. Serway, Chris Vuille

Chapter1: Units, Trigonometry. And Vectors

Section: Chapter Questions

Problem 1CQ: Estimate the order of magnitude of the length, in meters, of each of the following; (a) a mouse, (b)...

See similar textbooks

Similar questions

On an atwood machine why is the max acceleration of the hanging mass 9.8? Explain using net force equations Thanks
Draw a free body diagram for an object sliding down a ramp with friction?
10. A crate is accelerated at a constant rate along a rough horizontal floor. Draw a free-body diagram for the crate and compare all the forces exerted on the crate.
Explain why you have chosen that answer
Please consider the following phases of jumping: A. Being pushed up into the air, but not in contact with the trampoline. B. Flying up into the air above the trampoline. please see a free body diagram for the above cases. The diagram need to have all relevant forces labeled, scalded poportionstelt, and there to be a coordinate axis present. Indicate the net force if it is non-zero.
Please draw the free body diagram clear and also show in the free body diagram if you need to add force
Must have a free body diagram as well
I need a free body diagram
7. Draw a force diagram for a skydiver who is descending at a constant velocity. Label the forces and use equality marks on the force vectors.
A 6.0-kg box rests on a lab bench. A young lady pushes it with a horizontal 50.0-N force. The box slides against an unknown horizontal friction force. The acceleration is 2.0 m/s2 a) Draw a free-body diagram carefully labeling all the forces. (Note that the velocity is not part ofthe free-body diagram, and need not be shown. It helps to show it, only so that the direction ofthe force of friction can be determined correctly.)b) Write out the equation of motion (that is, Newton’s Second Law, Fnet = ma), and solve for thefriction force.c) If the young lady now abruptly stops pushing, in how much time will the box come to rest, if thebox is moving at 2.0 m/s?