A pertsn if contesting for an Engineer pasition in boo Companies, A and B- The chance of his puceess o become Engineer of he of winning A s 70%, wheread the possibility company N The Enginer of company B in os. What is the 0) Hhe will become pabability hat the Engineer of botth comparnie ( He will becomo the Engineer of at leaut one company li He will hot become the Engineer of at least one company Enginear

Obtain the probability that he will become engineer of both the companies.
The probability that he will become engineer of both the companies is obtained below:
Event:
The collection of outcomes in an experiment is called as an event.
Independent Events:
Any two events A and B are said to independent if the occurrence of the event A does not affect the occurrence of another event B.
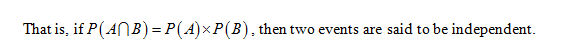
From the information, given that
Let A denote the probability that he will become engineer in company A. That is, P(A)=0.70.
Let B denote the probability that he will become engineer in company B. That is, P(B)=0.50.
Since both the events A and B are independent events.
The required probability is,
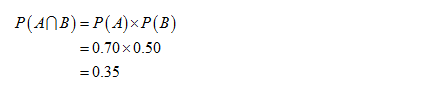
The probability that he will become engineer of both the companies is 0.35.
Obtain the probability that he will become the Engineer of at least one company.
The probability that he will become the Engineer of at least one company is obtained below:
Addition rule:
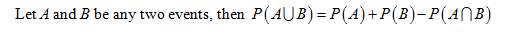
The required probability is,
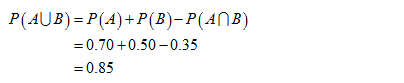
The probability that he will become the Engineer of at least one company is 0.85.
Step by step
Solved in 3 steps with 5 images









