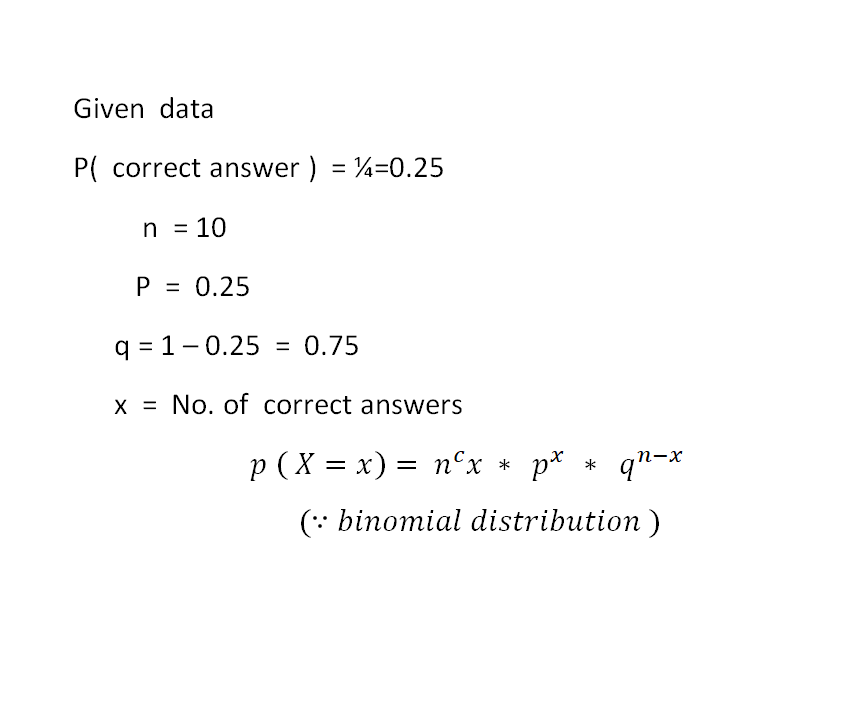
A multiple choice test has 10 questions each of which has 4 possible answers, only one of which is correct, if Judy, who forgot to study for the test, guesses on all questions, what is the probability that she will answer exactly 3 questions correctly? (hint: Binomial Distribution) 0.5006 0.0021 0.0156 0.2816 0.2503
A multiple choice test has 10 questions each of which has 4 possible answers, only one of which is correct, if Judy, who forgot to study for the test, guesses on all questions, what is the probability that she will answer exactly 3 questions correctly? (hint: Binomial Distribution) 0.5006 0.0021 0.0156 0.2816 0.2503
MATLAB: An Introduction with Applications
6th Edition
ISBN:9781119256830
Author:Amos Gilat
Publisher:Amos Gilat
Chapter1: Starting With Matlab
Section: Chapter Questions
Problem 1P
Related questions
Question
8.
![### Educational Content on Probability: Understanding Binomial Distribution
**Problem Scenario:**
A multiple choice test consists of 10 questions, each with 4 possible answers, only one of which is correct. If Judy, who forgot to study for the test, guesses on all questions, we seek to find the probability that she will answer exactly 3 questions correctly. (Hint: Binomial Distribution)
**Answer Choices:**
- 0.5006
- 0.0021
- 0.0156
- 0.2816
- 0.2503
### Explanation of the Concept:
This problem involves calculating probabilities using the **binomial distribution**. The binomial distribution models the number of successful outcomes in a fixed number of independent and identically distributed binary experiments. Here, each question represents a binary experiment with a success (correct answer) happening with a probability of 0.25 (since 1 out of 4 options is correct).
**Steps to Solve:**
1. **Define the variables:**
- Number of trials (n) = 10 (the number of questions)
- Probability of success on a single trial (p) = 0.25
- Number of successes (k) we are interested in = 3
2. **Binomial Probability Formula:**
\[
P(X = k) = \binom{n}{k} p^k (1-p)^{n-k}
\]
where \(\binom{n}{k}\) is the binomial coefficient, calculated as \(\frac{n!}{k!(n-k)!}\).
By plugging in the values, you can calculate the probability of exactly 3 correct answers:
\[
P(X = 3) = \binom{10}{3} (0.25)^3 (0.75)^7
\]
This setup helps in reinforcing the understanding of how to use the binomial distribution in probability scenarios.](/v2/_next/image?url=https%3A%2F%2Fcontent.bartleby.com%2Fqna-images%2Fquestion%2Fe5247ce5-a3e5-4e1f-ba29-c615e03ea9bd%2F24d20306-c204-420a-9569-8b1da23b974e%2F8c4g5pv_processed.png&w=3840&q=75)
Transcribed Image Text:### Educational Content on Probability: Understanding Binomial Distribution
**Problem Scenario:**
A multiple choice test consists of 10 questions, each with 4 possible answers, only one of which is correct. If Judy, who forgot to study for the test, guesses on all questions, we seek to find the probability that she will answer exactly 3 questions correctly. (Hint: Binomial Distribution)
**Answer Choices:**
- 0.5006
- 0.0021
- 0.0156
- 0.2816
- 0.2503
### Explanation of the Concept:
This problem involves calculating probabilities using the **binomial distribution**. The binomial distribution models the number of successful outcomes in a fixed number of independent and identically distributed binary experiments. Here, each question represents a binary experiment with a success (correct answer) happening with a probability of 0.25 (since 1 out of 4 options is correct).
**Steps to Solve:**
1. **Define the variables:**
- Number of trials (n) = 10 (the number of questions)
- Probability of success on a single trial (p) = 0.25
- Number of successes (k) we are interested in = 3
2. **Binomial Probability Formula:**
\[
P(X = k) = \binom{n}{k} p^k (1-p)^{n-k}
\]
where \(\binom{n}{k}\) is the binomial coefficient, calculated as \(\frac{n!}{k!(n-k)!}\).
By plugging in the values, you can calculate the probability of exactly 3 correct answers:
\[
P(X = 3) = \binom{10}{3} (0.25)^3 (0.75)^7
\]
This setup helps in reinforcing the understanding of how to use the binomial distribution in probability scenarios.
Expert Solution
Step 1
Step by step
Solved in 2 steps with 2 images

Recommended textbooks for you

MATLAB: An Introduction with Applications
Statistics
ISBN:
9781119256830
Author:
Amos Gilat
Publisher:
John Wiley & Sons Inc

Probability and Statistics for Engineering and th…
Statistics
ISBN:
9781305251809
Author:
Jay L. Devore
Publisher:
Cengage Learning

Statistics for The Behavioral Sciences (MindTap C…
Statistics
ISBN:
9781305504912
Author:
Frederick J Gravetter, Larry B. Wallnau
Publisher:
Cengage Learning

MATLAB: An Introduction with Applications
Statistics
ISBN:
9781119256830
Author:
Amos Gilat
Publisher:
John Wiley & Sons Inc

Probability and Statistics for Engineering and th…
Statistics
ISBN:
9781305251809
Author:
Jay L. Devore
Publisher:
Cengage Learning

Statistics for The Behavioral Sciences (MindTap C…
Statistics
ISBN:
9781305504912
Author:
Frederick J Gravetter, Larry B. Wallnau
Publisher:
Cengage Learning

Elementary Statistics: Picturing the World (7th E…
Statistics
ISBN:
9780134683416
Author:
Ron Larson, Betsy Farber
Publisher:
PEARSON

The Basic Practice of Statistics
Statistics
ISBN:
9781319042578
Author:
David S. Moore, William I. Notz, Michael A. Fligner
Publisher:
W. H. Freeman

Introduction to the Practice of Statistics
Statistics
ISBN:
9781319013387
Author:
David S. Moore, George P. McCabe, Bruce A. Craig
Publisher:
W. H. Freeman