A multiple-choice exam consists of 10 questions, each having 5 possible answers. To pass, you must answer at least 6 out of 10 questions correctly. What is the chance of this, if: You go into the exam without knowing a thing, and have to resort to pure guessing? You have studied enough so that on each question, 3 choices can be eliminated. But then you have to make a pure guess between the remaining 2 choices. You have studied enough so that you know for sure the correct answer on 3 questions. For the remaining 6 questions you have to resort to pure guessing
A multiple-choice exam consists of 10 questions, each having 5 possible answers. To pass, you must answer at least 6 out of 10 questions correctly. What is the chance of this, if: You go into the exam without knowing a thing, and have to resort to pure guessing? You have studied enough so that on each question, 3 choices can be eliminated. But then you have to make a pure guess between the remaining 2 choices. You have studied enough so that you know for sure the correct answer on 3 questions. For the remaining 6 questions you have to resort to pure guessing
A First Course in Probability (10th Edition)
10th Edition
ISBN:9780134753119
Author:Sheldon Ross
Publisher:Sheldon Ross
Chapter1: Combinatorial Analysis
Section: Chapter Questions
Problem 1.1P: a. How many different 7-place license plates are possible if the first 2 places are for letters and...
Related questions
Question
A multiple-choice exam consists of 10 questions, each having 5 possible answers. To pass, you must answer at least 6 out of 10 questions correctly. What is the chance of this, if:
- You go into the exam without knowing a thing, and have to resort to pure guessing?
- You have studied enough so that on each question, 3 choices can be eliminated. But then you have to make a pure guess between the remaining 2 choices.
- You have studied enough so that you know for sure the correct answer on 3 questions. For the remaining 6 questions you have to resort to pure guessing.
Expert Solution
Step 1
Here, n = 10 and p = .
1. The probability is,
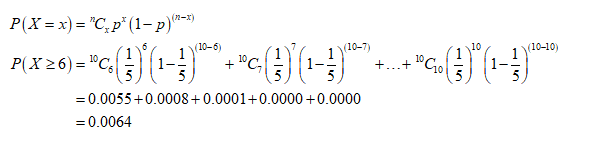
The probability is 0.0064.
Step by step
Solved in 3 steps with 3 images

Recommended textbooks for you

A First Course in Probability (10th Edition)
Probability
ISBN:
9780134753119
Author:
Sheldon Ross
Publisher:
PEARSON


A First Course in Probability (10th Edition)
Probability
ISBN:
9780134753119
Author:
Sheldon Ross
Publisher:
PEARSON
