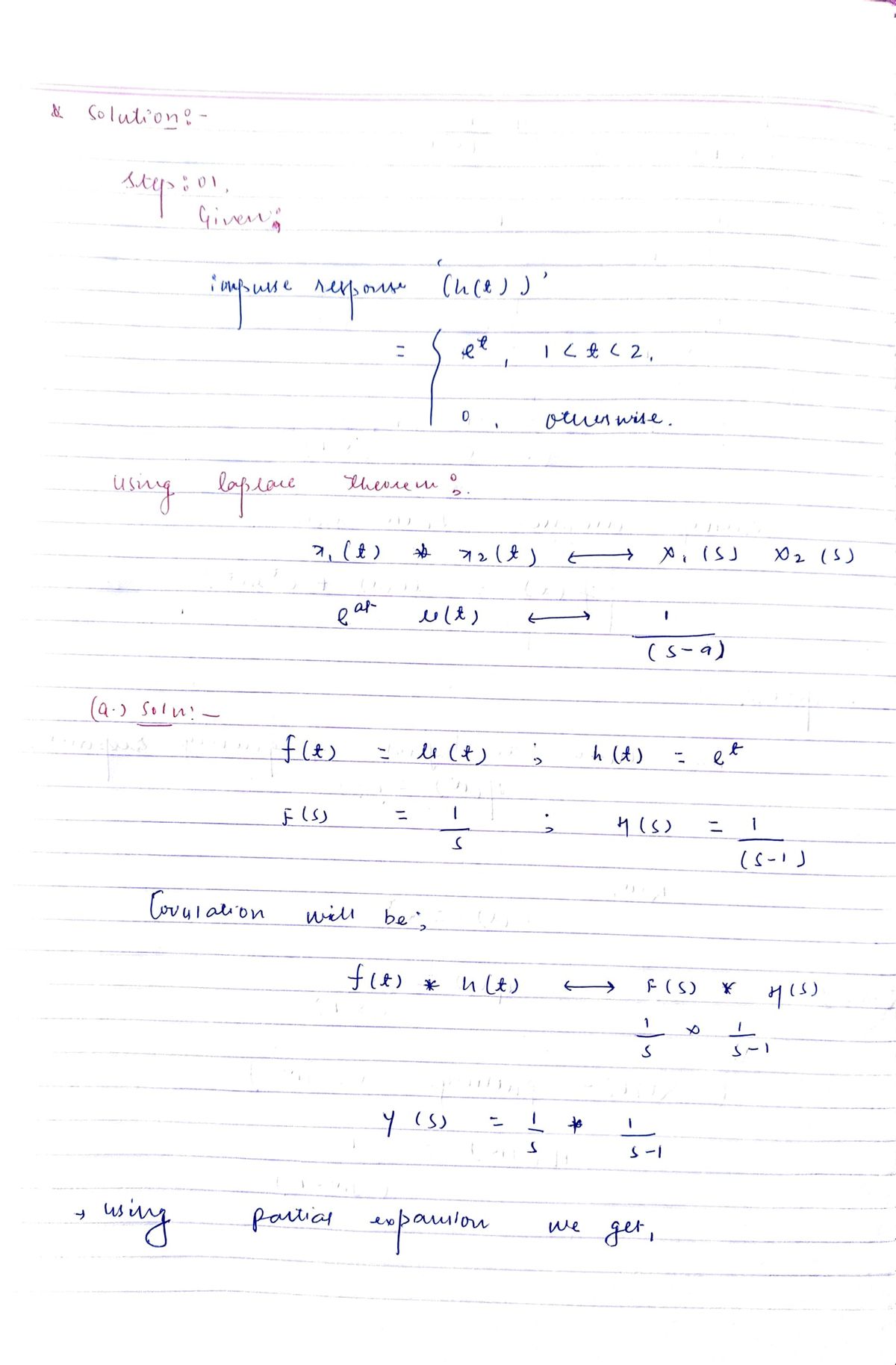
A continuous-time LTI system has impulse response et, -{ h(t) = 1
Advanced Engineering Mathematics
10th Edition
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Erwin Kreyszig
Chapter2: Second-order Linear Odes
Section: Chapter Questions
Problem 1RQ
Related questions
Question
Need help with answering this question, please write clearly, thanks.

Transcribed Image Text:5.
A continuous-time LTI system has impulse response
1 < t < 2,
0, otherwise.
h(t) =
(a) Using convolution in the time domain, find the response to the input f(t) = u(t).
(b) Find the frequency response H(w).
(c) Using the frequency response, find the response to the input f(t) = 3 cos (t+). To
do this, follow these steps:
• begin by using Euler's rule to express f(t) as the sum of two complex exponential
signals
• using the frequency response expression you derived in part (b), determine the
system's response to each of these two complex exponentials
use Euler's rule to re-combine the outputs into one real-valued cosine expression
(d) Now, we can take advantage of a shortcut to solve problems like part (c). In class,
we discussed the following useful fact: for an LTI system with a real-valued impulsc
response h(t), if the input f(t) = A cos(wot + p) for some amplitude A, frequency wo,
and phase o, then the output will be y(t) = A|H(wo)| cos(wot + + 2H(wo)). Use this
fact to check your answer from part (c), showing your work.
Expert Solution
Step 1
Trending now
This is a popular solution!
Step by step
Solved in 4 steps with 4 images

Recommended textbooks for you

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Mathematics For Machine Technology
Advanced Math
ISBN:
9781337798310
Author:
Peterson, John.
Publisher:
Cengage Learning,

