A circuit made up of 6 resistors is shown in the figure, with resistances R1 = 11 N, R2 = 65 Q, R3 = 38 Q, R4 = 69 N, R5 = 85 Q, and R6= 45 Q. The total current going through the circuit is I= 18 A. R, R4. a R3 R R, Re b Calculate the value of AV, in volts.
A circuit made up of 6 resistors is shown in the figure, with resistances R1 = 11 N, R2 = 65 Q, R3 = 38 Q, R4 = 69 N, R5 = 85 Q, and R6= 45 Q. The total current going through the circuit is I= 18 A. R, R4. a R3 R R, Re b Calculate the value of AV, in volts.
Related questions
Question

Transcribed Image Text:A circuit made up of 6 resistors is shown in the figure, with resistances \( R_1 = 11 \, \Omega \), \( R_2 = 65 \, \Omega \), \( R_3 = 38 \, \Omega \), \( R_4 = 69 \, \Omega \), \( R_5 = 85 \, \Omega \), and \( R_6 = 45 \, \Omega \). The total current going through the circuit is \( I = 18 \, A \).
**Diagram Explanation:**
- The circuit consists of a combination of series and parallel resistors.
- Resistors \( R_1 \) and \( R_2 \) are in series, connected between points \( a \) and \( b \).
- Resistors \( R_3 \), \( R_4 \), \( R_5 \), and \( R_6 \) form a bridge or ladder-like pattern between the series segments.
- \( R_3 \) and \( R_6 \) are in parallel, and are then in series with the combination of \( R_4 \) and \( R_5 \), which are also in parallel.
Calculate the value of \( \Delta V \), in volts.
Expert Solution
Step 1 (equivalent resistance)
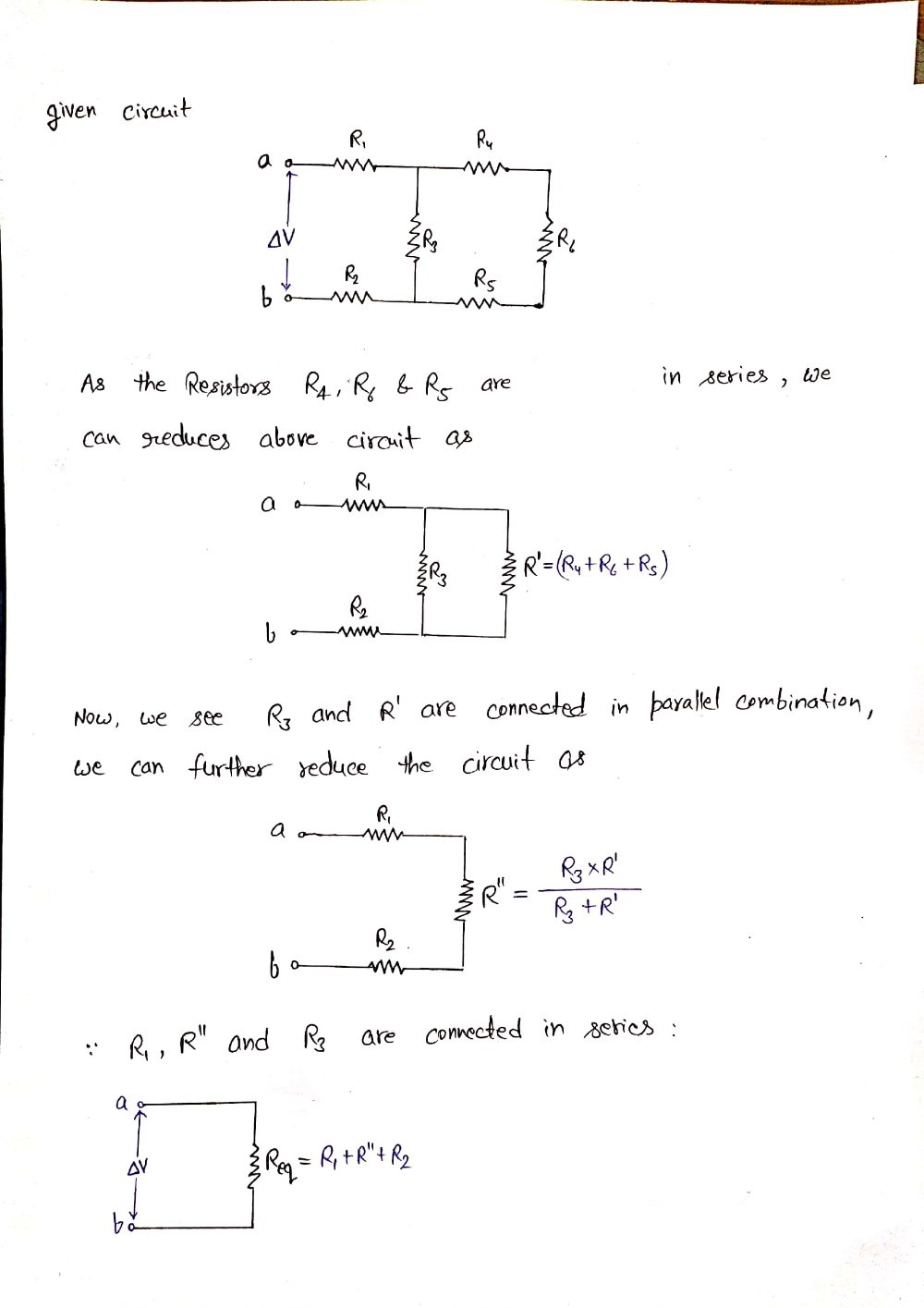 This problem is from Network analysis (Electronics). Let's solve this problem by solving series and parallel combination of resistors to equivalent resistance and Ohm's law. Please have a good look.
This problem is from Network analysis (Electronics). Let's solve this problem by solving series and parallel combination of resistors to equivalent resistance and Ohm's law. Please have a good look.
Step by step
Solved in 2 steps with 2 images
