7 1 m. b =- mm. 5 Calculate the length of a given that the two triangles are similar and d = m, e = 16 a d b e Enter the exact answer. a = mm
7 1 m. b =- mm. 5 Calculate the length of a given that the two triangles are similar and d = m, e = 16 a d b e Enter the exact answer. a = mm
Trigonometry (11th Edition)
11th Edition
ISBN:9780134217437
Author:Margaret L. Lial, John Hornsby, David I. Schneider, Callie Daniels
Publisher:Margaret L. Lial, John Hornsby, David I. Schneider, Callie Daniels
Chapter1: Trigonometric Functions
Section: Chapter Questions
Problem 1RE:
1. Give the measures of the complement and the supplement of an angle measuring 35°.
Related questions
Question
100%
![**Topic: Solving for Unknown Sides in Similar Triangles**
Calculate the length of \( a \) given that the two triangles are similar and \( d = \frac{7}{16} \) m, \( e = \frac{1}{4} \) m, \( b = \frac{5}{4} \) mm.
### Diagram Explanation
The image presents two similar triangles. Each triangle shows its corresponding sides:
- **Large triangle:**
- Side \( a \)
- Side \( b \)
- Side \( c \)
- **Small triangle:**
- Side \( d \)
- Side \( e \)
- Side \( f \)
### Task
You are required to find the length of side \( a \) of the larger triangle.
### Instructions
Enter the exact answer in the provided box.
**Calculation:**
\[ a = \_\_\_\_ \] mm
Utilize the properties of similar triangles and the given side length ratios to calculate the unknown side. Consider the proportion:
\[ \frac{a}{d} = \frac{b}{e} \]
Given:
- Side \( b \) of the large triangle is 5/4 mm
- Side \( e \) of the small triangle is 1/4 m (convert to the same unit if necessary)](/v2/_next/image?url=https%3A%2F%2Fcontent.bartleby.com%2Fqna-images%2Fquestion%2Fbb8f64bb-a739-44c8-9c71-41ea58cbca19%2F7e2f6cac-0274-4249-a496-c2dbfdab5f40%2F0oupyga_processed.png&w=3840&q=75)
Transcribed Image Text:**Topic: Solving for Unknown Sides in Similar Triangles**
Calculate the length of \( a \) given that the two triangles are similar and \( d = \frac{7}{16} \) m, \( e = \frac{1}{4} \) m, \( b = \frac{5}{4} \) mm.
### Diagram Explanation
The image presents two similar triangles. Each triangle shows its corresponding sides:
- **Large triangle:**
- Side \( a \)
- Side \( b \)
- Side \( c \)
- **Small triangle:**
- Side \( d \)
- Side \( e \)
- Side \( f \)
### Task
You are required to find the length of side \( a \) of the larger triangle.
### Instructions
Enter the exact answer in the provided box.
**Calculation:**
\[ a = \_\_\_\_ \] mm
Utilize the properties of similar triangles and the given side length ratios to calculate the unknown side. Consider the proportion:
\[ \frac{a}{d} = \frac{b}{e} \]
Given:
- Side \( b \) of the large triangle is 5/4 mm
- Side \( e \) of the small triangle is 1/4 m (convert to the same unit if necessary)
Expert Solution
Step 1
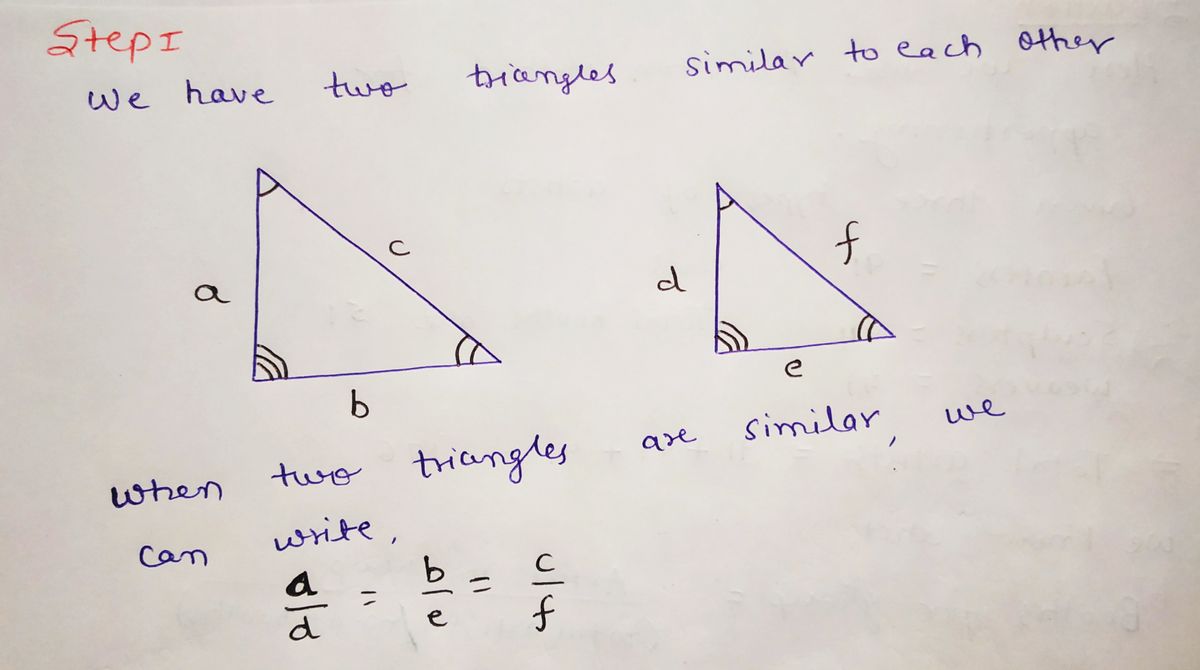
Step by step
Solved in 2 steps with 2 images

Recommended textbooks for you

Trigonometry (11th Edition)
Trigonometry
ISBN:
9780134217437
Author:
Margaret L. Lial, John Hornsby, David I. Schneider, Callie Daniels
Publisher:
PEARSON

Trigonometry (MindTap Course List)
Trigonometry
ISBN:
9781305652224
Author:
Charles P. McKeague, Mark D. Turner
Publisher:
Cengage Learning


Trigonometry (11th Edition)
Trigonometry
ISBN:
9780134217437
Author:
Margaret L. Lial, John Hornsby, David I. Schneider, Callie Daniels
Publisher:
PEARSON

Trigonometry (MindTap Course List)
Trigonometry
ISBN:
9781305652224
Author:
Charles P. McKeague, Mark D. Turner
Publisher:
Cengage Learning


Trigonometry (MindTap Course List)
Trigonometry
ISBN:
9781337278461
Author:
Ron Larson
Publisher:
Cengage Learning