-6 -4 X = -2 y decreasing 6 4 -2 -4 -6 2 4 (i) Identify the critical numbers of f. (Enter your answers as a comma-separated list.) 6 relative maximum X = X (ii) Identify the open interval(s) on which f is increasing or decreasing. (Enter your answer usin notation.) increasing (iii) Determine whether f has a relative maximum, a relative minimum, or neither at each critic (Enter your answers as a comma-separated list.) relative minimum X =
-6 -4 X = -2 y decreasing 6 4 -2 -4 -6 2 4 (i) Identify the critical numbers of f. (Enter your answers as a comma-separated list.) 6 relative maximum X = X (ii) Identify the open interval(s) on which f is increasing or decreasing. (Enter your answer usin notation.) increasing (iii) Determine whether f has a relative maximum, a relative minimum, or neither at each critic (Enter your answers as a comma-separated list.) relative minimum X =
Advanced Engineering Mathematics
10th Edition
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Erwin Kreyszig
Chapter2: Second-order Linear Odes
Section: Chapter Questions
Problem 1RQ
Related questions
Question
![### Graph Analysis: Derivative and Critical Points
The graph displayed represents the derivative \( f' \) of a function \( f \) over the interval \([-6, 6]\) on the x-axis and [-6, 6] on the y-axis.
#### Key Features of the Graph:
- The graph crosses the x-axis at approximately \( x = -4 \), \( x = -1 \), and \( x = 3 \).
- The function \( f' \) changes from positive to negative at \( x = -4 \) and \( x = 3 \), suggesting these are critical points where local maxima or minima of \( f \) might occur.
- The graph \( f' \) transitions from negative to positive at \( x = -1 \), suggesting a critical point for a local minimum of \( f \).
### Questions:
(i) **Identify the critical numbers of \( f \).**
_Enter your answers as a comma-separated list._
\[ x = \_\_\_\_ \]
(ii) **Identify the open interval(s) on which \( f \) is increasing or decreasing.**
_Enter your answer using interval notation._
- **Increasing:** \(\_\_\_\_\)
- **Decreasing:** \(\_\_\_\_\)
(iii) **Determine whether \( f \) has a relative maximum, a relative minimum, or neither at each critical point.**
_Enter your answers as a comma-separated list._
- **Relative minimum:** \( x = \_\_\_\_ \)
- **Relative maximum:** \( x = \_\_\_\_ \)
This exercise helps in understanding the relationship between the derivative of a function and its critical points, intervals of increase and decrease, and identifying local maxima and minima.](/v2/_next/image?url=https%3A%2F%2Fcontent.bartleby.com%2Fqna-images%2Fquestion%2F466923f0-6f55-4935-bc1a-e5c184127f99%2Fcffbc459-2371-44e6-b000-5785cfb985ab%2F3wvnje_processed.jpeg&w=3840&q=75)
Transcribed Image Text:### Graph Analysis: Derivative and Critical Points
The graph displayed represents the derivative \( f' \) of a function \( f \) over the interval \([-6, 6]\) on the x-axis and [-6, 6] on the y-axis.
#### Key Features of the Graph:
- The graph crosses the x-axis at approximately \( x = -4 \), \( x = -1 \), and \( x = 3 \).
- The function \( f' \) changes from positive to negative at \( x = -4 \) and \( x = 3 \), suggesting these are critical points where local maxima or minima of \( f \) might occur.
- The graph \( f' \) transitions from negative to positive at \( x = -1 \), suggesting a critical point for a local minimum of \( f \).
### Questions:
(i) **Identify the critical numbers of \( f \).**
_Enter your answers as a comma-separated list._
\[ x = \_\_\_\_ \]
(ii) **Identify the open interval(s) on which \( f \) is increasing or decreasing.**
_Enter your answer using interval notation._
- **Increasing:** \(\_\_\_\_\)
- **Decreasing:** \(\_\_\_\_\)
(iii) **Determine whether \( f \) has a relative maximum, a relative minimum, or neither at each critical point.**
_Enter your answers as a comma-separated list._
- **Relative minimum:** \( x = \_\_\_\_ \)
- **Relative maximum:** \( x = \_\_\_\_ \)
This exercise helps in understanding the relationship between the derivative of a function and its critical points, intervals of increase and decrease, and identifying local maxima and minima.
Expert Solution
Step 1: Finding critical points
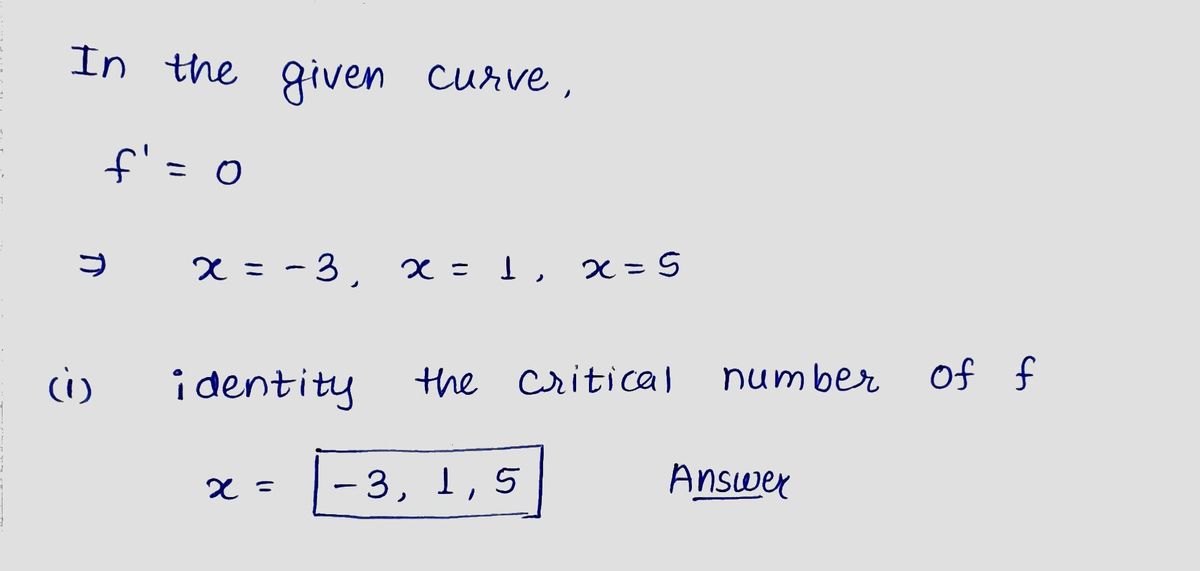
Step by step
Solved in 3 steps with 3 images

Recommended textbooks for you

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Mathematics For Machine Technology
Advanced Math
ISBN:
9781337798310
Author:
Peterson, John.
Publisher:
Cengage Learning,

