Homework Help is Here – Start Your Trial Now!
Math
Advanced Math
5. Determine whether the given statement is true or false. (3} E{{3}, (6}} Choose the correct answer below. O True O False

5. Determine whether the given statement is true or false. (3} E{{3}, (6}} Choose the correct answer below. O True O False

Advanced Engineering Mathematics
Advanced Engineering Mathematics
10th Edition
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Erwin Kreyszig
Chapter2: Second-order Linear Odes
Section: Chapter Questions
Problem 1RQ
See similar textbooks
icon
Related questions
Question
**Problem Statement:** 5. Determine whether the given statement is true or false. \[{3} \in \{{3}, {6}\}\] Choose the correct answer below: - ☐ True - ☐ False --- **Explanation:** The symbol \(\in\) signifies membership, meaning "is an element of." The statement is asking if the set \(\{3\}\) is an element of the set \(\{\{3\}, \{6\}\}\). - The set on the right side, \(\{\{3\}, \{6\}\}\), contains two elements: \({3}\) and \({6}\), both of which are sets. - The set on the left side is \(\{3\}\). Since \(\{3\}\) is indeed one of the elements within the set \(\{\{3\}, \{6\}\}\), the statement is true. --- Note: This exercise helps understand the concept of set membership and how sets can themselves be elements of other sets.
Transcribed Image Text:**Problem Statement:** 5. Determine whether the given statement is true or false. \[{3} \in \{{3}, {6}\}\] Choose the correct answer below: - ☐ True - ☐ False --- **Explanation:** The symbol \(\in\) signifies membership, meaning "is an element of." The statement is asking if the set \(\{3\}\) is an element of the set \(\{\{3\}, \{6\}\}\). - The set on the right side, \(\{\{3\}, \{6\}\}\), contains two elements: \({3}\) and \({6}\), both of which are sets. - The set on the left side is \(\{3\}\). Since \(\{3\}\) is indeed one of the elements within the set \(\{\{3\}, \{6\}\}\), the statement is true. --- Note: This exercise helps understand the concept of set membership and how sets can themselves be elements of other sets.
Expert Solution
Step 1

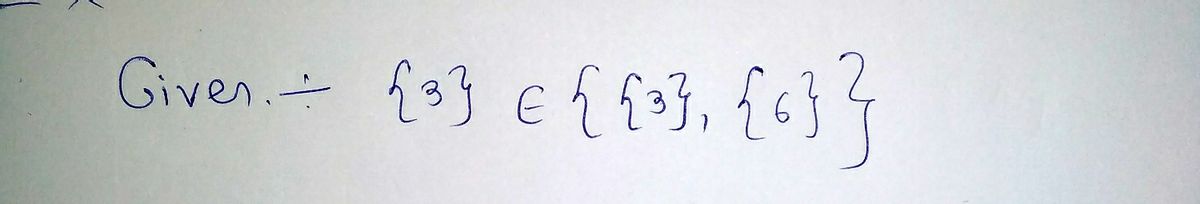
Advanced Math homework question answer, step 1, image 1

steps

Step by step

Solved in 2 steps with 2 images

SEE SOLUTIONCheck out a sample Q&A here
Blurred answer
Similar questions
Recommended textbooks for you
Advanced Engineering Mathematics
Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated
Numerical Methods for Engineers
Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education
Introductory Mathematics for Engineering Applicat…
Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY
Mathematics For Machine Technology
Mathematics For Machine Technology
Advanced Math
ISBN:
9781337798310
Author:
Peterson, John.
Publisher:
Cengage Learning,
Basic Technical Mathematics
Basic Technical Mathematics
Advanced Math
ISBN:
9780134437705
Author:
Washington
Publisher:
PEARSON
Topology
Topology
Advanced Math
ISBN:
9780134689517
Author:
Munkres, James R.
Publisher:
Pearson,
SEE MORE TEXTBOOKS