4. Let f(x) = xe¯* if x > 0 and f(x) = 0 if x < 0. (a) Verify that f is a probability density function. (b) Find P(1 < X < 2). %3D 6. Let f(x) = kx²(1 – x) if 0 < x < 1 and f(x) = 0 if x <0 or x > 1. (a) For what value of k is ƒ a probability density function? (b) For that value of k, find P(X >;). (c) Find the mean.
4. Let f(x) = xe¯* if x > 0 and f(x) = 0 if x < 0. (a) Verify that f is a probability density function. (b) Find P(1 < X < 2). %3D 6. Let f(x) = kx²(1 – x) if 0 < x < 1 and f(x) = 0 if x <0 or x > 1. (a) For what value of k is ƒ a probability density function? (b) For that value of k, find P(X >;). (c) Find the mean.
Advanced Engineering Mathematics
10th Edition
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Erwin Kreyszig
Chapter2: Second-order Linear Odes
Section: Chapter Questions
Problem 1RQ
Related questions
Question
I'm having trouble with these problems, can you help me please?

Transcribed Image Text:9-10 Sketch a direction field for the differential equation. Then use 10. y' = x – y + 1
it to sketch three solution curves.
2. Verify that y= -t cos t – t is a solution of the initial-value
problem
dy
t
= y + t²sin t
dt
y(7) = 0
4. (a) For what values of k does the function y = cos kt satisfy
the differential equation 4y" = –25y?
(b) For those values of k, verify that every member of the
family of functions y = A sin kt + B cos kt is also a
7.1:4
solution.

Transcribed Image Text:4. Let f(x) = xe¯* if x > 0 and f(x) = 0 if x < 0.
(a) Verify that f is a probability density function.
(b) Find P(1 < X < 2).
%3|
6.8: 4
6. Let f(x) = kx²(1 – x) if 0 < x < 1 and f(x) = 0 if x < 0
%3D
or x > 1.
(a) For what value of k is f a probability density function?
(b) For that value of k, find P(X > ;).
(c) Find the mean.
6.8:6
Expert Solution
Step 1
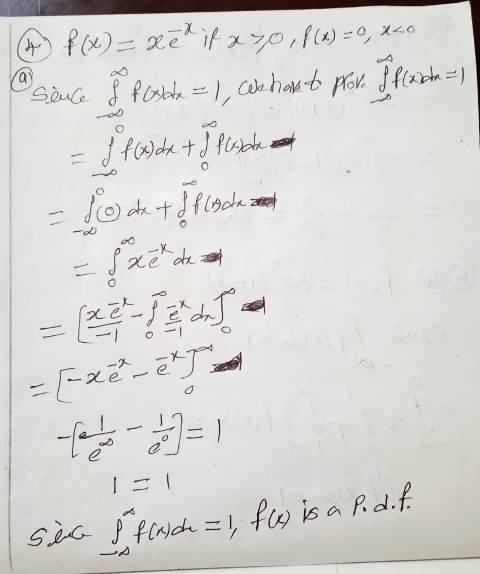
Trending now
This is a popular solution!
Step by step
Solved in 3 steps with 3 images

Recommended textbooks for you

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Mathematics For Machine Technology
Advanced Math
ISBN:
9781337798310
Author:
Peterson, John.
Publisher:
Cengage Learning,

