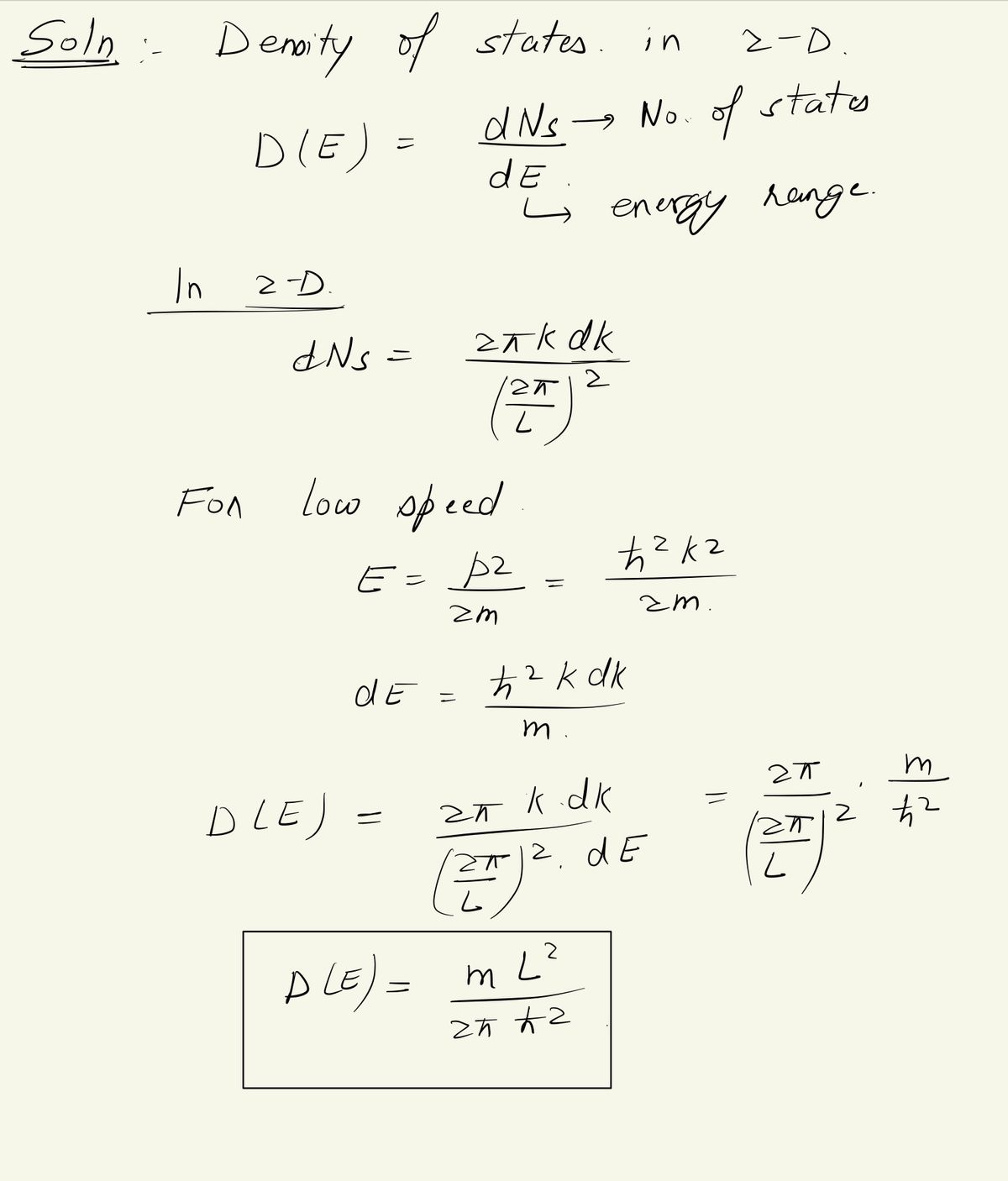
3. For free particles in two dimensions, what is density of states (DOS) in low speed limit (=p2/2m), and in high speed limit (=pc)?
Q: 1. A particle is in an infinite square well of width L. Calculate the ground-state energy if (a) the…
A:
Q: 3. Use the WKB approximation to find the energy level of a particle moving in the potential: V(x) =…
A: Solution by image is shown belowExplanation:Step 1: Step 2: Step 3: Step 4:
Q: 1. Consider a model thermodynamic assembly in which the allowed one-particle states have energies 0,…
A:
Q: The probability of finding a particle moves in the left quarter of a one-dimensional box of length a…
A:
Q: Jhe probability.distribution for bromine gas is shown in the diagram below. Determine the…
A:
Q: A beam of protons with an energy of 5ev Hits a potential energy barrier that is 6ev high and is…
A:
Q: Imagine a certain kind of particle, such that each single-particle state can be occupied by at most…
A: Red
Q: In a system with many particles, the partition function is - higher at higher temperatures. - higher…
A: The partition function is directly proportional to Z∝e-∆EkT -With increase in temperature the…
Q: A finite potential well has a potential of 0 between æ = 0 and æ = L. For an electron, the…
A: Let us try to find what must be the probability to the left side of the well by the given amount of…
Q: 5. Consider two non-interacting particles, each of mass m, in the infinite square wel one is in the…
A:
Q: If I have three fermions in states v, y', and " (a) Construct a fully asymmetric state V (F1, 72,…
A:
Q: A particle confined to move on a sphere is in the state ) = N(2|31) - i|1, -1) + |30) - 3|21)) (a)…
A:
Q: 1. Derive the density of states as a function of energy for a purely two-dimensional electron…
A:
Q: Show that the radial probability density of the Is level has its maximum value at r = ao.
A: Radial probability density function maximization involves knowledge of calculus and the expression…
Q: Using the variational method approximation, find the ground state energy of a particle in a box in…
A:
Q: Show that the probability density for the ground-state solution of the one-dimensional Coulomb…
A: The probability of finding a wave packet in a particular region of phase space is known as a…
Q: The walls of a box for a particle in a box scenario are: a. very small and very soft. b. very small…
A: Particle in a box This model describes that the particle is free to move inside a small region…
Q: In an experiment done by scattering 7.7-MeV a particles from a thin gold foil, experimenters find…
A:
Q: 2. Two particles, one of spin 2 and another of spin ½2, are at rest in a box. The spin state of the…
A: in this question we use clebcsh Gordon equation.
Q: A particle is confined to a one-dimensional harmonic oscillator having infinitely high walls and is…
A: Quantum mechanics started with Plank's work on blackbody radiation. In his work, he emphasized that…
Q: Which of the following is/are state function / functions? 1. q + w 2. q 3. w 4. H -TS…
A: State functions is term used in thermodynamics. State function are called those variables whose…
Q: 3. For Blasius solution of laminar boundary layer, prove for the momentum thickness. U T/x = 1²0 2vx…
A: Given data: θU2νx=∫0∞f'd(1-f') dη=f"(0)=0.4696
Q: To excite an electron in a one-dimensional box from its first excited state to its third excited…
A: Energy required, ∆E=17eV=17×1.6×10-19J
Q: Q11/ what is the physical meaning of: N(v)dv (a) : The average number of molecules that have…
A:
Q: For a spin 1/2 paramagnet with localised moments in the low-field, high-temperature limit the…
A: **As per our company guidelines we are supposed to solve only one question (if there are multiple…
Q: Consider the finite potential well IX xwa - E-lev E=0eV a. Can the measured value of a particle's…
A: (a) No the measured value of a particle's energy in the well can not be zero as the minimum energy…
Q: 1. Consider the Schrödinger equation for a particle in the presence of the potential of the form…
A: Since we answer up to one question, we will answer the first question only. Please resubmit the…
Q: 2. Calculate the transmission probability T for proton ('H; mass 1.007825 amu) and deuterium (2H;…
A:
Q: 1. A model of interest for quantum mechanics, due to its simplicity, corresponds to a delta wall. A…
A: Given, Potential is Vx=δx width of the well is L and height o the well is SL And Schrodinger's…
Q: A system has N weakly interacting identical particles. Each particle has 2 energy levels that are 0…
A: Hello. Since your question has multiple sub-parts, we will solve the first three sub-parts for you.…
Q: A system has three energy levels 0, & and 2 and consists of three particles. Explain the…
A: (I) Maxwell Boltzmann statistics The particles that follow Maxwell Boltzmann statistics are…
Q: 3. A system is in contact with a heat and particle reservoir. Calculate the proba- bility of finding…
A: The probability of finding the system in energy E is p=e-βEZ Where Z is the partition function ∝…
Q: The for dimensional oscillator is wave one v(x) = Ae-a* 72, for the ground state with energy %3D ho…
A: Given: ψx=Ae-α2x2/2 Ground state energy E0=hω2. α2=mωh Perturbation Vt=E0αx104. Since ψx is not…
Q: 3. In a particular semiconductor device, an oxide layer forms a barrier 0.6 nm wide and 9V high…
A:
Q: 4. Refer to the following one-dimensional quantum potential structure. In region 0 the potential V=…
A: Hello. Since your question has multiple sub-parts, we will solve first three sub-parts for you. If…
Q: 3. A particle of mass moves in one dimension in a potential given by v(s)-c8(s). where 8() is the…
A: Given that, A particle is in a one-dimensional box The mass of the particle is m And the potential…
Q: 7. Write out the partition function for a three-dimensional har- monic oscillator assuming that it…
A:
Step by step
Solved in 2 steps with 2 images
