How do I work with the radicals? ### Transcription of Math Problem Solving1. **Expression for $ h(x) $:** \[ h(x) = \sqrt{2 - x} - 2 \]2. **Simplification Steps:** \[ 2 \cdot \frac{1}{2} - \frac{x}{2} \]3. **Derivative Approximation:** \[ \frac{f(x+h) - f(x)}{h} \]4. **Simplifying the Expression:** \[ \frac{\sqrt{2-(x+h)} - \sqrt{2-x}}{h} \]5. **Rewriting the Expression:** \[ \frac{\sqrt{2-(x+h)} - \sqrt{2-x}}{h} = \frac{\left( \sqrt{2-(x+h)} - \sqrt{2-x} \right) \left( \sqrt{2-(x+h)} + \sqrt{2-x} \right)}{\sqrt{2-(x+h)} + \sqrt{2-x}} \]6. **Solving the Difference of Squares:** \[ 2 - (x+h) - 2 + x \] \[ 2 \cdot (-x - h) \] \[ -2x - 2h - 2x \]7. **Further Simplification:** \[ \frac{-4 - 2h}{h} \] \[ \frac{-x - 2h - 2}{h} \]### ExplanationThe work involves simplifying a mathematical expression involving radicals and using the concept of difference quotients for derivatives. The student approaches the problem by manipulating the expression to remove radicals through rationalization. This common algebraic technique is used to facilitate the computation of limits, as is typical in calculus when determining derivatives. The image contains a mathematical problem involving the function $ h(x) = \sqrt{2-x} $, with $ a = -2 $.### Problem Setup:1. **Function**: $ h(x) = \sqrt{2-x} $2. **Point**: $ a = -2 $### Derivation Process:- **Objective**: Determine the derivative $ h'(x) $ and evaluate at specific points.### Steps:1. **Expression for limit definition of a derivative**: \[ \frac{f(x+h) - f(x)}{h} \] Specifically applied to: \[ \frac{\sqrt{2-(x+h)} - \sqrt{2-x}}{h} \]2. **Simplification using conjugates**: Multiply the numerator and the denominator by the conjugate: \[ \frac{(\sqrt{2-(x+h)} - \sqrt{2-x})(\sqrt{2-(x+h)} + \sqrt{2-x})}{h(\sqrt{2-(x+h)} + \sqrt{2-x})} \]3. **Resulting expression**: - The numerator simplifies to: $-x-h$ - The full expression simplifies: \[ \frac{-x-h}{h(\sqrt{2-(x+h)} + \sqrt{2-x})} \]4. **Derivative $ h'(x) $**: Evaluating the derivative: \[ h'(x) = -\frac{1}{2\sqrt{2-x}} \] (Based on substitution and simplification.)5. **Specific evaluations**: - $ h'(-2) = -\frac{1}{2} $ - Further evaluations are suggested, but the steps aren’t filled in.### Additional Notes:- This exercise involves both algebraic manipulation and understanding of the derivative concept using limits.- The steps may include checking the rationalization of expressions using conjugates to achieve the simplified form.This solution provides a comprehensive example of applying the derivative concept to square root functions and verifying points as part of derivative evaluation.

Answered: Image /qna-images/answer/14857a3d-195c-477f-9528-fcb9a4d028a3.jpg

Answered: 3) A)-V2-a-2 3a.) (r)D 3b.) N(-2)-2…

Math

Calculus

3) A)-V2-a-2 3a.) (r)D 3b.) N(-2)-2 3c.) fxth)-f(x) 12-x+h) =D72-(x) (Jz-Cth)+

Calculus: Early Transcendentals

8th Edition

ISBN:9781285741550

Author:James Stewart

Publisher:James Stewart

Chapter1: Functions And Models

Section: Chapter Questions

Problem 1RCC: (a) What is a function? What are its domain and range? (b) What is the graph of a function? (c) How...

See similar textbooks

Related questions

Q: 3(4)0* =21

Q: How did you end up with 2 over 3 times pi? What happened to the 3 X 16 x pi? I don't understand the…

Q: 3x° (2x+16)°

Q: How do I write x2 + y2 + 8y + 2 = 0 in standard form.

A: Given,

Q: Why is it especially important to check the solutions to radical equations?

Q: simplify 6d + 3/3d / 2d + 1/4

Q: Solve the equation 6/5y - 1/6= 1/2 (3y-1)

A: To solve the given equation.

Q: I don't understand this writing. Can someone else redo the problem? Thanks!

Q: Noah says, "I worked the problem from left to right and ended up with `20-45x`."

A: NO, I don't agree with Noah. His approach is incorrect.

Q: what is 2x1/2x 3/4 =

Q: 1/3p + 7 = 25

Q: What are like radicals? Give an example.

A: We need to write what is radicals and need to give some examples. It is also known as free radicals.…

Q: 3. L se : +16,

A: The given Laplace function: f(t)=L-1se-2ts2+16 That is, to find the Laplace inverse function of, 1.…

Q: why does 2x-1=0 become x-1 = 0, why was it not devided by 2

A: I am going to solve the problem by using some simple calculus to get the required result of the…

Q: How to get rid of a radical

A: A radical is a term with one by nth power of the variable. The radical sign is as shown on the white…

Q: How do you find the value of zc?

Q: ou apply it? why do you apply it?

A: Correction Factor: This factor is applied when there is a change or it is deviating from the…

Q: 9x-6=4+8x how do I work this problem?

A: First add 6 to both sides

Q: How do you do these calculations without using excel?

A: Given data…

Q: how did you get 2x in part c?

A: Joint probability density is used to understand the probability value of several continous random…

Concept explainers

Power Operation

Power operation is topic of algebra in Math. It is use to represent repeated multiplication. Very big number and very small number can be easily express using power operation. Power operation is useful in many fields. In space engineering, it helps in representing the distance or size of particular heavenly body. In medical field, it is used to represent very small size. In medical field it helps to mention size of bacteria or virus.

Exponents

The exponent or power or index of a variable/number is the number of times that variable/number is multiplied by itself.

Question

How do I work with the radicals?

### Transcription of Math Problem Solving

1. **Expression for $ h(x) $:**
\[
h(x) = \sqrt{2 - x} - 2
\]

2. **Simplification Steps:**
\[
2 \cdot \frac{1}{2} - \frac{x}{2}
\]

3. **Derivative Approximation:**
\[
\frac{f(x+h) - f(x)}{h}
\]

4. **Simplifying the Expression:**
\[
\frac{\sqrt{2-(x+h)} - \sqrt{2-x}}{h}
\]

5. **Rewriting the Expression:**
\[
\frac{\sqrt{2-(x+h)} - \sqrt{2-x}}{h} = \frac{\left( \sqrt{2-(x+h)} - \sqrt{2-x} \right) \left( \sqrt{2-(x+h)} + \sqrt{2-x} \right)}{\sqrt{2-(x+h)} + \sqrt{2-x}}
\]

6. **Solving the Difference of Squares:**
\[
2 - (x+h) - 2 + x
\]
\[
2 \cdot (-x - h)
\]
\[
-2x - 2h - 2x
\]

7. **Further Simplification:**
\[
\frac{-4 - 2h}{h}
\]
\[
\frac{-x - 2h - 2}{h}
\]

### Explanation

The work involves simplifying a mathematical expression involving radicals and using the concept of difference quotients for derivatives. The student approaches the problem by manipulating the expression to remove radicals through rationalization. This common algebraic technique is used to facilitate the computation of limits, as is typical in calculus when determining derivatives.

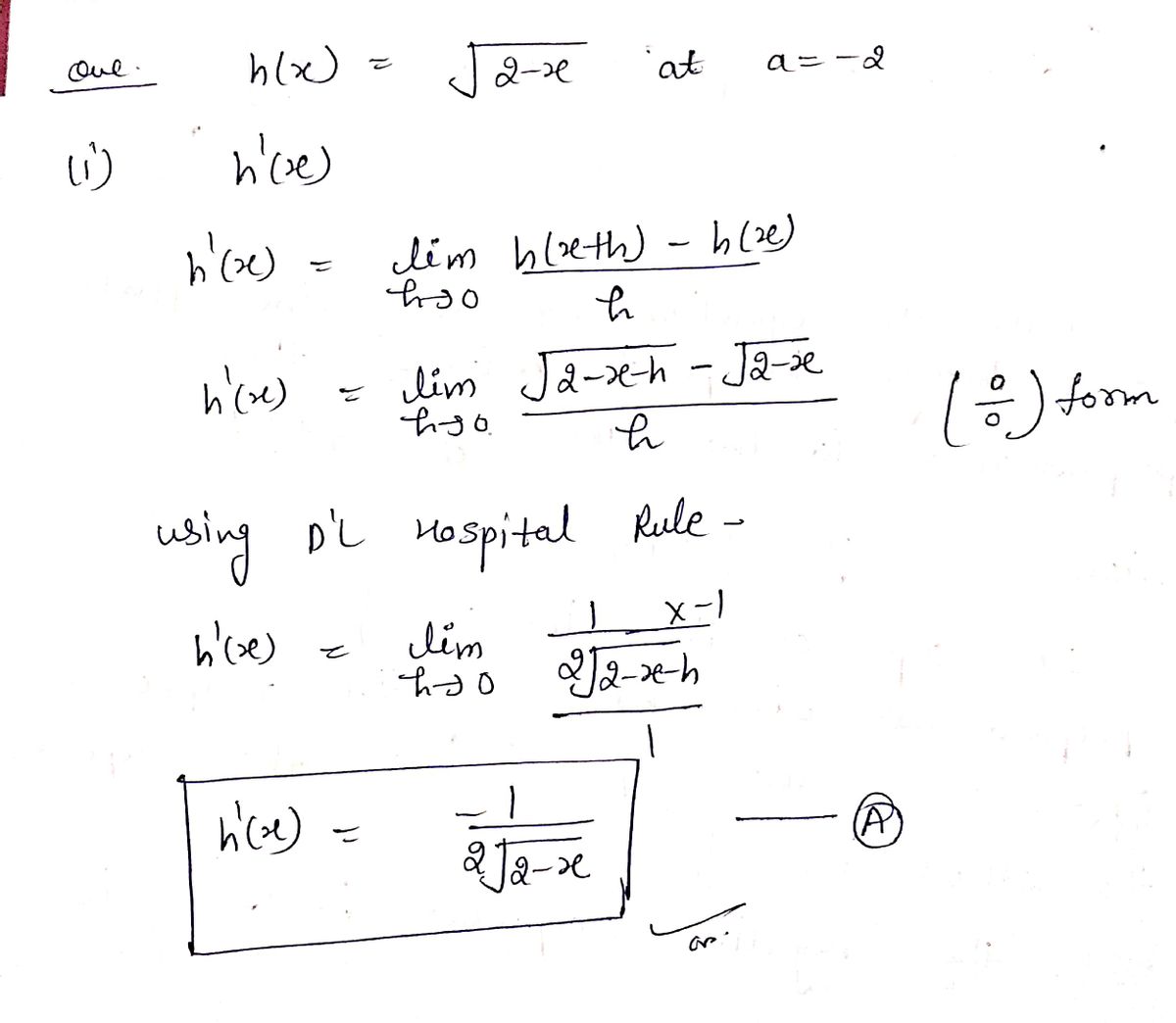
The image contains a mathematical problem involving the function $ h(x) = \sqrt{2-x} $, with $ a = -2 $.

### Problem Setup:
1. **Function**: $ h(x) = \sqrt{2-x} $
2. **Point**: $ a = -2 $

### Derivation Process:
- **Objective**: Determine the derivative $ h'(x) $ and evaluate at specific points.

### Steps:

1. **Expression for limit definition of a derivative**:
\[
\frac{f(x+h) - f(x)}{h}
\]
Specifically applied to:
\[
\frac{\sqrt{2-(x+h)} - \sqrt{2-x}}{h}
\]

2. **Simplification using conjugates**:
Multiply the numerator and the denominator by the conjugate:
\[
\frac{(\sqrt{2-(x+h)} - \sqrt{2-x})(\sqrt{2-(x+h)} + \sqrt{2-x})}{h(\sqrt{2-(x+h)} + \sqrt{2-x})}
\]

3. **Resulting expression**:
- The numerator simplifies to: $-x-h$
- The full expression simplifies:
\[
\frac{-x-h}{h(\sqrt{2-(x+h)} + \sqrt{2-x})}
\]

4. **Derivative $ h'(x) $**:
Evaluating the derivative:
\[
h'(x) = -\frac{1}{2\sqrt{2-x}}
\]
(Based on substitution and simplification.)

5. **Specific evaluations**:
- $ h'(-2) = -\frac{1}{2} $
- Further evaluations are suggested, but the steps aren’t filled in.

### Additional Notes:
- This exercise involves both algebraic manipulation and understanding of the derivative concept using limits.
- The steps may include checking the rationalization of expressions using conjugates to achieve the simplified form.

This solution provides a comprehensive example of applying the derivative concept to square root functions and verifying points as part of derivative evaluation.

Expert Solution

Step 1

Step by step

Solved in 2 steps with 2 images

SEE SOLUTION Check out a sample Q&A here

Knowledge Booster

$Exponents$

Learn more about

Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, calculus and related others by exploring similar questions and additional content below.

Similar questions