29. Construct 3 x 2 matrices A and B such that Ax = 0 has only the trivial solution and Bx = 0 has a nontrivial solution.
29. Construct 3 x 2 matrices A and B such that Ax = 0 has only the trivial solution and Bx = 0 has a nontrivial solution.
Advanced Engineering Mathematics
10th Edition
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Erwin Kreyszig
Chapter2: Second-order Linear Odes
Section: Chapter Questions
Problem 1RQ
Related questions
Question
29
![ectors
8
h
4
H
h
s 15-20
24. A is a 2 x 2 matrix with linearly dependent columns.
25. A is a 4 x 2 matrix, A = [a, a2], and a2 is not a multiple of
a₁.
26. A is a 4 x 3 matrix, A = [a az a3], such that {a₁, a₂} is
linearly independent and a; is not in Span (a, a2).
27. How many pivot columns must a 7 x 5 matrix have if its
columns are linearly independent? Why?
][1]
B
Jse Justify
kt.
28. How many pivot columns must a 5 x 7 matrix have if its
columns span RS? Why?
ndent if the
29. Construct 3 x 2 matrices A and B such that Ax = 0 has only
the trivial solution and Bx = 0 has a nontrivial solution.
or is a linear
30. a. Fill in the blank in the following statement: "If A is
an m xn matrix, then the columns of A are linearly
independent if and only if A has
pivot columns."
b. Explain why the statement in (a) is true.
Exercises 31 and 32 should be solved without performing row
operations. [Hint: Write Ax = 0 as a vector equation.]
2
3 5
-5 1-4
-3 -1 -4
10 1
31. Given A =
, observe that the third column
is the sum of the first two columns. Find a nontrivial solution
of Ax = 0.
4 1 6
5 3 observe that the first column
9-3 3
32. Given A = -7
-7
plus twice the second column equals the third column. Find
a nontrivial solution of Ax=0.
Each statement in Exercises 33-38 is either true (in all cases)
or false (for at least one example). If false, construct a specific
example to show that the statement is not always true. Such an
example is called a counterexample to the statement If
19. Sp
of
40. Supp
wby
M] In E
TO CONSITU
has only t
41. 4=](/v2/_next/image?url=https%3A%2F%2Fcontent.bartleby.com%2Fqna-images%2Fquestion%2F7cee403c-9139-49ee-992f-6fff9a4ab51f%2Fcd8eba82-300d-4e94-80a7-df36faa59020%2Fkx8fdus_processed.jpeg&w=3840&q=75)
Transcribed Image Text:ectors
8
h
4
H
h
s 15-20
24. A is a 2 x 2 matrix with linearly dependent columns.
25. A is a 4 x 2 matrix, A = [a, a2], and a2 is not a multiple of
a₁.
26. A is a 4 x 3 matrix, A = [a az a3], such that {a₁, a₂} is
linearly independent and a; is not in Span (a, a2).
27. How many pivot columns must a 7 x 5 matrix have if its
columns are linearly independent? Why?
][1]
B
Jse Justify
kt.
28. How many pivot columns must a 5 x 7 matrix have if its
columns span RS? Why?
ndent if the
29. Construct 3 x 2 matrices A and B such that Ax = 0 has only
the trivial solution and Bx = 0 has a nontrivial solution.
or is a linear
30. a. Fill in the blank in the following statement: "If A is
an m xn matrix, then the columns of A are linearly
independent if and only if A has
pivot columns."
b. Explain why the statement in (a) is true.
Exercises 31 and 32 should be solved without performing row
operations. [Hint: Write Ax = 0 as a vector equation.]
2
3 5
-5 1-4
-3 -1 -4
10 1
31. Given A =
, observe that the third column
is the sum of the first two columns. Find a nontrivial solution
of Ax = 0.
4 1 6
5 3 observe that the first column
9-3 3
32. Given A = -7
-7
plus twice the second column equals the third column. Find
a nontrivial solution of Ax=0.
Each statement in Exercises 33-38 is either true (in all cases)
or false (for at least one example). If false, construct a specific
example to show that the statement is not always true. Such an
example is called a counterexample to the statement If
19. Sp
of
40. Supp
wby
M] In E
TO CONSITU
has only t
41. 4=
Expert Solution
Step 1
29)
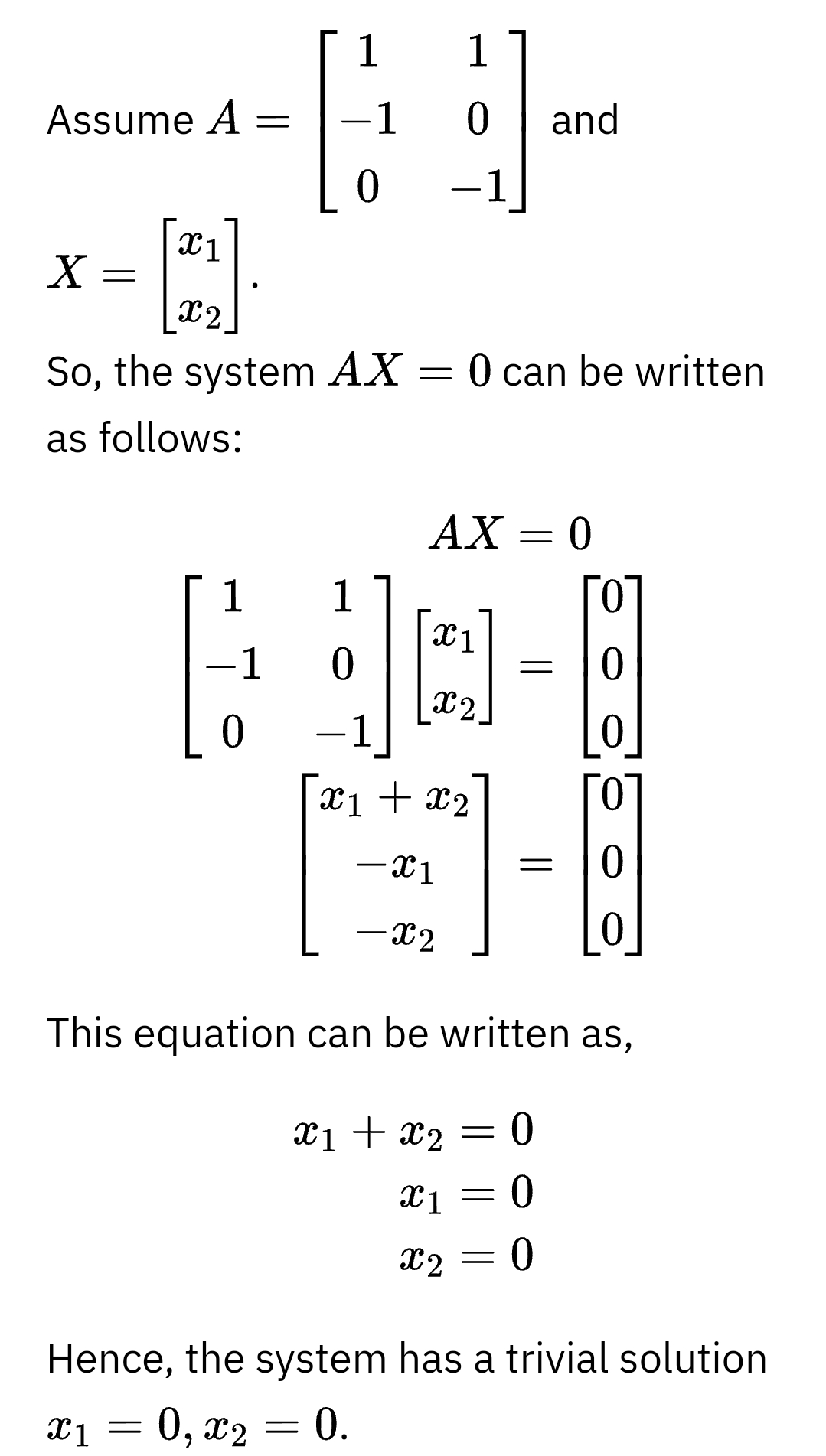
Trending now
This is a popular solution!
Step by step
Solved in 3 steps with 3 images

Recommended textbooks for you

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Mathematics For Machine Technology
Advanced Math
ISBN:
9781337798310
Author:
Peterson, John.
Publisher:
Cengage Learning,

