238 Groups Supplementary Exercises for Chapters 9-11 Every prospector drills many a dry hole, pulls out his rig, and moves on JOHN L. HESS True/false questions for Chapters 9-11 are available on the Web at: http://www.d.umn.edu/~jgallian/TF 1. Suppose that H is a subgroup of G and that each left coset of H G is some right coset of H in G. Prove that H is normal in G. 2. Use a factor group-induction argument to prove that a finite group of order n has a subgroup of order m for Abelian tive divisor m of n. every posi- 3. Let diag(G) = {(g, g) | g E G}. Prove that diag(G) GGif and only if G is Abelian. When G is finite, what is the index of diag(G) in G G? 4. Let H be any group of rotations in D. Prove that H is normal in D 5. Prove that Inn(G) Aut(G) n' n2 6. Let H be a subgroup of G. Prove that H is a normal subgroup if and only if, for alla and b in G, ab E H implies ba E H. 7. The factor group GL(2, R)/SL(2, R) is isomorphic to some very familiar group. What is the group? 8. Let k be a divisor of n. The factor group (Z(n))/((k)/(n)) is isomor phic to some very familiar group. What is the group? 9. Let а a, b, c E Q 0 1 Н — с 0 0 under matrix multiplication. a. Find Z(H). b. Prove that Z(H) is isomorphic to Q under addition. c. Prove that H/Z(H) is isomorphic to Q Q. d. Are your proofs for parts a and b valid when Q is replaced by R? Are they valid when Q is replaced by Z, where p is prime? 10. Prove that DIZ(D,) is isomorphic to Z, Z2. 11. Prove that Q/Z under addition is an infinite group in which every element has finite order. 12. Show that the intersection of any collection of normal subgroups of a group is a normal subgroup.
238 Groups Supplementary Exercises for Chapters 9-11 Every prospector drills many a dry hole, pulls out his rig, and moves on JOHN L. HESS True/false questions for Chapters 9-11 are available on the Web at: http://www.d.umn.edu/~jgallian/TF 1. Suppose that H is a subgroup of G and that each left coset of H G is some right coset of H in G. Prove that H is normal in G. 2. Use a factor group-induction argument to prove that a finite group of order n has a subgroup of order m for Abelian tive divisor m of n. every posi- 3. Let diag(G) = {(g, g) | g E G}. Prove that diag(G) GGif and only if G is Abelian. When G is finite, what is the index of diag(G) in G G? 4. Let H be any group of rotations in D. Prove that H is normal in D 5. Prove that Inn(G) Aut(G) n' n2 6. Let H be a subgroup of G. Prove that H is a normal subgroup if and only if, for alla and b in G, ab E H implies ba E H. 7. The factor group GL(2, R)/SL(2, R) is isomorphic to some very familiar group. What is the group? 8. Let k be a divisor of n. The factor group (Z(n))/((k)/(n)) is isomor phic to some very familiar group. What is the group? 9. Let а a, b, c E Q 0 1 Н — с 0 0 under matrix multiplication. a. Find Z(H). b. Prove that Z(H) is isomorphic to Q under addition. c. Prove that H/Z(H) is isomorphic to Q Q. d. Are your proofs for parts a and b valid when Q is replaced by R? Are they valid when Q is replaced by Z, where p is prime? 10. Prove that DIZ(D,) is isomorphic to Z, Z2. 11. Prove that Q/Z under addition is an infinite group in which every element has finite order. 12. Show that the intersection of any collection of normal subgroups of a group is a normal subgroup.
Advanced Engineering Mathematics
10th Edition
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Erwin Kreyszig
Chapter2: Second-order Linear Odes
Section: Chapter Questions
Problem 1RQ
Related questions
Concept explainers
Contingency Table
A contingency table can be defined as the visual representation of the relationship between two or more categorical variables that can be evaluated and registered. It is a categorical version of the scatterplot, which is used to investigate the linear relationship between two variables. A contingency table is indeed a type of frequency distribution table that displays two variables at the same time.
Binomial Distribution
Binomial is an algebraic expression of the sum or the difference of two terms. Before knowing about binomial distribution, we must know about the binomial theorem.
Topic Video
Question
4

Transcribed Image Text:238
Groups
Supplementary Exercises for Chapters 9-11
Every prospector drills many a dry hole, pulls out his rig, and moves on
JOHN L. HESS
True/false questions for Chapters 9-11 are available on the Web at:
http://www.d.umn.edu/~jgallian/TF
1. Suppose that H is a subgroup of G and that each left coset of H
G is some right coset of H in G. Prove that H is normal in G.
2. Use a factor group-induction argument to prove that a finite
group of order n has a subgroup of order m for
Abelian
tive divisor m of n.
every posi-
3. Let diag(G) = {(g, g) | g E G}. Prove that diag(G) GGif
and only if G is Abelian. When G is finite, what is the index of
diag(G) in G G?
4. Let H be any group of rotations in D. Prove that H is normal in D
5. Prove that Inn(G) Aut(G)
n'
n2
6. Let H be a subgroup of G. Prove that H is a normal subgroup if and
only if, for alla and b in G, ab E H implies ba E H.
7. The factor group GL(2, R)/SL(2, R) is isomorphic to some very
familiar group. What is the group?
8. Let k be a divisor of n. The factor group (Z(n))/((k)/(n)) is isomor
phic to some
very familiar group. What is the group?
9. Let
а
a, b, c E Q
0 1
Н —
с
0
0
under matrix multiplication.
a. Find Z(H).
b. Prove that Z(H) is isomorphic to Q under addition.
c. Prove that H/Z(H) is isomorphic to Q Q.
d. Are your proofs for parts a and b valid when Q is replaced by
R? Are they valid when Q is replaced by Z, where p is prime?
10. Prove that DIZ(D,) is isomorphic to Z, Z2.
11. Prove that Q/Z under addition is an infinite group in which every
element has finite order.
12. Show that the intersection of any collection of normal subgroups of
a group is a normal subgroup.
Expert Solution
Step 1
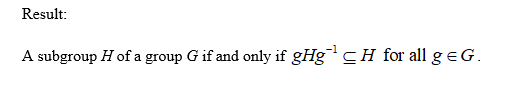
Step by step
Solved in 2 steps with 2 images

Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, advanced-math and related others by exploring similar questions and additional content below.Recommended textbooks for you

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Mathematics For Machine Technology
Advanced Math
ISBN:
9781337798310
Author:
Peterson, John.
Publisher:
Cengage Learning,

