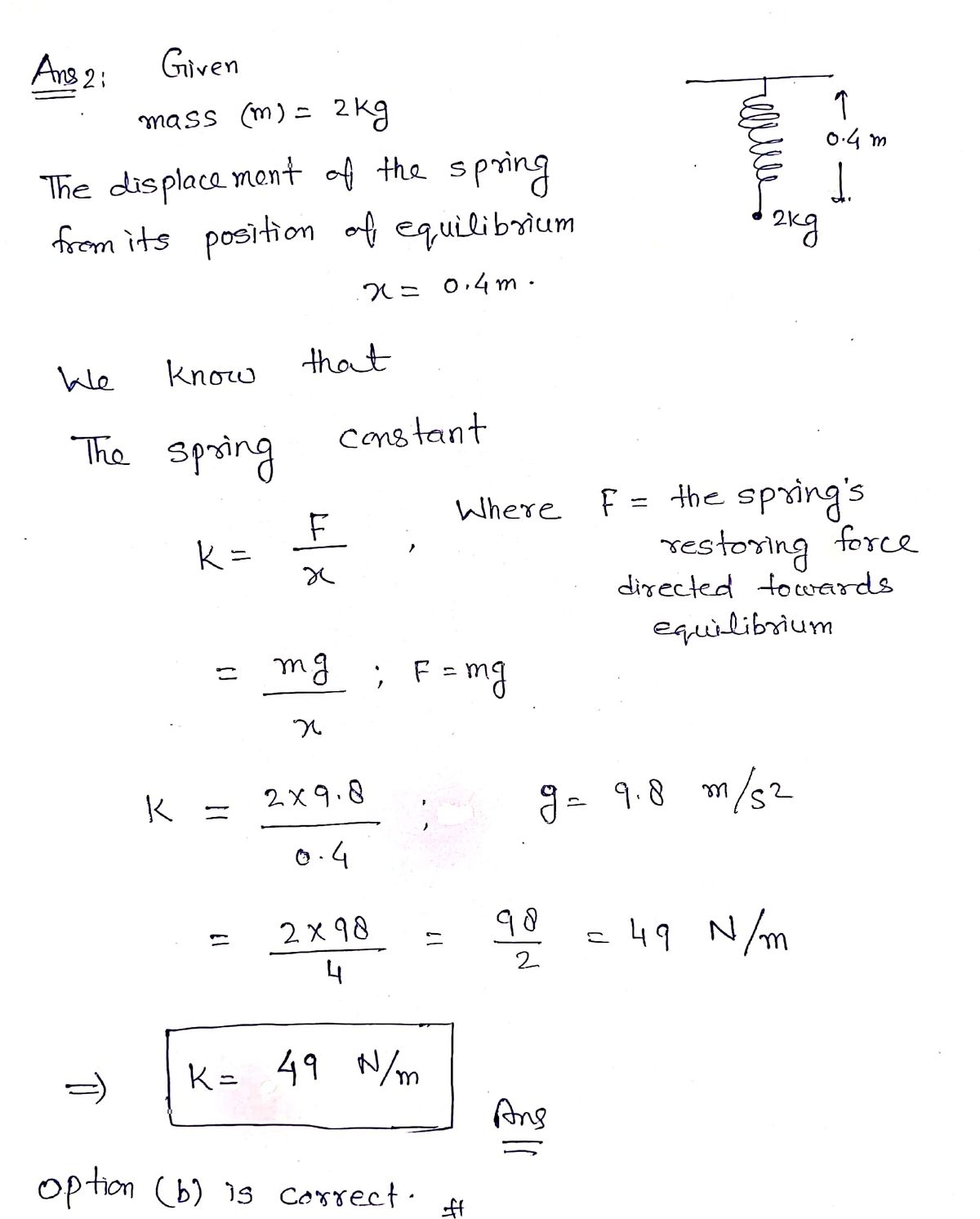
2. A 2 kg mass stretches a spring 0.4 m. The spring constant is (g = 9.8- m and m kg. = N) s2 (a) 0.2 kg/m (b) 49 N/m (c) 0.8 kg . m (d) 160 N 3. A 24 lb weight stretches a spring 3 in. The spring constant is (а) 8 b/ft (b) 76 Įb/ft (c) 6 lb/ft (d) 96 lb/ft
2. A 2 kg mass stretches a spring 0.4 m. The spring constant is (g = 9.8- m and m kg. = N) s2 (a) 0.2 kg/m (b) 49 N/m (c) 0.8 kg . m (d) 160 N 3. A 24 lb weight stretches a spring 3 in. The spring constant is (а) 8 b/ft (b) 76 Įb/ft (c) 6 lb/ft (d) 96 lb/ft
Advanced Engineering Mathematics
10th Edition
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Erwin Kreyszig
Chapter2: Second-order Linear Odes
Section: Chapter Questions
Problem 1RQ
Related questions
Question

Transcribed Image Text:**2. A 2 kg mass stretches a spring 0.4 m. The spring constant is (g = 9.8 m/s² and kg·m/s² = N)**
(a) 0.2 kg/m
(b) 49 N/m
(c) 0.8 kg·m
(d) 160 N
---
**3. A 24 lb weight stretches a spring 3 in. The spring constant is**
(a) 8 lb/ft
(b) 76 lb/ft
(c) 6 lb/ft
(d) 96 lb/ft
---
**Explanation of Concepts:**
The problems above relate to Hooke’s Law, which states that the force needed to extend or compress a spring by some distance \( x \) is proportional to that distance. This is often formulated as \( F = kx \), where \( k \) is the spring constant.
**Units:**
- In problem 2, the metric system is used with units of kilograms, meters, and Newtons (kg, m, N).
- In problem 3, the imperial system is used with units of pounds and inches (lb, in).
**Calculations:**
1. **For Problem 2:**
- Weight \( W = mg = 2 \, \text{kg} \times 9.8 \, \text{m/s}^2 = 19.6 \, \text{N} \)
- The spring constant \( k = \frac{F}{x} = \frac{19.6 \, \text{N}}{0.4 \, \text{m}} = 49 \, \text{N/m} \)
2. **For Problem 3:**
- Note that 3 inches must be converted to feet: \( 3 \, \text{in} = 0.25 \, \text{ft} \)
- Spring constant \( k = \frac{F}{x} = \frac{24 \, \text{lb}}{0.25 \, \text{ft}} = 96 \, \text{lb/ft} \)
Expert Solution
Step 1
Step by step
Solved in 2 steps with 2 images

Recommended textbooks for you

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Mathematics For Machine Technology
Advanced Math
ISBN:
9781337798310
Author:
Peterson, John.
Publisher:
Cengage Learning,

