Homework Help is Here – Start Your Trial Now!
Math
Advanced Math
-(1/t)2 for t0 2.65. If f(t) = show that f(n) (0) = 0 for all n for t= 0'

-(1/t)2 for t0 2.65. If f(t) = show that f(n) (0) = 0 for all n for t= 0'

Advanced Engineering Mathematics
Advanced Engineering Mathematics
10th Edition
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Erwin Kreyszig
Chapter2: Second-order Linear Odes
Section: Chapter Questions
Problem 1RQ
See similar textbooks
icon
Related questions
Topic Video
Question

Please rigorously prove this using mathematical induction and the difference quotient.

-(1/t)2 for t0 2.65. If f(t) = show that f(n) (0) = 0 for all n for t= 0'
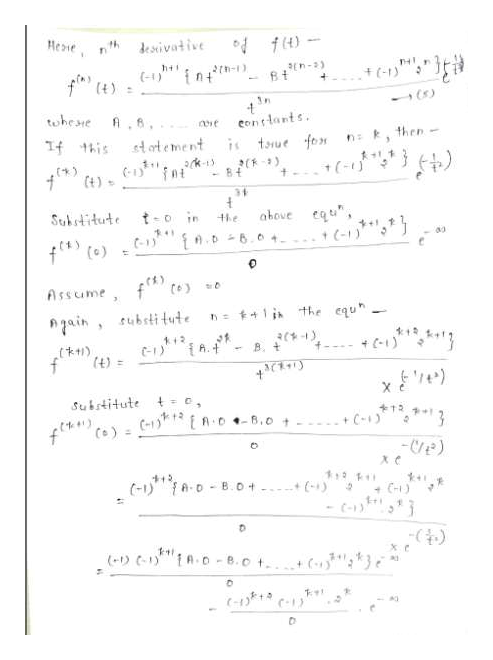
Transcribed Image Text:-(1/t)2 for t0 2.65. If f(t) = show that f(n) (0) = 0 for all n for t= 0'
Expert Solution
Step 1
fo fom tt denentinte ivn funrtin wi pet to , P A2ain biddeientite aheve fuinetion wth sespet f +6 (3) f (t) (1Rt-30c (4) Ih Mathematica Induction, we have to shows thal nataral be tue jo statement is tue fos also When (a) n , then twil ts Successo n : statement is tue fo n The () 4stue fe evesy Then the s tatement wil number n he natusal equn the in Substitute, -(010) 2 flo
Step 2
Heie hJesivative Ptn-2) ,2th-1) +(-1) (0) nt conlants i toue fo tohee A6 n the- t ort ement k- If this 3 f+) () 31 above eq" D 6.0 4 t o in Sukstitute the (-1 C Assume 1 the equ. ain ubtitute B. + (k) X e Sukstitute t +2 12 1 ** 3 +C- B.D t (-)AD B.0 ( C- ( 8.0 . + D -
steps

Step by step

Solved in 3 steps with 3 images

Blurred answer
Knowledge Booster
Propositional Calculus
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, advanced-math and related others by exploring similar questions and additional content below.
Recommended textbooks for you
Advanced Engineering Mathematics
Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated
Numerical Methods for Engineers
Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education
Introductory Mathematics for Engineering Applicat…
Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY
Mathematics For Machine Technology
Mathematics For Machine Technology
Advanced Math
ISBN:
9781337798310
Author:
Peterson, John.
Publisher:
Cengage Learning,
Basic Technical Mathematics
Basic Technical Mathematics
Advanced Math
ISBN:
9780134437705
Author:
Washington
Publisher:
PEARSON
Topology
Topology
Advanced Math
ISBN:
9780134689517
Author:
Munkres, James R.
Publisher:
Pearson,
SEE MORE TEXTBOOKS