13. Use the table below to complete parts a through d. Miles Driven Gas Left in Tank (gallons) 24 10 49 22 101 18 142 15 148 14 208 a. Write a linear equation that fits the data; (hint, make equation on desmos) b. Using your line of best fit, predict the amount of gas left in the tank after driving 125 miles. с. How many miles can you drive before you run out of gas? d. Find the correlation coefficient: e. Based on your answer from part b, select the statements that would be true of this correlation. (Select ALL that apply.) O The correlation is strong. O The correlation is weak. O The correlation is positive. O The correlation is negative. O There is no correlation at all.
Equations and Inequations
Equations and inequalities describe the relationship between two mathematical expressions.
Linear Functions
A linear function can just be a constant, or it can be the constant multiplied with the variable like x or y. If the variables are of the form, x2, x1/2 or y2 it is not linear. The exponent over the variables should always be 1.

Since you have posted a question with multiple sub-parts, we will solve the first three subparts for you. To get the remaining sub-parts solved please repost the complete question and mention the sub-parts to be solved.
a. The data is entered in a spreadsheet.
It assumed,
Y: Gas left in tank (dependant variable)
X: Miles driven (independent variable)
The steps to calculate simple regression are-
1) Go to ‘Data’ tab and click on ‘Data Analysis’.
2) Clicking ‘Data Analysis’, provides us with a list of Analysis tools and we select ‘Regression’.
3) From the resulting dialog box, select the respective cells for X-Range and Y-Range, select labels and click on ‘OK’. The regression table is then obtained.
The summary output obtained is-
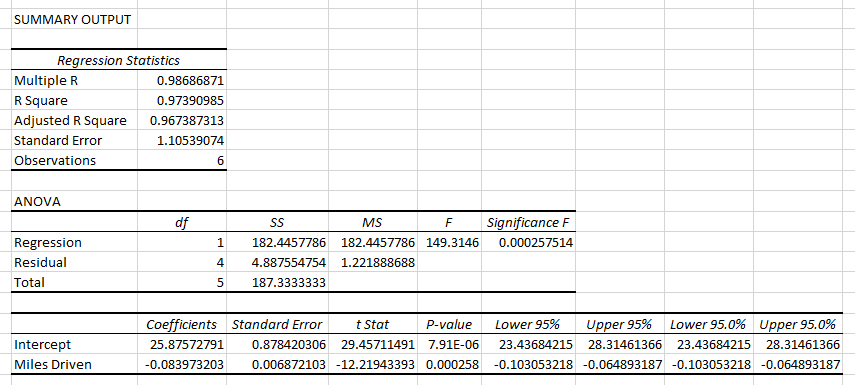
From the summary obtained the regression equation is found to be-
Y = 25.875-0.084X
Trending now
This is a popular solution!
Step by step
Solved in 2 steps with 1 images









