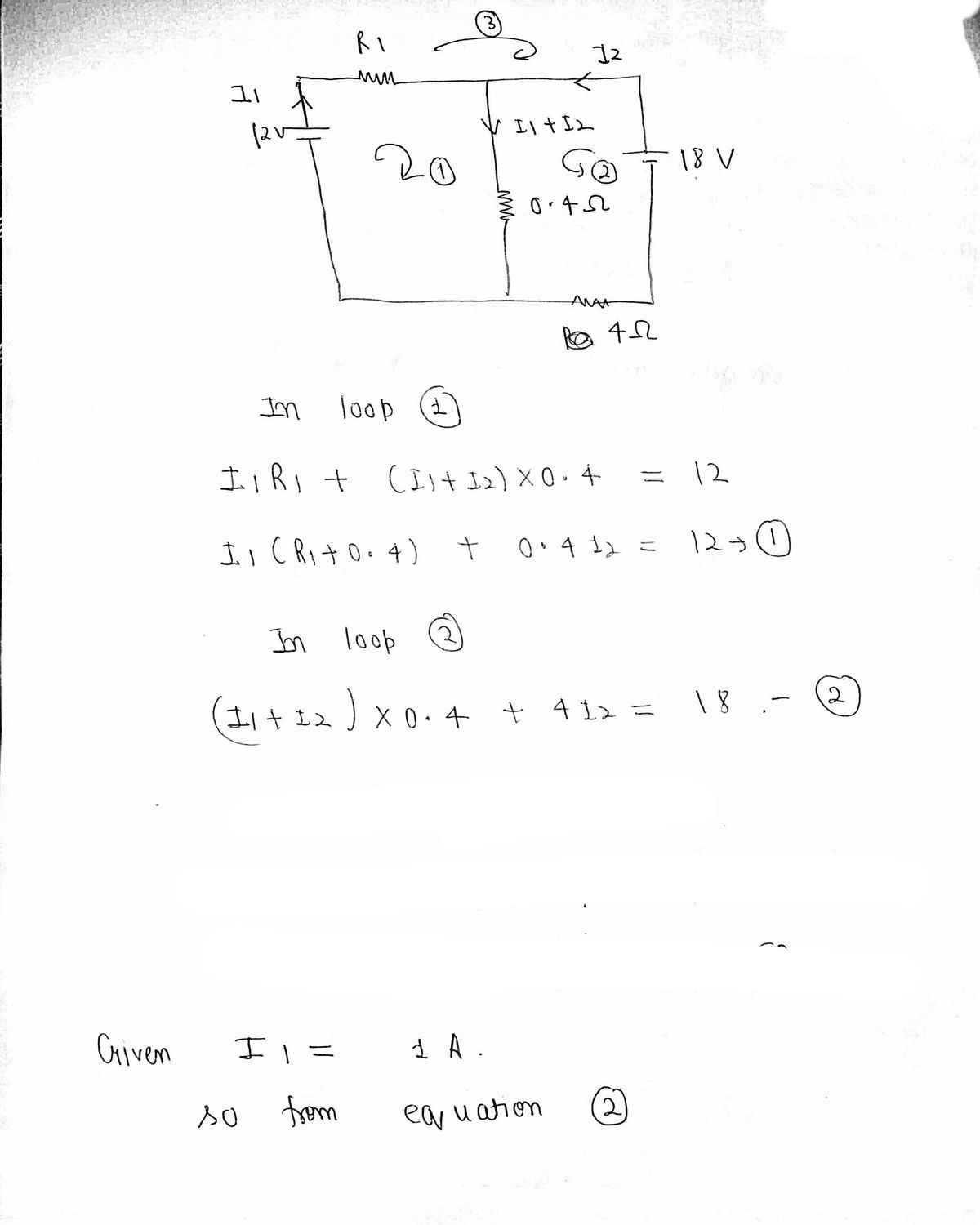
12 V 18 V R2 0.42 R3 42 In this circuit there is a current of 1A flowing to the right through resistor R1. Find the resistance of R1. Show your work.
12 V 18 V R2 0.42 R3 42 In this circuit there is a current of 1A flowing to the right through resistor R1. Find the resistance of R1. Show your work.
Related questions
Question
![### Circuit Analysis Problem
**Circuit Description:**
The diagram presents an electrical circuit featuring three resistors:
- \( R_1 \)
- \( R_2 = 0.4 \, \Omega \)
- \( R_3 = 4 \, \Omega \)
There are two voltage sources in the circuit:
- \( 12 \, \text{V} \) on the left side of \( R_1 \)
- \( 18 \, \text{V} \) on the right side of \( R_2 \)
**Current Flow:**
A current of \( 1 \, \text{A} \) is flowing to the right through resistor \( R_1 \).
**Task:**
Determine the resistance of \( R_1 \).
**Solution Approach:**
1. **Apply Ohm's Law:**
\[
V = I \times R
\]
2. **Utilize Kirchhoff's Voltage Law:**
The sum of the potential differences across the resistors must equal the net changes in voltage from sources.
3. **Calculate the Total Voltage:**
The voltage sources provide:
\[
18 \, \text{V} - 12 \, \text{V} = 6 \, \text{V}
\]
4. **Analyze the Voltage Drop:**
Total voltage drop across \( R_1 \), \( R_2 \), and \( R_3 \) should account for the available \( 6 \, \text{V} \).
5. **Express Voltage Drops:**
\[
V = I \times (R_1 + R_2 + R_3)
\]
\[
6 \, \text{V} = 1 \, \text{A} \times (R_1 + 0.4 \, \Omega + 4 \, \Omega)
\]
6. **Solve for \( R_1 \):**
\[
R_1 = 6 \, \text{V} / 1 \, \text{A} - 0.4 \, \Omega - 4 \, \Omega
\]
\[
R_1 = 1.6 \, \Omega
\]
**Conclusion:**
The resistance \( R_1 \) is found](/v2/_next/image?url=https%3A%2F%2Fcontent.bartleby.com%2Fqna-images%2Fquestion%2F313c41c0-bf91-42cc-904b-430da81366aa%2Fb5e820dc-881c-457d-a777-59994944767c%2Fyfbna0w_processed.jpeg&w=3840&q=75)
Transcribed Image Text:### Circuit Analysis Problem
**Circuit Description:**
The diagram presents an electrical circuit featuring three resistors:
- \( R_1 \)
- \( R_2 = 0.4 \, \Omega \)
- \( R_3 = 4 \, \Omega \)
There are two voltage sources in the circuit:
- \( 12 \, \text{V} \) on the left side of \( R_1 \)
- \( 18 \, \text{V} \) on the right side of \( R_2 \)
**Current Flow:**
A current of \( 1 \, \text{A} \) is flowing to the right through resistor \( R_1 \).
**Task:**
Determine the resistance of \( R_1 \).
**Solution Approach:**
1. **Apply Ohm's Law:**
\[
V = I \times R
\]
2. **Utilize Kirchhoff's Voltage Law:**
The sum of the potential differences across the resistors must equal the net changes in voltage from sources.
3. **Calculate the Total Voltage:**
The voltage sources provide:
\[
18 \, \text{V} - 12 \, \text{V} = 6 \, \text{V}
\]
4. **Analyze the Voltage Drop:**
Total voltage drop across \( R_1 \), \( R_2 \), and \( R_3 \) should account for the available \( 6 \, \text{V} \).
5. **Express Voltage Drops:**
\[
V = I \times (R_1 + R_2 + R_3)
\]
\[
6 \, \text{V} = 1 \, \text{A} \times (R_1 + 0.4 \, \Omega + 4 \, \Omega)
\]
6. **Solve for \( R_1 \):**
\[
R_1 = 6 \, \text{V} / 1 \, \text{A} - 0.4 \, \Omega - 4 \, \Omega
\]
\[
R_1 = 1.6 \, \Omega
\]
**Conclusion:**
The resistance \( R_1 \) is found
Expert Solution
Step 1
Step by step
Solved in 2 steps with 2 images
