10. REINFORCE Consider the function f(x) = -2x² +18. Fill in the following tables to describe f(x) and its inverse, f¹(x). Sketch a graph of f(x) and f(x) on the same graph grid. Is the inverse relation a function? Explain. f-¹(x) X f(x) agile Mind X -20 15 10 0 10 15 Page 5 of 8 20 Copyright Agile Mind, Inc. ® Content copyright Charles A. Dana Center, The University of Texas at Austin
10. REINFORCE Consider the function f(x) = -2x² +18. Fill in the following tables to describe f(x) and its inverse, f¹(x). Sketch a graph of f(x) and f(x) on the same graph grid. Is the inverse relation a function? Explain. f-¹(x) X f(x) agile Mind X -20 15 10 0 10 15 Page 5 of 8 20 Copyright Agile Mind, Inc. ® Content copyright Charles A. Dana Center, The University of Texas at Austin
Algebra and Trigonometry (6th Edition)
6th Edition
ISBN:9780134463216
Author:Robert F. Blitzer
Publisher:Robert F. Blitzer
ChapterP: Prerequisites: Fundamental Concepts Of Algebra
Section: Chapter Questions
Problem 1MCCP: In Exercises 1-25, simplify the given expression or perform the indicated operation (and simplify,...
Related questions
Question

Transcribed Image Text:**Educational Resource: Exploring Quadratic Functions and Their Inverses**
---
**Consider the Function \( f(x) = -2x^2 + 18 \):**
This exercise involves analyzing the quadratic function \( f(x) = -2x^2 + 18 \), filling in values for \( f(x) \) and its inverse \( f^{-1}(x) \), and then sketching both on a coordinate grid. It also includes determining if the inverse relation is a function.
**Tables for Values:**
- **\( x \) and \( f(x) \) Table:**
- This table is for recording outputs \( f(x) \) for given inputs \( x \).
- **\( x \) and \( f^{-1}(x) \) Table:**
- This table is meant to record values of \( x \) with corresponding \( f^{-1}(x) \), the inverse relation.
**Graph Details:**
- The graph grid has axes ranging from -5 to 20 on the x-axis and -5 to 20 on the y-axis.
- Students are expected to plot the quadratic function \( f(x) = -2x^2 + 18 \) and analyze its graph.
- Additionally, plot the values for the inverse and explore the symmetry, particularly focusing on line \( y=x \) which can help visualize relation inverses.
**Key Concepts:**
- **Inverse Relation of a Function:**
- For a quadratic like \( f(x) = -2x^2 + 18 \), the inverse may not be a function unless restricted on a specific interval because quadratics have a parabolic shape, which doesn’t pass the Horizontal Line Test.
- **Determining if Inverse is a Function:**
- Exploring if for each output there’s only one corresponding input in the inverse will be key. Graphically, the inverse would need to pass the Vertical Line Test if viewed as a function.
---
This exercise assists in understanding function inverses, graphical representation, and conceptualizing whether certain mathematical inverses satisfy the criteria to be functions themselves.
Expert Solution
Step 1
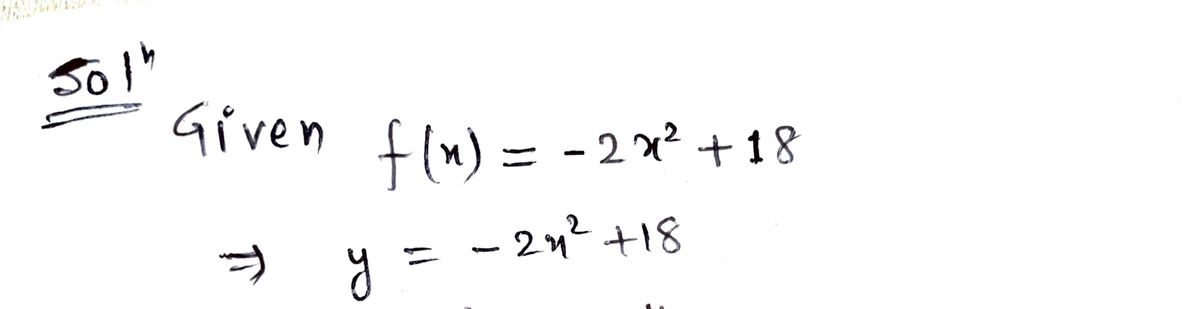
Step by step
Solved in 3 steps with 3 images

Recommended textbooks for you

Algebra and Trigonometry (6th Edition)
Algebra
ISBN:
9780134463216
Author:
Robert F. Blitzer
Publisher:
PEARSON

Contemporary Abstract Algebra
Algebra
ISBN:
9781305657960
Author:
Joseph Gallian
Publisher:
Cengage Learning

Linear Algebra: A Modern Introduction
Algebra
ISBN:
9781285463247
Author:
David Poole
Publisher:
Cengage Learning

Algebra and Trigonometry (6th Edition)
Algebra
ISBN:
9780134463216
Author:
Robert F. Blitzer
Publisher:
PEARSON

Contemporary Abstract Algebra
Algebra
ISBN:
9781305657960
Author:
Joseph Gallian
Publisher:
Cengage Learning

Linear Algebra: A Modern Introduction
Algebra
ISBN:
9781285463247
Author:
David Poole
Publisher:
Cengage Learning

Algebra And Trigonometry (11th Edition)
Algebra
ISBN:
9780135163078
Author:
Michael Sullivan
Publisher:
PEARSON

Introduction to Linear Algebra, Fifth Edition
Algebra
ISBN:
9780980232776
Author:
Gilbert Strang
Publisher:
Wellesley-Cambridge Press

College Algebra (Collegiate Math)
Algebra
ISBN:
9780077836344
Author:
Julie Miller, Donna Gerken
Publisher:
McGraw-Hill Education