1 Suppose that at t 4 the position of a particle is s(4)-8 m and its velocity is e(4) - 3 m/s. (a) Use an appropriate linearization L(t) to estimate the position of the particle at t-4.2. (b) Suppose that we know the particle's acceleration satisfies (a(t)| < 10 m/s² for all times. Determine the maximum possible value of the error (4.2) - L(4.2)
1 Suppose that at t 4 the position of a particle is s(4)-8 m and its velocity is e(4) - 3 m/s. (a) Use an appropriate linearization L(t) to estimate the position of the particle at t-4.2. (b) Suppose that we know the particle's acceleration satisfies (a(t)| < 10 m/s² for all times. Determine the maximum possible value of the error (4.2) - L(4.2)
Advanced Engineering Mathematics
10th Edition
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Erwin Kreyszig
Chapter2: Second-order Linear Odes
Section: Chapter Questions
Problem 1RQ
Related questions
Question

Transcribed Image Text:**Math 140**
**Written Homework 8: 4.1, 4.1 supplement**
**Page 1 of 4**
**Question 1**
Suppose that at \( t = 4 \) the position of a particle is \( a(4) = 8 \) m and its velocity is \( v(4) = 3 \) m/s.
- (a) Use an appropriate linearization \( L(t) \) to estimate the position of the particle at \( t = 4.2 \).
- (b) Suppose that we know the particle’s acceleration satisfies \( |a(t)| < 10 \, \text{m/s}^2 \) for all times. Determine the maximum possible value of the error \( |a(4.2) - L(4.2)| \).
**Question 2**
Oil is leaking from an uncapped well and polluting a lake. Ten days after the leak is discovered, environmental engineers measure the amount of oil in the water to be 200 gallons with a current inflow rate of 30 gallons per day. The leak is slowing so that on the tenth day, the inflow rate is decreasing by 5 gallons/day each day. Suppose \( A(t) \) is the amount of oil (in gallons) \( t \) days after the leak is discovered.
- (a) Find the quadratic approximation for \( A(t) \) centered at \( t = 10 \).
- (b) Use your answer in the previous part to estimate the amount of oil in the lake at \( t = 12 \).
**Question 3**
The period of a clock pendulum \( T \) is given by the equation \( T = 2\pi \sqrt{\frac{L}{g}} \) where the constant \( L \) is the length of the pendulum and \( g \) is the acceleration due to gravity. The period of the clock pendulum varies slightly depending on whether it is located on Earth’s surface, and on small changes in \( g \).
- Does this correspond to the clock speeding up or slowing down? Explain your reasoning.
- (a) Find the linear approximation for \( T(g) \) centered at \( g = 980 \, \text{cm/s}^2 \), if the length of the pendulum is held constant.
- (b) Suppose \(
Expert Solution
Formula
As multiple questions are posted and it is not mentioned that which specific one to be solved, the first whole question is solved as per bartleby policy.Please, post other questions separately....thank you
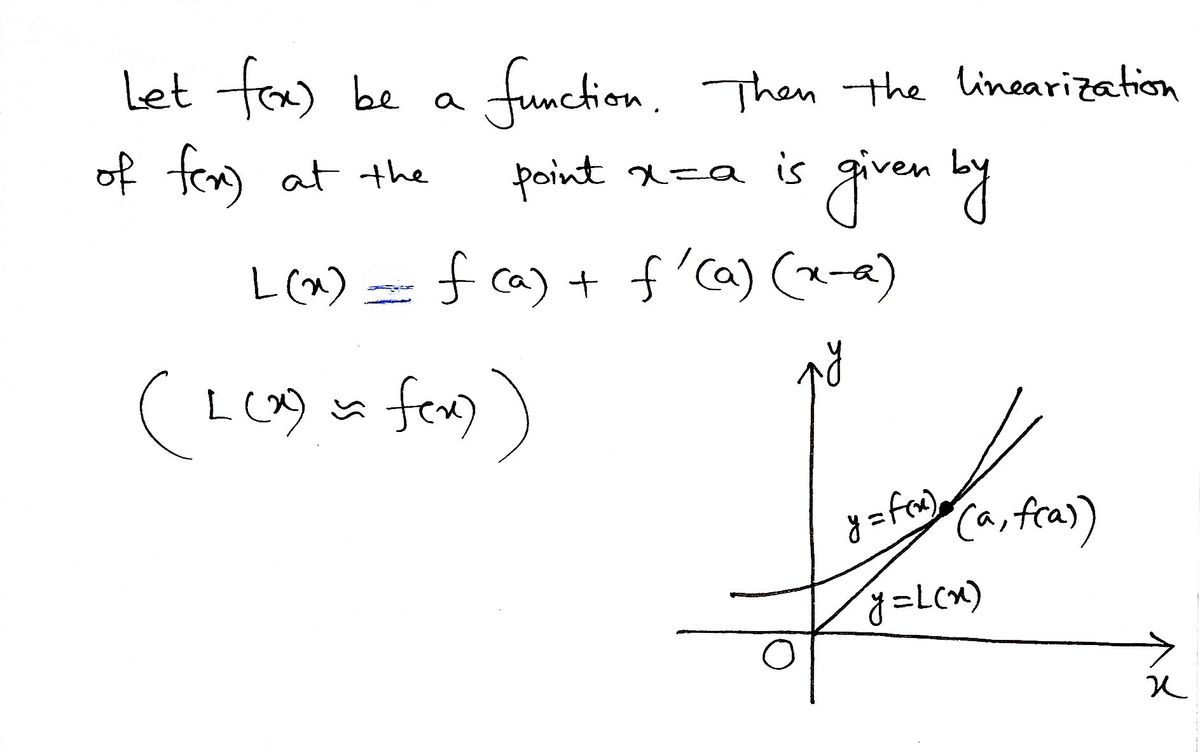
Step by step
Solved in 3 steps with 3 images

Recommended textbooks for you

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Mathematics For Machine Technology
Advanced Math
ISBN:
9781337798310
Author:
Peterson, John.
Publisher:
Cengage Learning,

