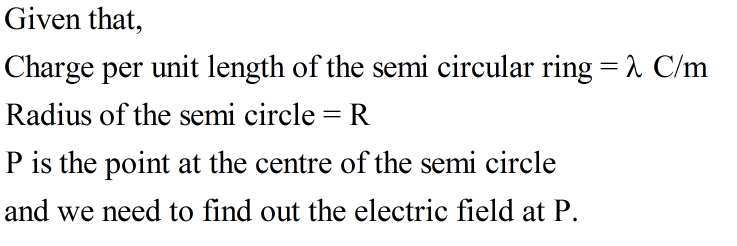
1) Consider a point located in the center of a semicircular line of charge. The radius of the semicircle is R and the line has a uniform charge density of A. Here, A is in units of Coulombs per meter and has a positive value: therefore the line itself has a net positive charge. Also, the thickness of the line itself can be ignored. For this problem, the point of interest can be envisioned as the origin of an (r,y) coordinate system, with positive r pointed toward the right and positive y pointed upward. Using this information, calculate the electric field E at the location of the point. Follow the steps below to achieve your final answer. a) Recall that a differential element dE of the electric field in terms of the differential charge element dą and the distance r may be expressed as 1 dq dE In this particular problem, what are dą and r in terms of A, R and a differential angle element d®? Hint: Here, 0 is an angle measured from the r-axis to a particular location on the line and dÐ is a differential angle element. If 0 is expressed in radians, how would you express a differential length along the line in terms of R and df? b) Describe the symmetry of the charge distribution on the line and – by extension – the direction of E at the location of the center of the line. Will there be a non-zero component of dE in the r-direction (call this component dEx)? Will there be a non-zero component of dE in the y-direction (call this component dEy)? Note that through these definitions dE = dEx + dEy. c) Express dEx and dEy in terms of dE and the appropriate trigonometric functions with 0 as the argument. d) Based on your answers to Parts (a) - (c), determine Ex and Ey – that is, the integrations of dEx and dEy – and therefore E = Ex + Ey. Over which variable do you perform the integrations for dEx and dEy? What are the bounds for the integration? Explain your answer.