1 ,2
Q: In the Marvel comics universe, Quicksilver is awfully fast. Let's say he can run at a velocity of…
A: Since Quicksilver can run at a velocity of 0.56c, we can use the Lorentz factor to determine how…
Q: Suppose a rocket starts from rest on Earth (frame S) and accelerates at a rate of 1000 m/sec² (as…
A: To solve this problem, we can use the principles of special relativity. We can start by calculating…
Q: what is the time interval between the flashes?
A: Part (d) Given: The distance at which big flash occurs is x1=1390 m. The time interval of flashes…
Q: In the laboratory inertial frame, which we assume to be inertial, two events occur simultaneously at…
A: Given data: Δx= 3 m & Δt=10-8 sTo Calculate: Spatial Distance(Δx')
Q: A spaceship travels at 0.79c, where c is the speed of light. The journey takes 2 years according to…
A:
Q: An observer in reference frame S claims that two events occur simultaneously. As this observer sees…
A: Given data- An observer in reference frame S claims two events occur simultaneously- Event A point…
Q: The factor y (gamma) appears throughout the expressions of special relativity. It depends only on…
A:
Q: Recall, from this chapter, that the factor gamma (γ) governs both time dilation and length…
A: The original time,
Q: A light flashes at position x = 0 m. One microsecond later, a light flashes at position x = 1000 m.…
A: In first reference frame S, Spatial interval of the event, ∆x=1000 m-0 m =1000 m Temporal…
Q: The elementary particle called a muon is unstable and decays in about 2.20μs, as observed in its…
A:
Q: Recall, from this chapter, that the factor gamma (γ) governs both time dilation and length…
A:
Q: George Gamow imagined a world in which the speed of light is only c = 10 mph (4.5 m/s), sothat…
A: The objective of this question is to understand the concept of relativistic effects and how they…
Q: A picture on the wall has dimensions of H x W inches. How fast does a moving observer need to go for…
A:
Q: Any process used to keep time will appear to happen more slowly in a moving object, when seen by an…
A: Option 1st and 3rd are correct option. Option 1 - Any process used to keep time will appear to…
Q: Observers in reference frame S see an explosion located at x1= 480 m. Asecond explosion occurs 5μs…
A:
Q: Two rockets are each 1800 m long in their rest frame. Rocket Orion, traveling at 0.80c relative to…
A: Let S be the earth's frame and S' be the rocket Sirius's reference frame. S' moves relative to S…
Q: The formula for length contraction gives the length of an interval on a ruler moving with velocity v…
A: The objective of the question is to find out the fraction by which the length of a meter stick is…
Q: A moving spaceship measures 20m long, but according to an observer at rest looking at it, it seems…
A: Given value--- moving spaceship measures 20m long. an observer at rest looking at it, it seems to…
Q: Recall, from this chapter, that the factor gamma (γ) governs both time dilation and length…
A: Given,
Q: Problem 1: Show that the spacetime coordinates of an event seen in two reference frames moving at a…
A: Introduction: The theory of special relativity explains how space and time are linked for objects…
Q: In their common rest frame, two stars are 92.0 light-years (ly) apart. If they are 36.0 apart as…
A:
Q: A brick of mass 1kg travels to the right at 0.9c, where c is the speed of light. It collides with…
A: Write the relativistic expression for the momentum. p=mv1−v2c2 Here, m is the mass of the particle,…
Q: An alien spaceship has a mass ofm= 3.2×109kg, and according to the crew, onboard the bow-to-stern…
A: mass of spaceship (m) = 3.2×109 kg Length measured onboard (Lo) = 680 m Length measured by…
Q: Recall, from this chapter, that the factor gamma (γ) governs both time dilation and length…
A: 0.1% of 1 kg be defined as, 0.001 kg. The energy released by conversion of mass 0.001 kg be given by…
Q: Chapter 37, Problem 006 Reference frame S' is to pass reference frame S at speed v along the common…
A: Solution: Given values, Time(∆ta)=14 svelocity(v)=0.99c
Q: Two spaceships are moving away from Earth in opposite directions, one at 0.83*c, and one at 0.83*c…
A:
Q: I need help on question 8?
A: Question no 8
Q: In the Marvel comics universe, Quicksilver is awfully fast. Let's say he can run at a velocity of…
A: A) Time measured by Quicksilver =distance measured by himHis speed =4.82 x 1050.75 x 3 x 108=0.0021…
Q: An observer in reference frame S claims that two events occur simultaneously. As this observer sees…
A:
Q: A particle has γ=18,399. a) Calculate c-v in m/s. (I would have asked for 1 - v/c, making the…
A: Given: a) γ=18,399 b) momentum, P = 1,739 MeV/c
Q: e Lorentz contraction formula in relativity theory relates the length L of an object moving at a…
A:
Q: An astronaut takes a light emitting beacon in a spaceship travelling at 0.87 c away from a…
A: Time dilation is the slowing down of a clock for an observer in relative motion w.r.t. to a…
Q: What is the velocity of an electron that has a momentum of 3.6 × 10-²¹kg. m/s? Electron mass is…
A:
recall from this chapter,that the factor gamma governs both time dilation and length contraction,where
when you multiply the time in moving frame by gamma,you get the longer(dilated) time in fixed fame.when you divide the length in moving frame by gamma,you get the shorter(contracted) length in your fixed frame.
Consider a high-speed rocket ship equipped with a flashing light source. If the frequency of flashes seen on an approaching ship is twice what it was when the ship was a fixed distance away, by how much is the period (time interval between flashes) changed? Is this period constant for a constant relative speed? For accelerated motion? Defend your answer.

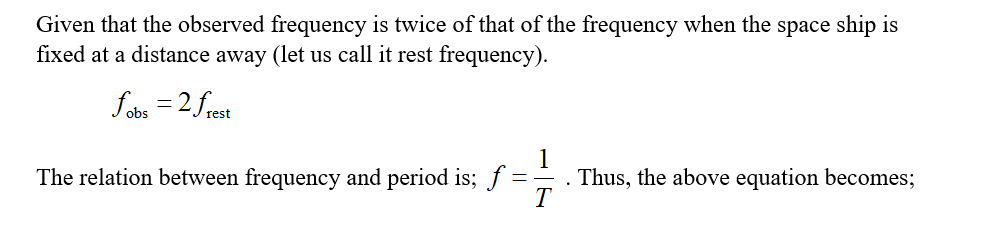

Trending now
This is a popular solution!
Step by step
Solved in 3 steps with 3 images

- You travel in a 2,500,000 kg spacecraft from Earth to our closest neighbouring star, Proxima Centauri, which is 4.22 ly (light years) away. Your spacecraft travels at 0.9 c. How much time does this take from Earth's frame of reference? (Ans: 4.7 y) How much time does it take from your frame of reference? (Ans: 2.0 y) c) What distance do you travel from your frame of reference? (Ans: 1.74x1016 m) What distance does an observer from Earth see you traveling? (Ans: 3.99 x 101 m) What would the mass of your spacecraft appear to be relative to the Earthlings?cordinates in MA particle has a lifetime of 121 nanoseconds, as measured in its own moving reference frame. It travels at a speed of 0.983c, where c is the speed of light. What distance will it travel before disintegrating, as measured in the reference frame of a fixed observer? Express your answer in meters and keep three significant digits.
- A Rigellian spaceship fleeing from battle passes Space Station Delta at an essentially constant velocity of 3/5 in the +x direction: let this be the origin event O. At event B, 80 seconds after even O as measured in the its own frame, Space Station Delta fires a photon torpedo (which travels at the speed of light) toward the fleeing ship. At event C, 100 seconds after event O in the space station frame, the Rigellians fire a laser flash (which also travels at the speed of light) back toward Space Station Delta. This laser flash destroys the torpedo at event D. a. On a sheet of hyperbola graph paper, draw the worldlines of Space Station Delta and the Rigellian ship. b. Locate events O, B, and C on the diagram. c. Draw and label the torpedo and laser flash worldlines and locate event D. What are its coordinates?Recall, from this chapter, that the factor gamma (γ) governs both time dilation and length contraction, where When you multiply the time in a moving frame by γ, you get the longer (dilated) time in your fixed fame. When you divide the length in a moving frame by γ, you get the shorter (contracted) length in your fixed frame. Assume that rocket taxis of the future move about the solar system at half the speed of light. For a 1-hour trip as measured by a clock in the taxi, a driver is paid 10 stellars. The taxi-driver’s union demands that pay be based on Earth time instead of taxi time. If their demand is met, show that the new payment for the same trip would be 11.5 stellars.To understand length contraction and time dilation. An inertial frame of reference is one in which Newton's laws hold. Any frame of reference that moves at a constant speed relative to an inertial frame of reference is also an inertial frame. The proper length l0 of an object is defined to be the length of the object as measured in the object's rest frame. If the length of the object is measured in any other inertial frame, moving with speed u relative to the object's rest frame (in a direction parallel to l0), the resulting length lll is given by the length contraction equation l= l0 *sqrt(1-u^2/ c^2) where c is the speed of light. Similarly, if two events occur at the same spatial point in a particular reference frame, and an observer at rest in this frame measures the time interval between these two events, the time interval so measured is defined to be the proper time Δt0. When the time interval is measured in any other inertial frame, again moving with speed uuu relative to the…
- An interstellar space probe is launched from Earth. After a brief period of acceleration, it moves with a constant velocity, 74.0% of the speed of light. Its nuclear-powered batteries supply the energy to keep its data transmitter active continuously. The batteries have a lifetime of 19.4 years as measured in a rest frame. Note that radio waves travel at the speed of light and fill the space between the probe and Earth at the time the battery fails. (a) How long do the batteries on the space probe last as measured by mission control on Earth? (Ignore the delay between the time the battery fails and the time mission control stops receiving the signal.) yr (b) How far is the probe from Earth when its batteries fail as measured by mission control? (Ignore the delay between the time the battery fails and the time mission control stops receiving the signal.) ly (c) How far is the probe from Earth as measured by its built-in trip odometer when its batteries fail? ly (d) For what total time…Please derive the Lorentz transformation equations for velocities. For an object, the velocity components seen in reference frame S are: ??, ??, ??. There is a reference frame S’ is moving relative to reference frame S in the direction of +?̂. Derive ?′?, ?′?, ?′?. Please show your derivation details.An astronaut is traveling at a constant velocity of 0.8 times the speed of light (c = 3 x 10^8 m/s) away from a space station. If the astronaut's clock is ticking normally, but the space station observes their clock to be running slower due to time dilation, calculate the time interval experienced by the astronaut during a 1-hour period according to their own clock.
- 1. You are an observer in a 100-m long spacecraft traveling from the earth to the moon at 0.8c. (a)What is the proper length of the spacecraft? (b) For a proper time interval of 1 sec., the relativistic time interval for the spacecraft measured from the earth reference frame would be: (c)Time dilation does not apply to all time-dependent physical and biological processes. T/F? (c) What is the relativistic length, DL measured from the reference frame of earth? (d) An APOLLO crew left a flat mirror reflector on the surface of the moon (for all you deniers out there, in the 50th anniversary year of APOLLO 11!). If the average surface-to-surface distance from the earth to the moon is 3.83 x 10^8 m, then how long does it take moonlight to reach earth?In frame S, event B occurs 2 ms after event A and at Dx = 1.5 km from event A. (a) How fast must an observer be moving along the +x axis so that events A and B occur simultaneously? (b) Is it possible for event B to precede event A for some observer?(c) Draw a spacetime diagram that illustrates your answers to (a) and (b). (d) Compute the spacetime interval and proper distance between the events.The proper length of one spaceship is three times that of another. The two spaceships are traveling in the same direction and, while both are passing overhead, an Earth observer measures the two spaceships to have the same length. If the slower spaceship has a speed of 0.354c with respect to Earth, determine the speed of the faster spaceship. (Give your answer to at least 3 significant figures.) |c