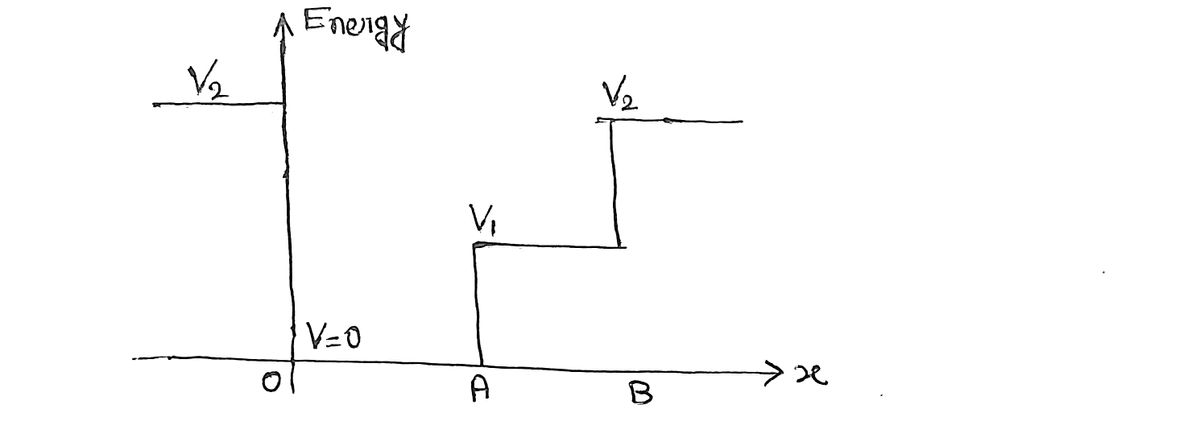
. A quantum particle of mass m is in the potential given in the figure below. Between x = 0 and x = A the potential is zero and between x = A and x = B the potential is V₁. Outside this region (if x ≥ B or x ≤ 0), the potential is V2. Assume the particle has total energy E which is less than V₂ but greater than V₁, so classically the particle would be trapped between x = 0 and x = B. Energy A V₂ IN V₁ V=0 0 A V₂ B X (a) If this were a classical particle, would it have more kinetic energy (and therefore more momentum) in the region where the potential is zero or in the region where the potential is V₁? Explain. = (b) In the region where V = 0, the particle's wavefunction satisfies the time-independent Schrödinger equation, which is ²2(2) -ky(x) for some constant k₁. What is k₁ in terms of the values given in the problem? What is the corresponding wavelength A₁? d.x² (c) In the region where V = V₁, the particle's wavefunction satisfies the time-independent d²v(x) Schrödinger equation, which is -k(x) for a different constant k₂. What is k₂ dx² in terms of the values given in the problem? What is the corresponding wavelength λ₂?
. A quantum particle of mass m is in the potential given in the figure below. Between x = 0 and x = A the potential is zero and between x = A and x = B the potential is V₁. Outside this region (if x ≥ B or x ≤ 0), the potential is V2. Assume the particle has total energy E which is less than V₂ but greater than V₁, so classically the particle would be trapped between x = 0 and x = B. Energy A V₂ IN V₁ V=0 0 A V₂ B X (a) If this were a classical particle, would it have more kinetic energy (and therefore more momentum) in the region where the potential is zero or in the region where the potential is V₁? Explain. = (b) In the region where V = 0, the particle's wavefunction satisfies the time-independent Schrödinger equation, which is ²2(2) -ky(x) for some constant k₁. What is k₁ in terms of the values given in the problem? What is the corresponding wavelength A₁? d.x² (c) In the region where V = V₁, the particle's wavefunction satisfies the time-independent d²v(x) Schrödinger equation, which is -k(x) for a different constant k₂. What is k₂ dx² in terms of the values given in the problem? What is the corresponding wavelength λ₂?
Related questions
Question
Please answer !!
![### Problem Statement
A quantum particle of mass \( m \) is in the potential given in the figure below. Between \( x = 0 \) and \( x = A \), the potential is zero. Between \( x = A \) and \( x = B \), the potential is \( V_1 \). Outside this region (if \( x \geq B \) or \( x \leq 0 \)), the potential is \( V_2 \). Assume the particle has total energy \( E \) which is less than \( V_2 \) but greater than \( V_1 \), so classically the particle would be trapped between \( x = 0 \) and \( x = B \).
### Diagram Explanation
The diagram depicts a step potential with three distinct regions:
- **Region 1**: \( 0 \leq x < A \), potential \( V = 0 \).
- **Region 2**: \( A \leq x < B \), potential \( V = V_1 \).
- **Region 3**: \( x \geq B \) or \( x \leq 0 \), potential \( V = V_2 \).
### Questions
**(a)** If this were a classical particle, would it have more kinetic energy (and therefore more momentum) in the region where the potential is zero or in the region where the potential is \( V_1 \)? Explain.
**(b)** In the region where \( V = 0 \), the particle’s wavefunction satisfies the time-independent Schrödinger equation:
\[
\frac{d^2\psi(x)}{dx^2} = -k_1^2 \psi(x)
\]
for some constant \( k_1 \). What is \( k_1 \) in terms of the values given in the problem? What is the corresponding wavelength \( \lambda_1 \)?
**(c)** In the region where \( V = V_1 \), the particle’s wavefunction satisfies the time-independent Schrödinger equation:
\[
\frac{d^2\psi(x)}{dx^2} = -k_2^2 \psi(x)
\]
for a different constant \( k_2 \). What is \( k_2 \) in terms of the values given in the problem? What is the corresponding wavelength \( \lambda_2 \)?](/v2/_next/image?url=https%3A%2F%2Fcontent.bartleby.com%2Fqna-images%2Fquestion%2Fe6208a55-bec6-433a-a894-0742aca7c9d7%2F3b7f82fe-bf04-4ae3-bf92-83944b1ab7b6%2Fund4bl_processed.jpeg&w=3840&q=75)
Transcribed Image Text:### Problem Statement
A quantum particle of mass \( m \) is in the potential given in the figure below. Between \( x = 0 \) and \( x = A \), the potential is zero. Between \( x = A \) and \( x = B \), the potential is \( V_1 \). Outside this region (if \( x \geq B \) or \( x \leq 0 \)), the potential is \( V_2 \). Assume the particle has total energy \( E \) which is less than \( V_2 \) but greater than \( V_1 \), so classically the particle would be trapped between \( x = 0 \) and \( x = B \).
### Diagram Explanation
The diagram depicts a step potential with three distinct regions:
- **Region 1**: \( 0 \leq x < A \), potential \( V = 0 \).
- **Region 2**: \( A \leq x < B \), potential \( V = V_1 \).
- **Region 3**: \( x \geq B \) or \( x \leq 0 \), potential \( V = V_2 \).
### Questions
**(a)** If this were a classical particle, would it have more kinetic energy (and therefore more momentum) in the region where the potential is zero or in the region where the potential is \( V_1 \)? Explain.
**(b)** In the region where \( V = 0 \), the particle’s wavefunction satisfies the time-independent Schrödinger equation:
\[
\frac{d^2\psi(x)}{dx^2} = -k_1^2 \psi(x)
\]
for some constant \( k_1 \). What is \( k_1 \) in terms of the values given in the problem? What is the corresponding wavelength \( \lambda_1 \)?
**(c)** In the region where \( V = V_1 \), the particle’s wavefunction satisfies the time-independent Schrödinger equation:
\[
\frac{d^2\psi(x)}{dx^2} = -k_2^2 \psi(x)
\]
for a different constant \( k_2 \). What is \( k_2 \) in terms of the values given in the problem? What is the corresponding wavelength \( \lambda_2 \)?
Expert Solution
Step 1
Step by step
Solved in 2 steps with 2 images
