What does the term Trigonometry mean?
The mathematical branch that deals with the angles and the sides of a right-angled triangle is called trigonometry. Two Greek words: “trigonon” and “metron” form the word trigonometry, where the meaning of the word trigonon is a triangle and the meaning of the word metron is to measure. One of the complex parts of mathematics is trigonometry, and its functions are known as trigonometric functions.
Measurements of Angles
The intersection of two lines or rays results in the formation of an angle, and the two rays are then called the sides or arms of the angle. The arm where the angle starts is known as the initial side, and the other arm is known as the terminal side. As soon as the initial ray goes along the positive x-axis and the origin contains the vertex, this condition states that the initial side is in standard position.
The rotation between the terminal and the initial side is used to determine the angle. When the rotation is anti-clockwise, the angle is positive, and when it is clockwise, the angle is negative.
The measurement of the angle can be done in two different ways:
1. Degrees
2. Radians
1. Degrees: - A degree is considered as of the angle which represents one revolution.Initially, the angle is measured in degree.
2. Radians: - One revolution measured in radians is 2π, where π is a constant, whose value is approximately 3.14.
Unit Circle
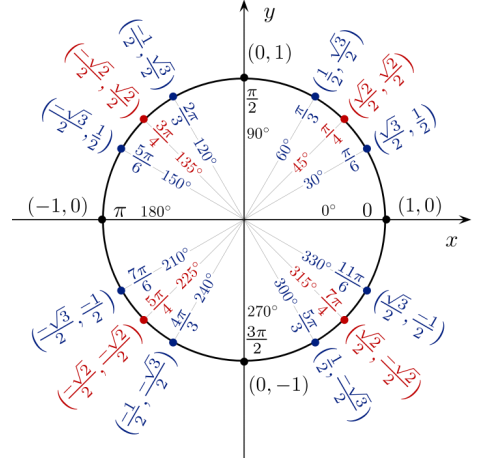
A circle whose radius is always one and the centre is at the origin is known as the unit circle. The trigonometry ratios are defined using the unit circle.
What is Trigonometric Function (Trig Function)?
There are six ratios (trigonometric functions) that justify the angles of a right-angled triangle (right triangle) and the side lengths. Remember that a right-angled triangle contains an angle of 90°. Consider a right-angled triangle that consists of an acute angle 𝜃 with the initial side of length ‘x’ and the terminal side of length ‘r’. Consider that the length of the remaining side is ‘y’. Here ‘r’ denotes the hypotenuse.
The Pythagorean theorem states that the square of the hypotenuse of a right triangle is the same as the sum of the squares of the lengths of the other two sides.
According to the Pythagorean Theorem: .
Therefore, .
𝜃 (theta) is used as an angle for the following six trigonometric functions:
- sine (sin)
- cosine (cos)
- tangent (tan)
- cosecant (csc)
- secant (sec)
- cotangent (cot)
The ratios for the three basic trigonometric functions are as follows:
1.
2.
3.
These three trigonometric functions have three reciprocal functions.
- The reciprocal function of sine is called the cosecant function.
5.The reciprocal function of cosine is called the secant function.
6. The reciprocal function of tangent is called the cotangent function.
Example:
Determine the values of the six trigonometric functions having angle theta.
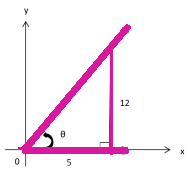
Before we can get the six trigonometric ratios, the missing side length is to be found.
For this, we can use the Pythagorean theorem.
Now,
Fundamentals Identities of Trigonometry Function
Reciprocal Identity
Quotient Identities
Pythagorean Identities
Negative Angle Identities
1. sin(−𝜃) = − sin 𝜃.
2. cos(−𝜃) = cos 𝜃.
3. tan(−𝜃) = − tan 𝜃.
4. csc(−𝜃) = − csc 𝜃.
5. sec(−𝜃) = sec 𝜃.
6. cot(−𝜃) = − cot 𝜃.
Co-Function Identities
When the summation of two angles is 90°, they are said to be complementary to each other. The functions f and g are said to be co-functions of each other if f(a)=g(b) whenever a and b are complementary angles. Below mentioned are the trigonometric co-function identities:
1.
2.
3.
4.
5.
6.
Formulae for Sum and Difference
- sin(x + t) = sin x cos t + cos x sin t.
- sin(x − t) = sin x cos t − cos x sin t.
- cos(x + t) = cos x cos t − sin x sin t.
- cos(x − t) = cos x cos t + sin x sin t.
5.
6.
Example 1: - Find the exact value of sin 15°.
Example 2: - Find the exact value of tan 75°
Similarly, for cot 75°, we can solve as a reciprocal of a tangent function.
Trigonometry Function of Double and Half Angles
For Double Angle
Formula for Half Angle
- cos 𝜃/2 = ±√ [(1+cos 𝜃)/2].
- sin 𝜃/2 = ±√ [(1−cos 𝜃)/2].
- tan 𝜃/2 = ±√ [(1−cos 𝜃)/(1+cos 𝜃)].
- tan 𝜃/2 = [(sin 𝜃/(1+cos 𝜃)].
- tan 𝜃/2 = (1−cos 𝜃)/ sin 𝜃.
Product to Sum Formulas
cos 𝐴 cos𝐵 = 1/2 [cos(𝐴 + 𝐵) + cos(𝐴 − 𝐵)].
sin 𝐴 sin𝐵 = 1/2 [cos(𝐴 − 𝐵) − cos(𝐴 + 𝐵)].
sin 𝐴 cos𝐵 = 1/2 [sin(𝐴 + 𝐵) + sin(𝐴 − 𝐵)].
cos 𝐴 sin 𝐵 = 1/2 [sin(𝐴 + 𝐵) − sin(𝐴 − 𝐵)].
Example: - Change the term sin 75° sin 15° to sum using the product to sum rule.
sin 75° sin 15° = 1/2 [cos(75° − 15°) − cos(75° + 15°)].
sin 75° sin 15° = 1/2 [cos 60° − cos 90°].
sin 75° sin 15° = 1/2 [ 1/2 − 0].
sin 75° sin 15° = 1/4.
Sine and Cosine Rule/Law
The rule is helpful for oblique triangles.
Sine Law
Let the side length opposite to vertex A be a. Similarly, the side length opposite to vertex B is b, and the side length opposite to vertex C is c. Then, as per the sine law, we have:
The sine law can be used when some side lengths and angles are given in the problem.
Law of Cosine
Cosine law is used when only one angle and the other three sides are given in the problem.
Graphing Trigonometry Functions
This section will be used to brief the graphing of three trigonometric functions, sine (sine function), cosine (cosine function), and tangent (tangent function). It will state to identify the graph of the trigonometric function and its key features. The sine and the cosine are referred to as periodic functions, which means if the graph is made, it will repeat after intervals for that trig function.
A periodic trig function relation can be defined as:
(𝑥) = 𝑓(𝑥 + 𝑛𝑝), where n denotes an integer.
We know that one revolution of the unit circle is measured as 2π radians, which means that the circumference of the unit circle is 2π. It is the period for sine and cosine.
Graph of the Sine Function (𝒚 = 𝒔𝒊𝒏 𝒙)
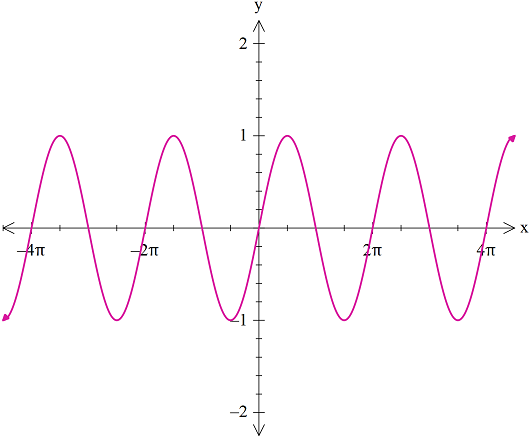
. The range and domain of the sine function is [-1, 1] and (-∞, ∞), respectively.
2. It is defined as an odd function because of the symmetry with the origin.
3. The repetition of the sine function occurs at intervals of 2𝜋.
Graph of the Cosine Function (𝒚 = 𝒄𝒐𝒔 𝒙)
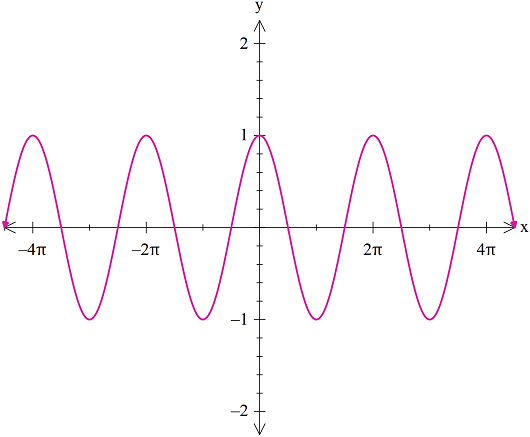
The scope (range) of the cosine function is [-1, 1], and the domain for the cosine function is (-∞, ∞).
2. This is an even function because it is symmetric with respect to the y-axis.
3. The repetition of the cos function occurs at the intervals of 2𝜋.
Graph of the Tangent Function (𝒚 = tan 𝒙)
!["Graph of tangent"]](https://cms-media.bartleby.com/wp-content/uploads/sites/2/2021/07/01122237/trigonometric-function-5.png)
1. Range of the tangent function is (−∞, ∞), and the domain is represented as {𝑥 | 𝑥 ≠ 𝑛𝜋 + 𝜋 / 2, 𝑤ℎ𝑒𝑟𝑒 𝑛 𝑖𝑠 𝑎𝑛𝑦 𝑖𝑛𝑡𝑒𝑔𝑒𝑟}.
2. The repetition of the tan function occurs at intervals of 𝜋.
3. When the numerator representing the sine function is zero, the tangent stands out to be zero.
4. When the value of the denominator representing the cosine function is zero, the tangent stands out to be undefined.
5. Vertical asymptotes are present in the tangent function graph, and the asymptotes' equations are given as 𝑥 = 𝑛𝜋 + 𝜋/2, where n is an integer.
6. The function is an odd function as the graph is symmetrical about the origin.
Inverse Trigonometric Function
The inverse trigonometric function is not the same as the reciprocal of the trigonometric function. Instead, the inverse trigonometric functions are found using the inverse relation of the given trigonometric functions. Some of the inverse trigonometric functions are as follows.
- Inverse Sine:
- Inverse Cos:
- Inverse Tan:
Common Mistakes
- All the trigonometric functions (sine, cosine, tangent, cosec, secant, and cot) are positive in the first quadrant.
- The trigonometry functions sine and cosecant are positive in the second quadrant.
- The trigonometry functions tangent and cotangent are positive in the third quadrant.
- The trigonometry functions cosine and secant are positive in the fourth quadrant.
Formulas
- Law of Cosine: -
- Product to Sum Formulas
cos 𝐴 cos𝐵 = 1/2 [cos(𝐴 + 𝐵) + cos(𝐴 − 𝐵)].
sin 𝐴 sin𝐵 = 1/2 [cos(𝐴 − 𝐵) − cos(𝐴 + 𝐵)].
sin 𝐴 cos𝐵 = 1/2 [sin(𝐴 + 𝐵) + sin(𝐴 − 𝐵)].
cos 𝐴 sin 𝐵 = 1/2 [sin(𝐴 + 𝐵) − sin(𝐴 − 𝐵)].
- For sine and cosine ratios formula
sin(x + t) = sin x cos t + cos x sin t.
sin(x − t) = sin x cos t − cos x sin t.
cos(x + t) = cos x cos t − sin x sin t.
cos(x − t) = cos x cos t + sin x sin t.
Context and Applications
This topic is significant in the professional exams for undergraduate and postgraduate courses, especially for:
- B.Sc. in Mathematics
- M.Sc. in Mathematics
Want more help with your trigonometry homework?
*Response times may vary by subject and question complexity. Median response time is 34 minutes for paid subscribers and may be longer for promotional offers.
Search. Solve. Succeed!
Study smarter access to millions of step-by step textbook solutions, our Q&A library, and AI powered Math Solver. Plus, you get 30 questions to ask an expert each month.
Trigonometric Function Homework Questions from Fellow Students
Browse our recently answered Trigonometric Function homework questions.
Search. Solve. Succeed!
Study smarter access to millions of step-by step textbook solutions, our Q&A library, and AI powered Math Solver. Plus, you get 30 questions to ask an expert each month.