Chapter 8.4, Problem 22P

Expand Your Knowledge: Confidence Intervals for μ_d Using techniques from Section 7.2, we can find a confidence interval for μ_d. Consider a random sample of n matched data pairs A, B. Let d = B – A be a random variable representing the difference between the values in a matched data pair. Compute the sample mean d of the differences and the sample standard deviation s_d. If d has a normal distribution or is mound-shaped, or if n ≥ 30, then a confidence interval for μ_d is

$\bar{d}-E<{\mu }_d<\bar{d}+E$

where $E=t_c\frac{S_d}{\sqrt{n}}$

c = confidence level (0 < c < 1)

t_c = critical value for confidence level c and d.f. = n – 1

1. (a) Using the data of Problem 9, find a 95% confidence interval for the mean difference between percentage increase in company revenue and percentage increase in CEO salary.
2. (b) Use the confidence interval method of hypothesis testing outlined in Problem 25 of Section 8.2 to test the hypothesis that population mean percentage increase in company revenue is different from that of CEO salary. Use a 5% level of significance.

9. Business: CEO Raises Are America’s top chief executive officers (CEOs) really worth all that money? One way to answer this question is to look at row B, the annual company percentage increase in revenue, versus row A, the CEO’s annual percentage salary increase in that same company (Source: Forbes, Vol. 159, No. 10). A random sample of companies such as John Deere & Co., General Electric, and Dow Chemical yielded the following data:

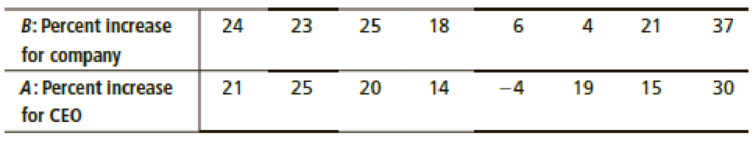
Do these data indicate that the population mean percentage increase in corporate revenue (row B) is different from the population mean percentage increase in CEO salary? Use a 5% level of significance.

25. Expand Your Knowledge: Confidence Intervals and Two-Tailed Hypothesis Tests Is there a relationship between confidence intervals and two-tailed hypothesis tests? Let c be the level of confidence used to construct a confidence interval from sample data. Let a be the level of significance for a two-tailed hypothesis test. The following statement applies to hypothesis tests of the mean.

For a two-tailed hypothesis test with level of significance α and null hypothesis H₀: µ = k, we reject H₀ Whenever k falls outside the c = 1 – α confidence interval for µ based on the sample data When k falls within the c = 1 – α confidence interval, we do not reject H₀.

(A corresponding relationship between confidence intervals and two-tailed hypothesis tests also is valid for other parameters, such as p, μ₁ – μ₂, and p₁ – p₂, which we will study in Sections 8.3 and 8.5.) Whenever the value of k given in the null hypothesis falls outside the c = 1 – α confidence interval for the parameter, we reject H₀. For example, consider a two-tailed hypothesis test with α = 0.01 and

H₀: µ = 20    H₁: µ ≠ 20

A random sample of size 36 has a sample mean $\bar{x}$ = 22 from a population with standard deviation σ = 4.

1. (a) What is the value of c = 1 – α? Using the methods of Chapter 7, construct a 1 – α confidence interval for μ from the sample data. What is the value of m given in the null hypothesis (i.e., what is k)? Is this value in the confidence interval? Do we reject or fail to reject H₀ based on this information?
2. (b) Using methods of this chapter, find the P-value for the hypothesis test. Do we reject or fail to reject H₀? Compare your result to that of part (a).