Algebra 2
Algebra 2
1st Edition
ISBN: 9780078884825
Author: McGraw-Hill/Glencoe
Publisher: Glencoe/McGraw-Hill School Pub Co
bartleby

Concept explainers

Family of Curves
A family of curves is a group of curves that are each described by a parametrization in which one or more variables are parameters. In general, the parameters have more complexity on the assembly of the curve than an ordinary linear transformation. These…
XZ Plane
In order to understand XZ plane, it's helpful to understand two-dimensional and three-dimensional spaces. To plot a point on a plane, two numbers are needed, and these two numbers in the plane can be represented as an ordered pair (a,b) where a and b are…
Euclidean Geometry
Geometry is the branch of mathematics that deals with flat surfaces like lines, angles, points, two-dimensional figures, etc. In Euclidean geometry, one studies the geometrical shapes that rely on different theorems and axioms. This (pure mathematics) ge…
Lines and Angles
In a two-dimensional plane, a line is simply a figure that joins two points. Usually, lines are used for presenting objects that are straight in shape and have minimal depth or width.
bartleby

Videos

Question
Book Icon
Chapter 5.7, Problem 21PPS
To determine

To graph: the quadratic equation as mentioned in the problem and to write the quadratic equation in vertex form.

Expert Solution & Answer
Check Mark

Answer to Problem 21PPS

The vertex form is (−(x−172)2+10894) and vertex is (172,10894) .

Explanation of Solution

Given information:

The quadratic equation is y=−15x2+255x+3000.

Formula:

For vertex form of quadratic equation we will use y=(x−h)2+k where (h,k) is the vertex.

Calculation:

Consider the quadratic equation y=−15x2+255x+3000⇒−x2+17x−200.

Recall that for vertex form of quadratic equation we will use y=(x−h)2+k where (h,k) is the vertex.

Therefore y=−x2+17x+200⇒−(x2−2*x*172+2894−2894−200)⇒−((x−172)2−10894).

Therefore the vertex form of the quadratic equation y=−15x2+255x+3000 is (−(x−172)2+10894) and the vertex is (172,10894).

Graph:

The graph mentioned below is in real number line of x axis and y axis whose vertex is (172,10894)⇒(8.5,272.25).

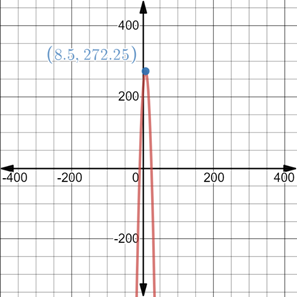
  Algebra 2, Chapter 5.7, Problem 21PPS , additional homework tip 1

Interpretation:

Consider the quadratic equation y=−x2+17x+200.

The mentioned quadratic equation above is in vertex form y=a(x−h)2+k where (h,k) is the vertex.

Therefore the vertex of equation is (172,10894)⇒(8.5,272.25).

We can write the mentioned quadratic equation in the standard form as mentioned below

  y=−x2+17x+200.

Which is comparable to ax2+bx+c where a=−15 which is less than 0.

Since a<0 the direction of graph will be negative side of y axis.

The discriminant of standard form of quadratic equation y=−x2+17x+200 is D=b2−4*a*c⇒d=(17)2−4*(−1)*(200)=289+800=1089.

Here D>0.

Therefore the graph will lie below x axis.

At x=0 the y=−x2+17x+200 will yield y=200.

Therefore graph will cut y axis at (0,200).

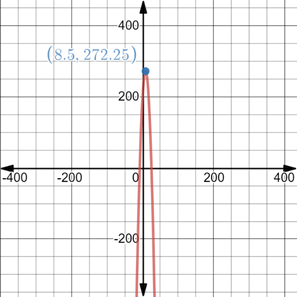
Therefore the graph will be as mentioned below.

. Algebra 2, Chapter 5.7, Problem 21PPS , additional homework tip 2

Therefore the vertex form of the quadratic equation y=−15x2+255x+3000 is (−(x−172)2+10894) and the vertex is (172,10894)⇒(8.5,272.25).

Previous
Chapter 5.7, Problem 20PPS
Next
Chapter 5.7, Problem 22PPS

Chapter 5 Solutions

Algebra 2

Ch. 5.1 - Prob. 1ACYPCh. 5.1 - Prob. 1BCYPCh. 5.1 - Prob. 2ACYPCh. 5.1 - Prob. 2BCYPCh. 5.1 - Prob. 2CCYPCh. 5.1 - Prob. 3ACYPCh. 5.1 - Prob. 3BCYPCh. 5.1 - Prob. 3CCYPCh. 5.1 - Prob. 4CYPCh. 5.1 - Prob. 1CYU
Ch. 5.1 - Prob. 2CYUCh. 5.1 - Prob. 3CYUCh. 5.1 - Prob. 4CYUCh. 5.1 - Prob. 5CYUCh. 5.1 - Prob. 6CYUCh. 5.1 - Prob. 7CYUCh. 5.1 - Prob. 8CYUCh. 5.1 - Prob. 9CYUCh. 5.1 - Prob. 10CYUCh. 5.1 - Prob. 11CYUCh. 5.1 - Prob. 12PPSCh. 5.1 - Prob. 13PPSCh. 5.1 - Prob. 14PPSCh. 5.1 - Prob. 15PPSCh. 5.1 - Prob. 16PPSCh. 5.1 - Prob. 17PPSCh. 5.1 - Prob. 18PPSCh. 5.1 - Prob. 19PPSCh. 5.1 - Prob. 20PPSCh. 5.1 - Prob. 21PPSCh. 5.1 - Prob. 22PPSCh. 5.1 - Prob. 23PPSCh. 5.1 - Prob. 24PPSCh. 5.1 - Prob. 25PPSCh. 5.1 - Prob. 26PPSCh. 5.1 - Prob. 27PPSCh. 5.1 - Prob. 28PPSCh. 5.1 - Prob. 29PPSCh. 5.1 - Prob. 30PPSCh. 5.1 - Prob. 31PPSCh. 5.1 - Prob. 32PPSCh. 5.1 - Prob. 33PPSCh. 5.1 - Prob. 34PPSCh. 5.1 - Prob. 35PPSCh. 5.1 - Prob. 36PPSCh. 5.1 - Prob. 37PPSCh. 5.1 - Prob. 38PPSCh. 5.1 - Prob. 39PPSCh. 5.1 - Prob. 40PPSCh. 5.1 - Prob. 41PPSCh. 5.1 - Prob. 42PPSCh. 5.1 - Prob. 43PPSCh. 5.1 - Prob. 44PPSCh. 5.1 - Prob. 45PPSCh. 5.1 - Prob. 46PPSCh. 5.1 - Prob. 47PPSCh. 5.1 - Prob. 48PPSCh. 5.1 - Prob. 49PPSCh. 5.1 - Prob. 50PPSCh. 5.1 - Prob. 51PPSCh. 5.1 - Prob. 52PPSCh. 5.1 - Prob. 53PPSCh. 5.1 - Prob. 54PPSCh. 5.1 - Prob. 55PPSCh. 5.1 - Prob. 56PPSCh. 5.1 - Prob. 57PPSCh. 5.1 - Prob. 58PPSCh. 5.1 - Prob. 59PPSCh. 5.1 - Prob. 60PPSCh. 5.1 - Prob. 61HPCh. 5.1 - Prob. 62HPCh. 5.1 - Prob. 63HPCh. 5.1 - Prob. 64HPCh. 5.1 - Prob. 65HPCh. 5.1 - Prob. 66HPCh. 5.1 - Prob. 67HPCh. 5.1 - Prob. 68HPCh. 5.1 - Prob. 69HPCh. 5.1 - Prob. 70STPCh. 5.1 - Prob. 71STPCh. 5.1 - Prob. 72STPCh. 5.1 - Prob. 73STPCh. 5.1 - Prob. 74STPCh. 5.1 - Prob. 75STPCh. 5.1 - Prob. 76STPCh. 5.1 - Prob. 77STPCh. 5.1 - Prob. 78STPCh. 5.1 - Prob. 79STPCh. 5.1 - Prob. 80SCh. 5.1 - Prob. 81SCh. 5.1 - Prob. 82SCh. 5.1 - Prob. 1ECh. 5.1 - Prob. 2ECh. 5.1 - Prob. 3ECh. 5.1 - Prob. 4ECh. 5.2 - Prob. 1ACYPCh. 5.2 - Prob. 1BCYPCh. 5.2 - Prob. 2ACYPCh. 5.2 - Prob. 2BCYPCh. 5.2 - Prob. 3CYPCh. 5.2 - Prob. 4CYPCh. 5.2 - Prob. 5CYPCh. 5.2 - Prob. 6CYPCh. 5.2 - Prob. 1CYUCh. 5.2 - Prob. 2CYUCh. 5.2 - Prob. 3CYUCh. 5.2 - Prob. 4CYUCh. 5.2 - Prob. 5CYUCh. 5.2 - Prob. 6CYUCh. 5.2 - Prob. 7CYUCh. 5.2 - Prob. 8CYUCh. 5.2 - Prob. 9CYUCh. 5.2 - Prob. 10CYUCh. 5.2 - Prob. 11CYUCh. 5.2 - Prob. 12CYUCh. 5.2 - Prob. 13CYUCh. 5.2 - Prob. 14PPSCh. 5.2 - Prob. 15PPSCh. 5.2 - Prob. 16PPSCh. 5.2 - Prob. 17PPSCh. 5.2 - Prob. 18PPSCh. 5.2 - Prob. 19PPSCh. 5.2 - Prob. 20PPSCh. 5.2 - Prob. 21PPSCh. 5.2 - Prob. 22PPSCh. 5.2 - Prob. 23PPSCh. 5.2 - Prob. 24PPSCh. 5.2 - Prob. 25PPSCh. 5.2 - Prob. 26PPSCh. 5.2 - Prob. 27PPSCh. 5.2 - Prob. 28PPSCh. 5.2 - Prob. 29PPSCh. 5.2 - Prob. 30PPSCh. 5.2 - Prob. 31PPSCh. 5.2 - Prob. 32PPSCh. 5.2 - Prob. 33PPSCh. 5.2 - Prob. 34PPSCh. 5.2 - Prob. 35PPSCh. 5.2 - Prob. 36PPSCh. 5.2 - Prob. 37PPSCh. 5.2 - Prob. 38PPSCh. 5.2 - Prob. 39PPSCh. 5.2 - Prob. 40PPSCh. 5.2 - Prob. 41PPSCh. 5.2 - Prob. 42PPSCh. 5.2 - Prob. 43PPSCh. 5.2 - Prob. 44PPSCh. 5.2 - Prob. 45PPSCh. 5.2 - Prob. 46PPSCh. 5.2 - Prob. 47PPSCh. 5.2 - Prob. 48PPSCh. 5.2 - Prob. 49PPSCh. 5.2 - Prob. 50PPSCh. 5.2 - Prob. 51PPSCh. 5.2 - Prob. 52HPCh. 5.2 - Prob. 53HPCh. 5.2 - Prob. 54HPCh. 5.2 - Prob. 55HPCh. 5.2 - Prob. 56HPCh. 5.2 - Prob. 57HPCh. 5.2 - Prob. 58HPCh. 5.2 - Prob. 59HPCh. 5.2 - Prob. 60HPCh. 5.2 - Prob. 61STPCh. 5.2 - Prob. 62STPCh. 5.2 - Prob. 63STPCh. 5.2 - Prob. 64STPCh. 5.2 - Prob. 65STPCh. 5.2 - Prob. 66STPCh. 5.2 - Prob. 67STPCh. 5.2 - Prob. 68STPCh. 5.2 - Prob. 69STPCh. 5.2 - Prob. 70STPCh. 5.2 - Prob. 71STPCh. 5.2 - Prob. 72STPCh. 5.2 - Prob. 73STPCh. 5.2 - Prob. 74SCh. 5.2 - Prob. 75SCh. 5.2 - Prob. 76SCh. 5.2 - Prob. 1ECh. 5.2 - Prob. 2ECh. 5.2 - Prob. 3ECh. 5.2 - Prob. 4ECh. 5.2 - Prob. 5ECh. 5.2 - Prob. 6ECh. 5.2 - Prob. 7ECh. 5.2 - Prob. 8ECh. 5.2 - Prob. 9ECh. 5.2 - Prob. 10ECh. 5.2 - Prob. 11ECh. 5.2 - Prob. 12ECh. 5.3 - Prob. 1CYPCh. 5.3 - Prob. 2ACYPCh. 5.3 - Prob. 2BCYPCh. 5.3 - Prob. 2CCYPCh. 5.3 - Prob. 3ACYPCh. 5.3 - Prob. 3BCYPCh. 5.3 - Prob. 3CCYPCh. 5.3 - Prob. 4ACYPCh. 5.3 - Prob. 4BCYPCh. 5.3 - Prob. 4CCYPCh. 5.3 - Prob. 4DCYPCh. 5.3 - Prob. 5CYPCh. 5.3 - Prob. 1CYUCh. 5.3 - Prob. 2CYUCh. 5.3 - Prob. 3CYUCh. 5.3 - Prob. 4CYUCh. 5.3 - Prob. 5CYUCh. 5.3 - Prob. 6CYUCh. 5.3 - Prob. 7CYUCh. 5.3 - Prob. 8CYUCh. 5.3 - Prob. 9CYUCh. 5.3 - Prob. 10CYUCh. 5.3 - Prob. 11CYUCh. 5.3 - Prob. 12CYUCh. 5.3 - Prob. 13CYUCh. 5.3 - Prob. 14CYUCh. 5.3 - Prob. 15CYUCh. 5.3 - Prob. 16CYUCh. 5.3 - Prob. 17PPSCh. 5.3 - Prob. 18PPSCh. 5.3 - Prob. 19PPSCh. 5.3 - Prob. 20PPSCh. 5.3 - Prob. 21PPSCh. 5.3 - Prob. 22PPSCh. 5.3 - Prob. 23PPSCh. 5.3 - Prob. 24PPSCh. 5.3 - Prob. 25PPSCh. 5.3 - Prob. 26PPSCh. 5.3 - Prob. 27PPSCh. 5.3 - Prob. 28PPSCh. 5.3 - Prob. 29PPSCh. 5.3 - Prob. 30PPSCh. 5.3 - Prob. 31PPSCh. 5.3 - Prob. 32PPSCh. 5.3 - Prob. 33PPSCh. 5.3 - Prob. 34PPSCh. 5.3 - Prob. 35PPSCh. 5.3 - Prob. 36PPSCh. 5.3 - Prob. 37PPSCh. 5.3 - Prob. 38PPSCh. 5.3 - Prob. 39PPSCh. 5.3 - Prob. 40PPSCh. 5.3 - Prob. 41PPSCh. 5.3 - Prob. 42PPSCh. 5.3 - Prob. 43PPSCh. 5.3 - Prob. 44PPSCh. 5.3 - Prob. 45PPSCh. 5.3 - Prob. 46PPSCh. 5.3 - Prob. 47PPSCh. 5.3 - Prob. 48PPSCh. 5.3 - Prob. 49PPSCh. 5.3 - Prob. 50PPSCh. 5.3 - Prob. 51PPSCh. 5.3 - Prob. 52PPSCh. 5.3 - Prob. 53PPSCh. 5.3 - Prob. 54PPSCh. 5.3 - Prob. 55PPSCh. 5.3 - Prob. 56PPSCh. 5.3 - Prob. 57PPSCh. 5.3 - Prob. 58PPSCh. 5.3 - Prob. 59PPSCh. 5.3 - Prob. 60PPSCh. 5.3 - Prob. 61PPSCh. 5.3 - Prob. 62PPSCh. 5.3 - Prob. 63PPSCh. 5.3 - Prob. 64PPSCh. 5.3 - Prob. 65PPSCh. 5.3 - Prob. 66PPSCh. 5.3 - Prob. 67PPSCh. 5.3 - Prob. 68PPSCh. 5.3 - Prob. 69PPSCh. 5.3 - Prob. 70PPSCh. 5.3 - Prob. 71PPSCh. 5.3 - Prob. 72PPSCh. 5.3 - Prob. 73PPSCh. 5.3 - Prob. 74PPSCh. 5.3 - Prob. 75PPSCh. 5.3 - Prob. 76PPSCh. 5.3 - Prob. 77PPSCh. 5.3 - Prob. 78PPSCh. 5.3 - Prob. 79HPCh. 5.3 - Prob. 80HPCh. 5.3 - Prob. 81HPCh. 5.3 - Prob. 82HPCh. 5.3 - Prob. 83HPCh. 5.3 - Prob. 84HPCh. 5.3 - Prob. 85HPCh. 5.3 - Prob. 86HPCh. 5.3 - Prob. 87HPCh. 5.3 - Prob. 88HPCh. 5.3 - Prob. 89HPCh. 5.3 - Prob. 90HPCh. 5.3 - Prob. 91STPCh. 5.3 - Prob. 92STPCh. 5.3 - Prob. 93STPCh. 5.3 - Prob. 94STPCh. 5.3 - Prob. 95STPCh. 5.3 - Prob. 96STPCh. 5.3 - Prob. 97STPCh. 5.3 - Prob. 98SCh. 5.3 - Prob. 99SCh. 5.3 - Prob. 100SCh. 5.4 - Prob. 1ACYPCh. 5.4 - Prob. 1BCYPCh. 5.4 - Prob. 2ACYPCh. 5.4 - Prob. 2BCYPCh. 5.4 - Prob. 2CCYPCh. 5.4 - Prob. 3ACYPCh. 5.4 - Prob. 3BCYPCh. 5.4 - Prob. 4CYPCh. 5.4 - Prob. 5ACYPCh. 5.4 - Prob. 5BCYPCh. 5.4 - Prob. 6CYPCh. 5.4 - Prob. 7ACYPCh. 5.4 - Prob. 7BCYPCh. 5.4 - Prob. 1CYUCh. 5.4 - Prob. 2CYUCh. 5.4 - Prob. 3CYUCh. 5.4 - Prob. 4CYUCh. 5.4 - Prob. 5CYUCh. 5.4 - Prob. 6CYUCh. 5.4 - Prob. 7CYUCh. 5.4 - Prob. 8CYUCh. 5.4 - Prob. 9CYUCh. 5.4 - Prob. 10CYUCh. 5.4 - Prob. 11CYUCh. 5.4 - Prob. 12CYUCh. 5.4 - Prob. 13CYUCh. 5.4 - Prob. 14CYUCh. 5.4 - Prob. 15CYUCh. 5.4 - Prob. 16CYUCh. 5.4 - Prob. 17CYUCh. 5.4 - Prob. 18PPSCh. 5.4 - Prob. 19PPSCh. 5.4 - Prob. 20PPSCh. 5.4 - Prob. 21PPSCh. 5.4 - Prob. 22PPSCh. 5.4 - Prob. 23PPSCh. 5.4 - Prob. 24PPSCh. 5.4 - Prob. 25PPSCh. 5.4 - Prob. 26PPSCh. 5.4 - Prob. 27PPSCh. 5.4 - Prob. 28PPSCh. 5.4 - Prob. 29PPSCh. 5.4 - Prob. 30PPSCh. 5.4 - Prob. 31PPSCh. 5.4 - Prob. 32PPSCh. 5.4 - Prob. 33PPSCh. 5.4 - Prob. 34PPSCh. 5.4 - Prob. 35PPSCh. 5.4 - Prob. 36PPSCh. 5.4 - Prob. 37PPSCh. 5.4 - Prob. 38PPSCh. 5.4 - Prob. 39PPSCh. 5.4 - Prob. 40PPSCh. 5.4 - Prob. 41PPSCh. 5.4 - Prob. 42PPSCh. 5.4 - Prob. 43PPSCh. 5.4 - Prob. 44PPSCh. 5.4 - Prob. 45PPSCh. 5.4 - Prob. 46PPSCh. 5.4 - Prob. 47PPSCh. 5.4 - Prob. 48PPSCh. 5.4 - Prob. 49PPSCh. 5.4 - Prob. 50PPSCh. 5.4 - Prob. 51PPSCh. 5.4 - Prob. 52PPSCh. 5.4 - Prob. 53PPSCh. 5.4 - Prob. 54PPSCh. 5.4 - Prob. 55PPSCh. 5.4 - Prob. 56PPSCh. 5.4 - Prob. 57PPSCh. 5.4 - Prob. 58PPSCh. 5.4 - Prob. 59PPSCh. 5.4 - Prob. 60PPSCh. 5.4 - Prob. 61PPSCh. 5.4 - Prob. 62PPSCh. 5.4 - Prob. 63PPSCh. 5.4 - Prob. 64PPSCh. 5.4 - Prob. 65PPSCh. 5.4 - Prob. 66HPCh. 5.4 - Prob. 67HPCh. 5.4 - Prob. 68HPCh. 5.4 - Prob. 69HPCh. 5.4 - Prob. 70HPCh. 5.4 - Prob. 71HPCh. 5.4 - Prob. 72HPCh. 5.4 - Prob. 73HPCh. 5.4 - Prob. 74HPCh. 5.4 - Prob. 75STPCh. 5.4 - Prob. 76STPCh. 5.4 - Prob. 77STPCh. 5.4 - Prob. 78STPCh. 5.4 - Prob. 79STPCh. 5.4 - Prob. 80STPCh. 5.4 - Prob. 81STPCh. 5.4 - Prob. 82STPCh. 5.4 - Prob. 83STPCh. 5.4 - Prob. 84SCh. 5.4 - Prob. 85SCh. 5.4 - Prob. 86SCh. 5.4 - Prob. 87SCh. 5.4 - Prob. 88SCh. 5.4 - Prob. 89SCh. 5.5 - Prob. 1ACYPCh. 5.5 - Prob. 1BCYPCh. 5.5 - Prob. 2ACYPCh. 5.5 - Prob. 2BCYPCh. 5.5 - Prob. 3CYPCh. 5.5 - Prob. 4ACYPCh. 5.5 - Prob. 4BCYPCh. 5.5 - Prob. 5ACYPCh. 5.5 - Prob. 5BCYPCh. 5.5 - Prob. 6ACYPCh. 5.5 - Prob. 6BCYPCh. 5.5 - Prob. 1CYUCh. 5.5 - Prob. 2CYUCh. 5.5 - Prob. 3CYUCh. 5.5 - Prob. 4CYUCh. 5.5 - Prob. 5CYUCh. 5.5 - Prob. 6CYUCh. 5.5 - Prob. 7CYUCh. 5.5 - Prob. 8CYUCh. 5.5 - Prob. 9CYUCh. 5.5 - Prob. 10CYUCh. 5.5 - Prob. 11CYUCh. 5.5 - Prob. 12CYUCh. 5.5 - Prob. 13CYUCh. 5.5 - Prob. 14PPSCh. 5.5 - Prob. 15PPSCh. 5.5 - Prob. 16PPSCh. 5.5 - Prob. 17PPSCh. 5.5 - Prob. 18PPSCh. 5.5 - Prob. 19PPSCh. 5.5 - Prob. 20PPSCh. 5.5 - Prob. 21PPSCh. 5.5 - Prob. 22PPSCh. 5.5 - Prob. 23PPSCh. 5.5 - Prob. 24PPSCh. 5.5 - Prob. 25PPSCh. 5.5 - Prob. 26PPSCh. 5.5 - Prob. 27PPSCh. 5.5 - Prob. 28PPSCh. 5.5 - Prob. 29PPSCh. 5.5 - Prob. 30PPSCh. 5.5 - Prob. 31PPSCh. 5.5 - Prob. 32PPSCh. 5.5 - Prob. 33PPSCh. 5.5 - Prob. 34PPSCh. 5.5 - Prob. 35PPSCh. 5.5 - Prob. 36PPSCh. 5.5 - Prob. 37PPSCh. 5.5 - Prob. 38PPSCh. 5.5 - Prob. 39PPSCh. 5.5 - Prob. 40PPSCh. 5.5 - Prob. 41PPSCh. 5.5 - Prob. 42PPSCh. 5.5 - Prob. 43PPSCh. 5.5 - Prob. 44PPSCh. 5.5 - Prob. 45PPSCh. 5.5 - Prob. 46PPSCh. 5.5 - Prob. 47PPSCh. 5.5 - Prob. 48PPSCh. 5.5 - Prob. 49PPSCh. 5.5 - Prob. 50PPSCh. 5.5 - Prob. 51PPSCh. 5.5 - Prob. 52PPSCh. 5.5 - Prob. 53PPSCh. 5.5 - Prob. 54PPSCh. 5.5 - Prob. 55PPSCh. 5.5 - Prob. 56PPSCh. 5.5 - Prob. 57PPSCh. 5.5 - Prob. 58HPCh. 5.5 - Prob. 59HPCh. 5.5 - Prob. 60HPCh. 5.5 - Prob. 61HPCh. 5.5 - Prob. 62HPCh. 5.5 - Prob. 63HPCh. 5.5 - Prob. 64HPCh. 5.5 - Prob. 65HPCh. 5.5 - Prob. 66HPCh. 5.5 - Prob. 67STPCh. 5.5 - Prob. 68STPCh. 5.5 - Prob. 69STPCh. 5.5 - Prob. 70STPCh. 5.5 - Prob. 71STPCh. 5.5 - Prob. 72STPCh. 5.5 - Prob. 73STPCh. 5.5 - Prob. 74STPCh. 5.5 - Prob. 75STPCh. 5.5 - Prob. 76STPCh. 5.5 - Prob. 77STPCh. 5.5 - Prob. 78STPCh. 5.5 - Prob. 79STPCh. 5.5 - Prob. 80STPCh. 5.5 - Prob. 81SCh. 5.5 - Prob. 82SCh. 5.5 - Prob. 83SCh. 5.5 - Prob. 1ECh. 5.5 - Prob. 2ECh. 5.5 - Prob. 3ECh. 5.5 - Prob. 4ECh. 5.5 - Prob. 5ECh. 5.5 - Prob. 6ECh. 5.5 - Prob. 7ECh. 5.5 - Prob. 8ECh. 5.5 - Prob. 9ECh. 5.6 - Prob. 1ACYPCh. 5.6 - Prob. 1BCYPCh. 5.6 - Prob. 2ACYPCh. 5.6 - Prob. 2BCYPCh. 5.6 - Prob. 3ACYPCh. 5.6 - Prob. 3BCYPCh. 5.6 - Prob. 4ACYPCh. 5.6 - Prob. 4BCYPCh. 5.6 - Prob. 5ACYPCh. 5.6 - Prob. 5BCYPCh. 5.6 - Prob. 1CYUCh. 5.6 - Prob. 2CYUCh. 5.6 - Prob. 3CYUCh. 5.6 - Prob. 4CYUCh. 5.6 - Prob. 5CYUCh. 5.6 - Prob. 6CYUCh. 5.6 - Prob. 7CYUCh. 5.6 - Prob. 8CYUCh. 5.6 - Prob. 9CYUCh. 5.6 - Prob. 10CYUCh. 5.6 - Prob. 11CYUCh. 5.6 - Prob. 12CYUCh. 5.6 - Prob. 13CYUCh. 5.6 - Prob. 14PPSCh. 5.6 - Prob. 15PPSCh. 5.6 - Prob. 16PPSCh. 5.6 - Prob. 17PPSCh. 5.6 - Prob. 18PPSCh. 5.6 - Prob. 19PPSCh. 5.6 - Prob. 20PPSCh. 5.6 - Prob. 21PPSCh. 5.6 - Prob. 22PPSCh. 5.6 - Prob. 23PPSCh. 5.6 - Prob. 24PPSCh. 5.6 - Prob. 25PPSCh. 5.6 - Prob. 26PPSCh. 5.6 - Prob. 27PPSCh. 5.6 - Prob. 28PPSCh. 5.6 - Prob. 29PPSCh. 5.6 - Prob. 30PPSCh. 5.6 - Prob. 31PPSCh. 5.6 - Prob. 32PPSCh. 5.6 - Prob. 33PPSCh. 5.6 - Prob. 34PPSCh. 5.6 - Prob. 35PPSCh. 5.6 - Prob. 36PPSCh. 5.6 - Prob. 37PPSCh. 5.6 - Prob. 38PPSCh. 5.6 - Prob. 39PPSCh. 5.6 - Prob. 40PPSCh. 5.6 - Prob. 41PPSCh. 5.6 - Prob. 42PPSCh. 5.6 - Prob. 43HPCh. 5.6 - Prob. 44HPCh. 5.6 - Prob. 45HPCh. 5.6 - Prob. 46HPCh. 5.6 - Prob. 47HPCh. 5.6 - Prob. 48HPCh. 5.6 - Prob. 49HPCh. 5.6 - Prob. 50HPCh. 5.6 - Prob. 51HPCh. 5.6 - Prob. 52HPCh. 5.6 - Prob. 53STPCh. 5.6 - Prob. 54STPCh. 5.6 - Prob. 55STPCh. 5.6 - Prob. 56STPCh. 5.6 - Prob. 57STPCh. 5.6 - Prob. 58STPCh. 5.6 - Prob. 59STPCh. 5.6 - Prob. 60STPCh. 5.6 - Prob. 61SCh. 5.6 - Prob. 62SCh. 5.6 - Prob. 63SCh. 5.6 - Prob. 1ECh. 5.6 - Prob. 2ECh. 5.6 - Prob. 3ECh. 5.6 - Prob. 4ECh. 5.6 - Prob. 5ECh. 5.6 - Prob. 6ECh. 5.6 - Prob. 7ECh. 5.6 - Prob. 8ECh. 5.6 - Prob. 9ECh. 5.6 - Prob. 10ECh. 5.7 - Prob. 1ACYPCh. 5.7 - Prob. 1BCYPCh. 5.7 - Prob. 2CYPCh. 5.7 - Prob. 3ACYPCh. 5.7 - Prob. 3BCYPCh. 5.7 - Prob. 1CYUCh. 5.7 - Prob. 2CYUCh. 5.7 - Prob. 3CYUCh. 5.7 - Prob. 4CYUCh. 5.7 - Prob. 5CYUCh. 5.7 - Prob. 6CYUCh. 5.7 - Prob. 7CYUCh. 5.7 - Prob. 8PPSCh. 5.7 - Prob. 9PPSCh. 5.7 - Prob. 10PPSCh. 5.7 - Prob. 11PPSCh. 5.7 - Prob. 12PPSCh. 5.7 - Prob. 13PPSCh. 5.7 - Prob. 14PPSCh. 5.7 - Prob. 15PPSCh. 5.7 - Prob. 16PPSCh. 5.7 - Prob. 17PPSCh. 5.7 - Prob. 18PPSCh. 5.7 - Prob. 19PPSCh. 5.7 - Prob. 20PPSCh. 5.7 - Prob. 21PPSCh. 5.7 - Prob. 22PPSCh. 5.7 - Prob. 23PPSCh. 5.7 - Prob. 24PPSCh. 5.7 - Prob. 25PPSCh. 5.7 - Prob. 26PPSCh. 5.7 - Prob. 27PPSCh. 5.7 - Prob. 28PPSCh. 5.7 - Prob. 29PPSCh. 5.7 - Prob. 30PPSCh. 5.7 - Prob. 31PPSCh. 5.7 - Prob. 32PPSCh. 5.7 - Prob. 33PPSCh. 5.7 - Prob. 34PPSCh. 5.7 - Prob. 35PPSCh. 5.7 - Prob. 36PPSCh. 5.7 - Prob. 37PPSCh. 5.7 - Prob. 38PPSCh. 5.7 - Prob. 39PPSCh. 5.7 - Prob. 40PPSCh. 5.7 - Prob. 41PPSCh. 5.7 - Prob. 42PPSCh. 5.7 - Prob. 43PPSCh. 5.7 - Prob. 44PPSCh. 5.7 - Prob. 45PPSCh. 5.7 - Prob. 46PPSCh. 5.7 - Prob. 47PPSCh. 5.7 - Prob. 48HPCh. 5.7 - Prob. 49HPCh. 5.7 - Prob. 50HPCh. 5.7 - Prob. 51HPCh. 5.7 - Prob. 52HPCh. 5.7 - Prob. 53HPCh. 5.7 - Prob. 54HPCh. 5.7 - Prob. 55HPCh. 5.7 - Prob. 56HPCh. 5.7 - Prob. 57STPCh. 5.7 - Prob. 58STPCh. 5.7 - Prob. 59STPCh. 5.7 - Prob. 60STPCh. 5.7 - Prob. 61STPCh. 5.7 - Prob. 62STPCh. 5.7 - Prob. 63STPCh. 5.7 - Prob. 64STPCh. 5.7 - Prob. 65STPCh. 5.7 - Prob. 66STPCh. 5.7 - Prob. 67STPCh. 5.7 - Prob. 68STPCh. 5.7 - Prob. 69STPCh. 5.7 - Prob. 70STPCh. 5.7 - Prob. 71STPCh. 5.7 - Prob. 72STPCh. 5.7 - Prob. 73STPCh. 5.7 - Prob. 74SCh. 5.7 - Prob. 75SCh. 5.7 - Prob. 76SCh. 5.7 - Prob. 1ECh. 5.7 - Prob. 2ECh. 5.7 - Prob. 3ECh. 5.7 - Prob. 4ECh. 5.8 - Prob. 1ACYPCh. 5.8 - Prob. 1BCYPCh. 5.8 - Prob. 2ACYPCh. 5.8 - Prob. 2BCYPCh. 5.8 - Prob. 3ACYPCh. 5.8 - Prob. 3BCYPCh. 5.8 - Prob. 4CYPCh. 5.8 - Prob. 5ACYPCh. 5.8 - Prob. 5BCYPCh. 5.8 - Prob. 1CYUCh. 5.8 - Prob. 2CYUCh. 5.8 - Prob. 3CYUCh. 5.8 - Prob. 4CYUCh. 5.8 - Prob. 5CYUCh. 5.8 - Prob. 6CYUCh. 5.8 - Prob. 7CYUCh. 5.8 - Prob. 8CYUCh. 5.8 - Prob. 9CYUCh. 5.8 - Prob. 10CYUCh. 5.8 - Prob. 11CYUCh. 5.8 - Prob. 12CYUCh. 5.8 - Prob. 13PPSCh. 5.8 - Prob. 14PPSCh. 5.8 - Prob. 15PPSCh. 5.8 - Prob. 16PPSCh. 5.8 - Prob. 17PPSCh. 5.8 - Prob. 18PPSCh. 5.8 - Prob. 19PPSCh. 5.8 - Prob. 20PPSCh. 5.8 - Prob. 21PPSCh. 5.8 - Prob. 22PPSCh. 5.8 - Prob. 23PPSCh. 5.8 - Prob. 24PPSCh. 5.8 - Prob. 25PPSCh. 5.8 - Prob. 26PPSCh. 5.8 - Prob. 27PPSCh. 5.8 - Prob. 28PPSCh. 5.8 - Prob. 29PPSCh. 5.8 - Prob. 30PPSCh. 5.8 - Prob. 31PPSCh. 5.8 - Prob. 32PPSCh. 5.8 - Prob. 33PPSCh. 5.8 - Prob. 34PPSCh. 5.8 - Prob. 35PPSCh. 5.8 - Prob. 36PPSCh. 5.8 - Prob. 37PPSCh. 5.8 - Prob. 38PPSCh. 5.8 - Prob. 39PPSCh. 5.8 - Prob. 40PPSCh. 5.8 - Prob. 41PPSCh. 5.8 - Prob. 42PPSCh. 5.8 - Prob. 43PPSCh. 5.8 - Prob. 44PPSCh. 5.8 - Prob. 45PPSCh. 5.8 - Prob. 46PPSCh. 5.8 - Prob. 47PPSCh. 5.8 - Prob. 48PPSCh. 5.8 - Prob. 49PPSCh. 5.8 - Prob. 50PPSCh. 5.8 - Prob. 51PPSCh. 5.8 - Prob. 52PPSCh. 5.8 - Prob. 53PPSCh. 5.8 - Prob. 54PPSCh. 5.8 - Prob. 55PPSCh. 5.8 - Prob. 56PPSCh. 5.8 - Prob. 57HPCh. 5.8 - Prob. 58HPCh. 5.8 - Prob. 59HPCh. 5.8 - Prob. 60HPCh. 5.8 - Prob. 61HPCh. 5.8 - Prob. 62HPCh. 5.8 - Prob. 63HPCh. 5.8 - Prob. 64HPCh. 5.8 - Prob. 65HPCh. 5.8 - Prob. 66HPCh. 5.8 - Prob. 67STPCh. 5.8 - Prob. 68STPCh. 5.8 - Prob. 69STPCh. 5.8 - Prob. 70STPCh. 5.8 - Prob. 71STPCh. 5.8 - Prob. 72STPCh. 5.8 - Prob. 73STPCh. 5.8 - Prob. 74STPCh. 5.8 - Prob. 75STPCh. 5.8 - Prob. 76STPCh. 5.8 - Prob. 77SCh. 5.8 - Prob. 78SCh. 5.8 - Prob. 79SCh. 5.8 - Prob. 80SCh. 5.8 - Prob. 81SCh. 5.8 - Prob. 82SCh. 5 - Prob. 1QCCh. 5 - Prob. 2QCCh. 5 - Prob. 3QCCh. 5 - Prob. 4QCCh. 5 - Prob. 5QCCh. 5 - Prob. 6QCCh. 5 - Prob. 7QCCh. 5 - Prob. 8QCCh. 5 - Prob. 9QCCh. 5 - Prob. 10QCCh. 5 - Prob. 11QCCh. 5 - Prob. 12QCCh. 5 - Prob. 13QCCh. 5 - Prob. 14QCCh. 5 - Prob. 15QCCh. 5 - Prob. 1MCQCh. 5 - Prob. 2MCQCh. 5 - Prob. 3MCQCh. 5 - Prob. 4MCQCh. 5 - Prob. 5MCQCh. 5 - Prob. 6MCQCh. 5 - Prob. 7MCQCh. 5 - Prob. 8MCQCh. 5 - Prob. 9MCQCh. 5 - Prob. 10MCQCh. 5 - Prob. 11MCQCh. 5 - Prob. 12MCQCh. 5 - Prob. 13MCQCh. 5 - Prob. 14MCQCh. 5 - Prob. 15MCQCh. 5 - Prob. 16MCQCh. 5 - Prob. 17MCQCh. 5 - Prob. 18MCQCh. 5 - Prob. 19MCQCh. 5 - Prob. 20MCQCh. 5 - Prob. 21MCQCh. 5 - Prob. 22MCQCh. 5 - Prob. 23MCQCh. 5 - Prob. 1SGRCh. 5 - Prob. 2SGRCh. 5 - Prob. 3SGRCh. 5 - Prob. 4SGRCh. 5 - Prob. 5SGRCh. 5 - Prob. 6SGRCh. 5 - Prob. 7SGRCh. 5 - Prob. 8SGRCh. 5 - Prob. 9SGRCh. 5 - Prob. 10SGRCh. 5 - Prob. 11SGRCh. 5 - Prob. 12SGRCh. 5 - Prob. 13SGRCh. 5 - Prob. 14SGRCh. 5 - Prob. 15SGRCh. 5 - Prob. 16SGRCh. 5 - Prob. 17SGRCh. 5 - Prob. 18SGRCh. 5 - Prob. 19SGRCh. 5 - Prob. 20SGRCh. 5 - Prob. 21SGRCh. 5 - Prob. 22SGRCh. 5 - Prob. 23SGRCh. 5 - Prob. 24SGRCh. 5 - Prob. 25SGRCh. 5 - Prob. 26SGRCh. 5 - Prob. 27SGRCh. 5 - Prob. 28SGRCh. 5 - Prob. 29SGRCh. 5 - Prob. 30SGRCh. 5 - Prob. 31SGRCh. 5 - Prob. 32SGRCh. 5 - Prob. 33SGRCh. 5 - Prob. 34SGRCh. 5 - Prob. 35SGRCh. 5 - Prob. 36SGRCh. 5 - Prob. 37SGRCh. 5 - Prob. 38SGRCh. 5 - Prob. 39SGRCh. 5 - Prob. 40SGRCh. 5 - Prob. 41SGRCh. 5 - Prob. 42SGRCh. 5 - Prob. 43SGRCh. 5 - Prob. 44SGRCh. 5 - Prob. 45SGRCh. 5 - Prob. 46SGRCh. 5 - Prob. 47SGRCh. 5 - Prob. 48SGRCh. 5 - Prob. 49SGRCh. 5 - Prob. 50SGRCh. 5 - Prob. 51SGRCh. 5 - Prob. 52SGRCh. 5 - Prob. 53SGRCh. 5 - Prob. 54SGRCh. 5 - Prob. 55SGRCh. 5 - Prob. 56SGRCh. 5 - Prob. 57SGRCh. 5 - Prob. 58SGRCh. 5 - Prob. 59SGRCh. 5 - Prob. 60SGRCh. 5 - Prob. 61SGRCh. 5 - Prob. 62SGRCh. 5 - Prob. 63SGRCh. 5 - Prob. 64SGRCh. 5 - Prob. 65SGRCh. 5 - Prob. 66SGRCh. 5 - Prob. 67SGRCh. 5 - Prob. 68SGRCh. 5 - Prob. 69SGRCh. 5 - Prob. 70SGRCh. 5 - Prob. 71SGRCh. 5 - Prob. 72SGRCh. 5 - Prob. 73SGRCh. 5 - Prob. 1PTCh. 5 - Prob. 2PTCh. 5 - Prob. 3PTCh. 5 - Prob. 4PTCh. 5 - Prob. 5PTCh. 5 - Prob. 6PTCh. 5 - Prob. 7PTCh. 5 - Prob. 8PTCh. 5 - Prob. 9PTCh. 5 - Prob. 10PTCh. 5 - Prob. 11PTCh. 5 - Prob. 12PTCh. 5 - Prob. 13PTCh. 5 - Prob. 14PTCh. 5 - Prob. 15PTCh. 5 - Prob. 16PTCh. 5 - Prob. 17PTCh. 5 - Prob. 18PTCh. 5 - Prob. 19PTCh. 5 - Prob. 20PTCh. 5 - Prob. 21PTCh. 5 - Prob. 22PTCh. 5 - Prob. 23PTCh. 5 - Prob. 24PTCh. 5 - Prob. 25PTCh. 5 - Prob. 26PTCh. 5 - Prob. 1ECh. 5 - Prob. 2ECh. 5 - Prob. 3ECh. 5 - Prob. 4ECh. 5 - Prob. 5ECh. 5 - Prob. 6ECh. 5 - Prob. 1STPCh. 5 - Prob. 2STPCh. 5 - Prob. 3STPCh. 5 - Prob. 4STPCh. 5 - Prob. 5STPCh. 5 - Prob. 6STPCh. 5 - Prob. 7STPCh. 5 - Prob. 8STPCh. 5 - Prob. 9STPCh. 5 - Prob. 10STPCh. 5 - Prob. 11STPCh. 5 - Prob. 12STPCh. 5 - Prob. 13STPCh. 5 - Prob. 14STPCh. 5 - Prob. 15STPCh. 5 - Prob. 16STP

Additional Math Textbook Solutions

Find more solutions based on key concepts
In the following exercises, simplify. 9+12/3+4

PREALGEBRA

Fill in each blanks so that the resulting statement is true. Any set of ordered pairs is called a/an _______. T...

College Algebra

For the following exercises, factor the polynomial. 60.4r(2r1)235(2r1)12

College Algebra

Explain why a 2 2 matrix can have at most two distinct eigenvalues. Explain why an n n matrix can have at mos...

Linear Algebra and Its Applications (5th Edition)

In the following exercises, solve by completing the square. 88. (y+9)(y+7)=79

Elementary Algebra

Fill in each blanks so that the resulting statement is true. Any set of ordered pairs is called a/an _______. T...

College Algebra (7th Edition)

Knowledge Booster
Background pattern image
Algebra
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, algebra and related others by exploring similar questions and additional content below.
Recommended textbooks for you
Text book image
Algebra and Trigonometry (6th Edition)
Algebra
ISBN:9780134463216
Author:Robert F. Blitzer
Publisher:PEARSON
Text book image
Contemporary Abstract Algebra
Algebra
ISBN:9781305657960
Author:Joseph Gallian
Publisher:Cengage Learning
Text book image
Linear Algebra: A Modern Introduction
Algebra
ISBN:9781285463247
Author:David Poole
Publisher:Cengage Learning
Text book image
Algebra And Trigonometry (11th Edition)
Algebra
ISBN:9780135163078
Author:Michael Sullivan
Publisher:PEARSON
Text book image
Introduction to Linear Algebra, Fifth Edition
Algebra
ISBN:9780980232776
Author:Gilbert Strang
Publisher:Wellesley-Cambridge Press
Text book image
College Algebra (Collegiate Math)
Algebra
ISBN:9780077836344
Author:Julie Miller, Donna Gerken
Publisher:McGraw-Hill Education
SEE MORE TEXTBOOKS
Points, Lines, Planes, Segments, & Rays - Collinear vs Coplanar Points - Geometry; Author: The Organic Chemistry Tutor;https://www.youtube.com/watch?v=dDWjhRfBsKM;License: Standard YouTube License, CC-BY
Naming Points, Lines, and Planes; Author: Florida PASS Program;https://www.youtube.com/watch?v=F-LxiLSSaLg;License: Standard YouTube License, CC-BY