
Find a transformation
Want to see the full answer?
Check out a sample textbook solution
Chapter 14 Solutions
EBK CALCULUS EARLY TRANSCENDENTALS SING
Additional Math Textbook Solutions
Algebra and Trigonometry (6th Edition)
Calculus: Early Transcendentals (2nd Edition)
Intro Stats, Books a la Carte Edition (5th Edition)
A Problem Solving Approach To Mathematics For Elementary School Teachers (13th Edition)
Elementary Statistics (13th Edition)
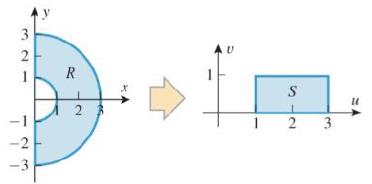
- Integrate (x + y) dA, where R = {(x, y):0 < x < 2, x < y < x + 4}. Use the transformation x = 2u and y = 4v + 2u. Hint: Sketch the given region R and then the new region in the uv plane.arrow_forwardPlz answer correctly asaparrow_forwardEvaluate exp{}dA where R is the region in the ry-plane bounded by the trapezoid with vertices (0, 1), (0, 2), (2,0), and (1,0) by a suitable change of variables.arrow_forward
- Let D be the triangular region in the uv-plane with vertices (0, 1), (4, 1), (1, 3) and letG(u, v) = (u − v, 2v).Sketch D in the uv-plane and G(D) in the xy-plane.Find the area of G(D) by using Jac(G)arrow_forward3. Use the transformation u = 4, v = xy to find 1 /| xy³ dA over the region R in the first quadrant enclosed by y = x, y= 3x, xy = 1, xy = 4.arrow_forwardSketch a diagram of the linear map G that maps the rectangle R = [0, 1]×[0, 1] inthe uv-plane to the parallelogram P in the xy-plane with vertices (0, 0),(2, 2),(1, 4),and (3, 6). Draw the rectangle in the uv-plane on the left and its image P in thexy-plane on the right, with an arrow labeled G in the middle. Find the map G(u, v) = (x(u, v), y(u, v)) explicitly. Set up, but do not evaluate an integral in u and v to calculate Z ZRxy dA.arrow_forward
- Use the cross product to find the area of the portion of the plane defined by z = x + y which lies above the square [0, 1] x [0, 1] in the xy-plane. Draw a picture.arrow_forwardi need the answer quicklyarrow_forwardlet T(x,y) = (2x-y, xy) a) What is T(0,0) ? b) Is T a linear transformation? Explain c) Find the image under T of the region R bounded y=1/x ; y=2/x ; y= 2x; y=2x-1 d) ∫∫ R ( 2x+y/xy)dA where R is the same as in part (c)arrow_forward
- Sketch the surface of f(x,y)arrow_forwardFind the global min and max of the provided function on the provided region R: f(x,y) = x - y - xy on the triangle R with vertices (0,0), (0,2), and (4,0)arrow_forwardLet R be the region bounded by the lines x − 2y = 0, x − 2y = −4, x + y = 4, and x + y = 1 . Find a transformation T from a region S to R such that S is a rectangular region (with sides parallel to the u- or v-axis).arrow_forward
- Algebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage
